The Ellipse at Incidence

We proceed in the same way as before. The potential, with the flow coming at an angle a from the X-axis, reads

( b2 Г

Ф = U r + cos(0 – a) – (в – a) (2.70)

r 2n

In this case, on the cylinder, the velocity components are

Г

V = 0, Ve = —2U sin (в – a) – (2.71)

2n b

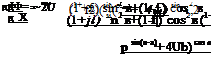The circulation corresponding to a stagnation point at в = 0 is given by Г = 4Ub sin a. On the surface of the ellipse, after some algebra, one finds

Since the ellipse has a rounded trailing edge, there is no mechanism to control the circulation in inviscid flow and an ellipse at incidence will in general produce a flow with zero circulation. For Г = 0 the two stagnation points are located at в = a and в = ж + a, see Fig. 2.18.

The pressure coefficient is given by

This is represented in Fig. 2.19 as – C + and-Cp for the upper and lower surfaces, respectively.

Fig. 2.19 Pressure coefficients for the ellipse at incidence (Г — 0)

From anti-symmetry, the lift is zero. The moment however is not. It is obtained from

where O stands for the center of the ellipse, where the moment is calculated. After lengthy algebra, the result reads

The two stagnation points are now located at в — 0 and в — n + 2a. The corresponding flow is sketched in Fig. 2.20.

The surface pressure distribution for the ellipse with a small flap is shown in Fig. 2.21.

Fig. 2.20 Flow past an ellipse with a small flap at the trailing edge

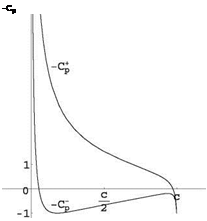Fig. 2.21 Pressure

distribution on the ellipse with small flap at the trailing edge

The lift coefficient is given by the Kutta-Joukowski lift theorem

where c = 2(b2 + a2)/b. Substituting the value of the circulation gives

b2 ґ e

Ci = 4n sin a = 2n 1 + sin a (2.78)

b2 + a2 c’

The results for the cylinder and the flat plate are obtained for e/c = 1 and e/c = 0, respectively. In small perturbation theory, the term e/c can be neglected as a second – order term when multiplied by sina ~ a.