Vorticity Transport and Irrotationality
The behavior of vorticity will be examined by formally taking the curl of the momentum equation (1.36). The manipulations will use the following identities, which are valid for any vector fields a and b.
V(a ■ b) = a ■ Vb + b ■ Va + a x (Vx b) + b x (Vx a) (1.87)
V x (a x b) = a V ■ b — b V – a + b ■ Va — a ■ Vb (1.88)
1.9.1 Helmholtz vorticity transport equation
Setting a = b = V in identity (1.87) gives
^V(V-V) = V’VV + Vxw (1.89)
ш = Vx V (1.90)
where ш is the vorticity. Using (1.89) to replace the V ■ VV term in the momentum equation (1.36) puts it into the following alternative form.
|
|
||
|
|||
We now take the curl V x [equation (1.91)], use the identity V xV() = 0, and note that the curl commutes
with the d()/dt operation. The body force field f is also assumed irrotational as is typical, so that Vxf = 0.
^-Vx(Vxu;) = – V(“) xVP + Vx ("^r) (L92)
Next we set a = V and b = ш in identity (1.88) which gives
D(1 /Р)
Dt
where the convective mass equation
![]() lDp = D(1 /p)
lDp = D(1 /p)
p Df Df
and the identity V – ш = V(VxV) = 0, have been used. Substituting (1.93) in (1.92), dividing through by p, and combining and rearranging terms finally gives the Helmholtz vorticity transport equation, with its simpler incompressible form resulting from p and p being constant.
The baroclinic source term Vp x Vp in the compressible Helmholtz equation (1.94) can cause vorticity to appear wherever there are density and pressure gradients present. However, in isentropic flow where the viscous term is negligible the isentropic p(p) relation (1.69) holds, so here the p and p gradients are parallel
Vp = yrVp = 7 – Vp (1.96)
dp p
and therefore the baroclinic term vanishes since Vp x Vp ~ Vp xVp = 0.
The term ш ■ VV on the righthand sides represents vortex tilting and vortex stretching, the latter causing a rotating fluid’s vorticity to intensify when the rotating fluid is stretched by the components of the velocity gradient matrix VV which are parallel to ш itself. However, if ш = 0 to begin with, then this term is disabled, since there is no vorticity to stretch or tilt.
The Helmholtz vorticity equation (1.94) or (1.95) simplifies greatly for most aerodynamic flows. These typically have uniform flow and hence ш = 0 upstream, and their viscous stresses are negligible outside of viscous layers and outside of shocks. In these circumstances (1.94) gives
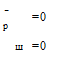
![]() (1.97)
(1.97)
(1.97) with the conclusion being that initial irrotationality persists downstream outside of the viscous layers and shock wakes. These are the same requirements as those for isentropy, discussed earlier and shown in Figure 1.11. Hence we can further conclude that flows which are irrotational are also isentropic, as illustrated in Figure 1.14.
 |
s = constant ^^ ш = 0
Irrotationality of the velocity field has great implications for flow-field representation and modeling, which will be treated in Chapter 2. It also enables the various Bernoulli relations for the pressure, considered next.











