Modern Topics

Hitherto, we have given the summary of so called classical and conventional aerodynamics. Starting from 1970s, somewhat unconventional analyses based on numerical methods and high tech experimental techniques have been introduced in the literature to study the effect of leading edge separation on the very high lifting wings or on unsteady studies for generating propulsion or power extraction. Under the title of modern topics we will be studying (i) vortex lift, (ii) different sorts of wing rock, and (iii) flapping wing aerodynamics.

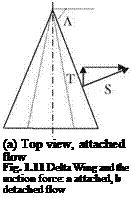
(i) Vortex lift: The additional lift generated by the sharp leading edge separation of highly swept wings at high angles of attack is called the vortex lift. This additional lift is calculated with the leading edge suction analogy and introduced by Polhamus (1971). This theory which is also validated by experiments is named Polhamus theory for the low aspect ratio delta wings. Now, let us analyze the generation of vortex lift with the aid of Fig. 1.10. According to the potential theory, the sectional lifting force was given in terms of the product of the density, free stream speed and bound circulation as in Eq. 1.1. We can resolve the lifting force into its chord wise component S and the normal component N. Here, S is the suction force generated by the leading edge portion of the upper surface of the airfoil. Accordingly, if the angle of attack is a then the suction force S = p U Г sina. Now, let us denote the effective circulation and the effective span of the delta wing, shown in Fig. 1.11, Г and h respectively. Here, we define the effective span as the length when multiplied with the average sectional lift that gives the total lifting force of the wing. This way, the total suction force of the wing becomes as

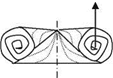
(b) perspective view, detached flow

simple as Sh. Because of wing being finite, there is an induced drag force which opposes the leading edge suction force of the wing.

Accordingly, the thrust force T in terms of the leading edge suction and the down wash w, reads T = p Г h (U sina-w;). Let us define a non dimensional coefficient Kp emerging from potential considerations in terms of the area A of the wing,

Kp = 2 Г h /(A Usina)

The total thrust coefficient can be expressed as

In Eq. 1.33, at the low angles of attack the potential contribution and at high angles of attack the vortex lift term becomes effective. For the low aspect ratio wings at angles of attack less than 10o, the total lift coefficient given by Eq. 1.11 is proportional to the angle of attack. Similarly, Eq. 1.33 also gives the lift coeffi­cient proportional with the angle of attack at low angles of attack.

For the case of low aspect ratio delta wings as shown in Fig. 1.11 if the angle of attack is further increased, the symmetry between the two vortices becomes spoiled. As a result of this asymmetry, the suction forces at the left and at right sides of the wing become unequal to create a moment with respect to the wing axes. This none zero moment in turn causes wing to rock along its axes.

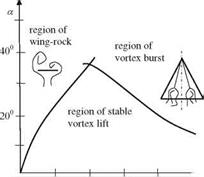
(ii) Wing-Rock: The symmetry of the leading edge vortices for the low aspect ratio wings is sustained until a critical angle of attack. The further increase of angle of attack beyond the critical value for a certain wing or further reduction of the aspect ratio causes the symmetry to be spoiled. This in turn results in an almost periodic motion with respect to wing axis and this self induced motion is called wing-rock. The wing-rock was first observed during the stability experiments of delta wings performed in wind tunnels and then was validated with numerical investigations. During 1980s the vortex lattice method was extensively used to predict the wing-rock parameters for a single degree of freedom in rolling motion only. After those years however, two more degrees of freedom, displacements in vertical and span wise directions, are added to the studies based on Euler solvers. The Navier-Stokes solvers are expected to give the effect of viscosity on the wing – rock. The basics of wing-rock however, are given with the experimental data. Accordingly, the onset of wing-rock starts for the wings whose sweep angle is more than 74o (Ericksson 1984). For the wings having less then 74o sweep angle, instead of asymmetric vortex roll up, the vortex burst occurs at the left and right sides of the wing. In Fig. 1.12, the enveloping curve for the stable region, wing – rock and the vortex burst are given as functions of the aspect ratio and the angle of attack. The leading edge vortex burst causes a sudden suction loss at one side of the wing which causes a dynamic instability called roll divergence (Ericksson

AR

1984). After the onset of roll divergence, the wing starts to turn continuously around its own axis.

Let us now give the regions for the wing-rock, vortex burst and the 2-D sep­aration in terms of the aspect ratio and the angle of attack by means of Fig. 1.12.

The information summarized in Fig. 1.12 also includes the conventional aerodynamics region for fixed wings having large aspect ratios.

The effect of roll angle and its rate on the generation of roll moment will be given in detail in later chapters.

(iii) Flapping wing theory (ornithopter aerodynamics): The flight of birds and their wing flapping to obtain propulsive and lifting forces have been of interest to many aerodynamicists as well as the natural scientists called ornithologists. After long and exhausting years of research and development only recently the proto­types of micro air vehicles are being flown for a short duration of experimental flights (Mueller and DeLaurier 2003). In this context, a simple model of a flight tested ornithopter prototype was given by its designer and producer (DeLaurier 1993).

The overall propulsive efficiency of flapping finite wing aerodynamics, which is only in vertical motion, was first given in 1940s with the theoretical work of Kucheman and von Holst as follows

1

g = TTI/AR (134)

Although their approach was based on quasi steady aerodynamics, according to Eq. 1.34 the efficiency was increasing with increase in aspect ratio. As we have stated before, the quasi steady aerodynamics is valid for the low values of the reduced frequency. This is only possible at considerably high free stream speeds. Because of speed limitations and geometry, the reduced frequency values must be greater than 0.3, which makes the unsteady aerodynamic treatment necessary. If the unsteady aerodynamics is utilized, with the leading edge suction the propulsion efficiency becomes inversely proportional with the reduced frequency. For the vertically flapping thin airfoil the efficiency value is 90% for k = 0.07 and becomes 50% as k approaches infinity (Garrick (1936)). Using the Garrick’s model for pitching and heaving-plunging airfoil, with certain phase lag between two degrees of freedom, it is possible to evaluate the lifting and the propulsive forces by means of strip theory. In addition, if we impose the span wise geometry and the elastic behavior of the wing to include the bending and torsional deflections, necessary power and the flapping moments are calculated for a sustainable flight (DeLaurier and Harris 1993). While making these calculations, the dynamic stall and the leading edge separation effects are also considered. The progress made and the challenges faced in determining the propulsive forces obtained via wing flapping, including the strong leading edge separation studies, are summarized in an extensive work of Platzer et al. (2008) Exactly opposite usage of wing flapping in a pitch-plunge mode is for the purpose of power extraction through efficient wind milling. The relevant conditions of power extraction via pitch-plunge

oscillations are discussed in a detail by Kinsey and Dumas (2008). More detailed information on proper applications of wing flapping will be given in the following chapters.