The incompressibility assumption

As a first step in calculating the stagnation pressure coefficient in compressible flow we use Eqn (1.6d) to rewrite the dynamic pressure as follows:

(2.30)

where M is Mach number.

When the ratio of the specific heats, 7, is given the value 1.4 (approximately the value for air), the stagnation pressure coefficient then becomes

po-p _ 1 fpo 1

Pa Q. lpM2 0.1MP p

Now

m = [1 + l M217/2 (Eqn (6.16a)) P 5

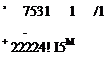Expanding this by the binomial theorem gives

Then

(?-*)

M2 M4 M6 + 4 + 40 + 1600

It can be seen that this will become unity, the incompressible value, at M = 0. This is the practical meaning of the incompressibility assumption, i. e. that any velocity changes are small compared with the speed of sound in the fluid. The result given in Eqn (2.32) is the correct one, that applies at all Mach numbers less than unity. At supersonic speeds, shock waves may be formed in which case the physics of the flow are completely altered.

Table 2.1 shows the variation of CPo with Mach number. It is seen that the error in assuming Cpt — 1 is only 2% at M — 0.3 but rises rapidly at higher Mach numbers, being slightly more than 6% at M = 0.5 and 27.6% at M = 1.0.

Table 2.1 Variation of stagnation pressure coefficient with Mach numbers less than unity

M 0 0.2 0.4 0.6 0.7 0.8 0.9 1.0

CP0 1 1.01 1.04 1.09 1.13 1.16 1.217 1.276

It is often convenient to regard the effects of compressibility as negligible if the flow speed nowhere exceeds about 100 m s-1. However, it must be remembered that this is an entirely arbitrary limit. Compressibility applies at all flow speeds and, therefore, ignoring it always introduces an error. It is thus necessary to consider, for each problem, whether the error can be tolerated or not.

In the following examples use will be made of the equation (1.6d) for the speed of sound that can also be written as

a = 4/7 RT

For air, with 7 = 1.4 and R = 287.3 J kg-1K-1 this becomes

a = 20.054/7 ms"1

where T is the temperature in K.

Example 2.1 The air-speed indicator fitted to a particular aeroplane has no instrument errors and is calibrated assuming incompressible flow in standard conditions. While flying at sea level in the ISA the indicated air speed is 950 km h-1. What is the true air speed?

950 kmh-1 = 264ms-1 and this is the speed corresponding to the pressure difference applied to the instrument based on the stated calibration. This pressure difference can therefore be calculated by

Po-p = Ap = – po^i

and therefore

p0-p = – x 1.226(264)2 = 42670Nm-2

Now

In standard conditions p = 101 325Nm 2. Therefore

po _ 42670 p ~ 101 325

Therefore

1+ім2 = (1.421)2/7 = 1.106

^M2 = 0.106

M2 = 0.530 M = 0.728

The speed of sound at standard conditions is

a = 20.05(288)* = 340.3 m s-1

Therefore, true air speed = Ma = 0.728 x 340.3

248ms-1 = 891 km IT1

In this example, a = 1 and therefore there is no effect due to density, i. e. the difference is due entirely to compressibility. Thus it is seen that neglecting compressibility in the calibration has led the air-speed indicator to overestimate the true air speed by 59 km IT1.