Limitations of full potential solutions

One limitation of the FP equation is that it applies only to inviscid flows. This can be mostly remedied by using the Wall-Transpiration boundary layer model described in Chapter 3. For example, in the 2D case the flow-tangency BC (8.24) would be modified to

d m

pV Ф-П = — (8.28)

ds

where m = peue5* is the viscous mass defect. This is governed by a suitable form of the boundary layer equations which would need to be solved together with the FP equation.

Another limitation of the FP equation is that its resulting velocity field V = УФ is irrotational, and hence its solution will not have a shock wake like the one shown in Figure 8.8, even if the solution has a shock present on the airfoil. The wave drag as calculated by far-field integral (8.19) over the shock wake will then incor­rectly be zero. The correct calculation of wave drag therefore appears to require the direct pressure force integration over the airfoil surface via (5.5). However, this was shown to be very sensitive to cancellation errors in Section 5.1.2, so the following more accurate approach is needed.

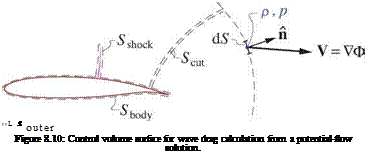
Consider the control volume surface shown in Figure 8.10, consisting of the four pieces Souter, Scut, St>ody, Sshock. The integral momentum theorem in the freestream X direction summed over all four pieces can be assumed to be zero, since the overall contour is topologically empty and contains only smooth potential flow which satisfies the momentum equation.

[ pn + p(V ■ n)V ] ■ X dS = 0 or [ ] dS + [ ] dS + [ ] dS + [ ] dS = 0

outer cut body shock

In addition, in 2D flow the Souter piece can also assumed to be zero since the outer flow is potential and has no momentum defect, and the Scut piece is also zero since its two П vectors are opposite. Hence the two remaining pieces must be equal and opposite, so we have

DWave = [ ] dS

body

= – [ pn + p(V■ n)V ] ■ X dS (8.29)

shock

and therefore the wave drag can be computed by evaluating integral (8.29) only on the contour surrounding the shock, noting that n points into this contour. This avoids the cancellation errors which would occur with evaluating the Sbody integral directly.

Similar arguments can be made for wave drag in 3D potential flows. In this case the Souter integral is only the induced drag Di, which can be evaluated by Trefftz-plane integration over the wake cut as discussed previously. The Sbody integral is then Di + Dwave, so that the integral over the shock-enclosing contour isolates the wave drag component.

Dwave = – S [ pn + p(V■ n)V ] ■ X dS (8.30)

shock