Theoretical and applied aerodynamics
The purpose of this chapter is to provide the basic aerodynamics necessary to an understanding of the material developed in the following chapters. To begin, three fundamental relationships of fluid mechanics are considered: the equation for conservation of mass and the momentum and energy theorems.
Conservation of Mass
 |
Consider a fixed control surface S shown in Fig. 2-1 through which fluid is passing. The rate at which mass accumulates within the volume contained
within S is equal to the rate at which mass flows into the volume minus the rate at which it flows out. To put it another way, the net mass rate of flow out of S must equal the negative of the rate at which mass accumulates within the volume. Hence, if n is the unit normal directed outward from S, then
V is the volume enclosed by S and dx is a differential element of volume. If we use Gauss’s divergence theorem and the fact that V is fixed, we obtain
dx = 0.
Because the volume V is arbitrary, the integrand must equal zero; hence the differential equation which the mass density and velocity vector must obey in order to conserve mass at every point in a flow becomes
![]() V-(p V) + ^ = o.
V-(p V) + ^ = o.
For steady incompressible flow this result leads to the condition that the divergence of the velocity vector must equal zero
V-V = 0. (2-2)
In rectangular coordinates this is expressed as
du 8v dw dx + dy + 8z
u, v, and w are the components of the velocity vector in the x-, y-, and z-directions, respectively.
Momentum Theorem
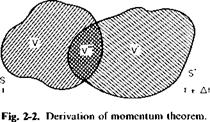 |
Consider the aggregate of fluid particles contained within a control surface S at time t, as shown in Fig. 2-2. At time / + At these particles have
|
J p (t + At) dx — J* p V(t) Jtj; |
moved to a new position and are now enclosed by the surface S’; V is the volume enclosed by S, V, by S’, and V" is the volume common to both V and V. The rate of change of momentum of the fluid particles under consideration is obviously
|
im0 h {J*+ Лг) ~ V(^dx V" |
The first term in the first limit represents the momentum that has passed out of the volume V in time At; the second term represents the amount that has entered V during the interval Аг. Hence the difference between the two in the limit, as Аг -*■ 0, is the time rate at which momentum passes out of the surface S, sometimes stated as the net flux of momentum out of the surface. If n is the unit normal directed positively out from the surface S, the first limit can be written
|| pV(-n)dS.
s
The second limit represents the rate of change of momentum of the fluid particles contained in the volume V (or V" in the limit). In the limit, as Аг -* 0, this term becomes (5/51) JJJ pV dx.
v
Thus, if F is the total vector sum of all the forces acting on the fluid particles in V, it follows from Newton’s second law of motion that
Of most concern to the material in this book is the problem of the steady flow of an inviscid fluid—in particular, air. Thus for the particular case in which gravity forces, viscous shearing forces, and unsteady effects can be neglected Eq. (2-4) takes the form
-|| pn dS + В = || pV(V-n) dS. (2-5)
s s
In Eq. (2-5) p is the normal pressure acting on the control surface and В represents any body forces that are present within the control surface.
An equation can also be formulated for the angular momentum in a manner similar to that used for the linear momentum. The result, similar to (2-2), states
Here Q is the vector sum of any torques acting on the fluid particles within the control surface.
The results expressed by Eqs. (2-4) and (2-6) can be stated simply: The sum of external forces (or moments) acting on a control surface and internal forces (or moments) acting on the fluid within the control surface produces a change in the flux of (angular) momentum through the surface and an instantaneous rate of change of (angular) momentum of the fluid particles within the control surface.











