Drag

The drag is given by Viviand as

u v w

d£, — 2 Hy +—- nz d^R

JsRUU y U V R

— ArefCDi + ArefCDw (6.20)

where n — {0; ny; nz} is the normal unit vector to SR. The two terms are interpreted, the former as the induced drag due to the vortex sheet, the latter as the wave drag due to shock waves in supersonic flow. The induced drag refers to the drag induced by lift or vortex drag. If the circulation is zero, there will be no vortex sheet and the first term will vanish.

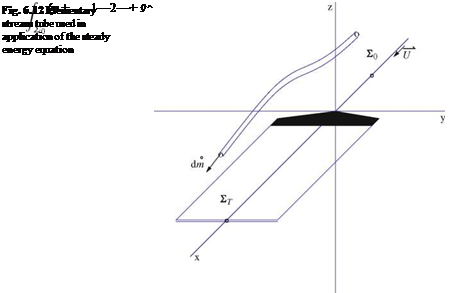It is also possible to derive the formula for induced drag from the steady energy equation for small disturbance flow. Consider the small stream tube entering through the plane S0 and exiting at the Trefftz plane, Fig. 6.12. The steady energy equation applied to all such small stream tubes yields

The internal energy term u = Cv T, and the pressure/density term cancel out as the flow returns to its free stream temperature, pressure and density. The gravity term is neglected. Ws represents the shaft work done to the fluid. Upon simplification, this reads

One can see that the kinetic energy leaving the Trefftz plane is larger than the kinetic energy entering through the S0 plane. Work has been done to the fluid. The work is due to the thrust that is needed to balance the induced drag to maintain the steady motion of the wing in the frame where the fluid is at rest in front of the wing. The induced drag is present, even in inviscid flow. It can be written

1 3

Ws = – T. U = D. U = 2 P^U 3ArefCot (6.23)

Upon substitution of the definition of the induced drag coefficient, one finds

The surface integral can be transformed into a contour integral along the trace of the vortex sheet. Consider the identities

The induced drag now reads

The last integral vanishes since, in the Trefftz plane, the flow is two-dimensional with u = 0 hence д2ф/дх2 = 0 and the governing equation reduces to the Laplace equation in the (y, z)-plane. The divergence theorem is applied to the first integral where the vortex sheet is excluded in order to have a simply connected domain with a

Fig. 6.13

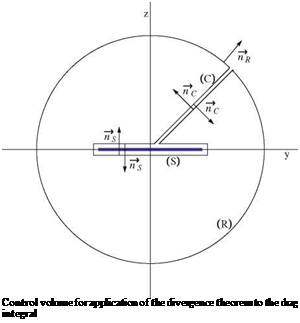contour made of a large circle (R) of radius R in the far field, a contour (S) surrounding the sheet and a cut (C) to connect them, as shown in Fig. 6.13.

The integral now reads

The contribution on (R) goes to zero as R since ф and Vф vanish far away from the vortex sheet. The integral on (C) is zero from continuity of the integrand and change of sign of the normal vectors. There remains

where we have accounted for ny = 0, n+ = — 1and nz = 1, and replaced the contour (S) by the segment [— |, |]. We have seen that the w-component is continuous across the vortex sheet, and the jump of the potential is the circulation, i. e. Г(y) = ф+ — ф— =< ф >. The result follows

where wT stands for the vertical component of the induced velocity in the Trefftz plane. It will be seen that the vortex sheet trailing a thin wing with positive lift induces a negative component w above and below the sheet, called the downwash. The downwash is responsible for the induced drag. According to the 2-D result of thin airfoil theory in incompressible flow, the contribution to the downwash at a point y of the vortex sheet due to an infinite vortex line of intensity dГ = Г’dn located at n is given by

1 Г’

2n y – n

and the resulting wT reads

In incompressible flow, one interpretation of the induced drag is obtained by revisiting the Kutta-Joukowski lift theorem applied to a section of the wing, but accounting now for the downwash. Lets assume that there is a downwash ww (y) induced by the vortex sheet in the neighborhood of the wing. The bound vortex sees an incoming flow that has been deflected down, as shown in Fig. 6.14.

The angle between the undisturbed velocity vector U and the velocity vector q seen by the profile is called induced incidence. The induced incidence a. t (y) = tan-1 (ww(y)/U) ~ ww(y)/U is negative, in general, for positive lift. The lift contribution is unchanged by the induced incidence since cos a. i ~ 1. The induced element of drag is given by

dDt = – dF sin at ~ – p^Ur(y)atdy = – p^r(y)ww(y)dy (6.32)

The induced drag coefficient therefore becomes

This result is consistent with that derived earlier, because at the wing, application of the Biot-Savart formula in incompressible flow shows that the induced velocity is

half that in the Trefftz plane, since the contribution of a semi-infinite line vortex is half that of an infinite one, i. e.

The formula for the induced drag can be manipulated upon substituting the down – wash as

b b,

1 Г2 [2 r'(n)

f = 2nW_b/bГ( y^n

Using integration by parts for the integral in the bracket, yields

^ Г'(n) [Г(y) ln |y – n|]-b – f b_ Г'(y) ln Iy – nldy I dn

The integrated term in the bracket is zero since Г (±— 0, thus we are left with a symmetrical expression for the induced drag that reads

Interestingly, in slender body theory, a similar formula can be derived for the wave drag. Indeed, away from the body, the disturbance potential behaves as if the body is a body of revolution and the leading term in the expansion of the potential solution corresponds to a distribution of sources along the axis that depend on S'(x), where S(x) represents the cross-section area of the body of length l. If furthermore the body satisfies S'(0) — S'(l) — 0, that is if the body is finite or connects to a cylindrical part, the wave drag reads

ArefCDw –1 S"(x)S"(0 ln |x – eidxde (6.38)

2n 0 0

This analogy can be used to find the body of revolution with minimum wave drag in supersonic flow from the finite wing with minimum induced drag in incompressible flow.