Streamline and Stream Tube

These terms are defined in Section 2.3. For steady flows—the subject of this chapter—a streamline is the path that a fluid particle traces as it traverses the flow field. Another way to express this is that streamlines are lines in the flow that are everywhere tangent to the local velocity vector. Notice that because there can be no flow across a streamline, a solid wall can be interpreted as a limiting streamline. From this, it can be seen that any flow streamline can be “cross-hatched” and thought of as a solid wall if such an interpretation proves useful. In a two-dimensional flow, the tangency property of a streamline requires that

where (x, y) are the coordinate directions and (u, v) are the respective velocity com­ponents. Notice that Eq. 4.1 is equally valid for a compressible flow. A single stream­line indicates velocity direction, not magnitude. However, because there is no flow across streamlines, the relative spacing between two nearby streamlines at several points in a flow field in an incompressible flow is a measure of the relative flow – velocity magnitudes at these points because the mass flux (pV) is constant between streamlines and the density is constant as well. A stream tube in a two-dimensional flow is defined by the distance between two adjacent streamlines and a dimension that is an arbitrary length perpendicular to the plane of the flow and usually set to unity.

EXAMPLE 4.1 Given: A steady, incompressible, two-dimensional flow has a velo­city field with velocity components given by u = y, v = x2.

Required: Find the equation of the streamline passing through the point (3,2).

Approach: Appeal to the definition of a streamline.

Solution: Using Eq. 4.1, the slope of the streamline is given by:

— = ——> ydy = x2dx. Integrating, -— — = constant. dx y 2 3

Therefore, the equation of the stramline passing through point (3,2) is given by:

Appraisal: As a preliminary, the differential form of the continuity equation (Eq. 3.13) could have been used to test whether the “given” is a physically poss­ible flow field. (It is, and the student should confirm this.)

EXAMPLE 4.2 Given: A two-dimensional, incompressible flow is described by the magnitude of the local velocity vector and the equation of the streamlines—namely:

IVI = (y2 + лс4)1/2, y— x— = constant.

Required: Find the velocity components, u and v.

Approach: Appeal to the definition of a vector in terms of its components and then use the streamline definition, Eq. 4.1.

Solution: |V| = Vu2 + v2 = u, 1 + —- = ^y2 + x4. Next, taking the derivative of

V u2

the given equation of the streamlines, ydy – x2dx = 0. From this, it follows that:

= — = — (see the following appraisal). dx y u

Substituting the quantity x2/y for v/u in the square root expression and squaring

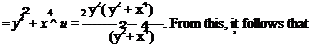both sides: u21 1 + x­I y

u = ± y. Finally,

v v x 2

— = — = — ^ v = ± x2. u ±y y

Hence, the required velocity components are found.

Appraisal: There is a plus/minus in the answer because the flow direction is not specified. Thus, the flow could be either up and to the right or down and to the left. Note also that although v/u = x2/y, we cannot state that v = x2 and u = y (because the v/u expression corresponds to one equation in two unknowns). To see that taking v and u as simply the numerator and denominator of the quotient is not correct, rework this problem with the same equation for the streamlines

but with the magnitude of the velocity vector given by 2yJ y2 + x4.