Lifting Surface Theory for Slender Wings of Low Aspect Ratio

Lifting line theory is applicable only to wings of large aspect ratio of approximately four or more. For wings of lower aspect ratio we should resort to a lifting surface theory, which involves finding a potential flow to satisfy the Kutta condition all along the span but at the same time satisfying the boundary condition that the normal velocity components vanish every­where on the wing surface. The exact formulation and solution of the general lifting surface problem can be found in several references. Unfortunately, these formulations are rather unwieldy unless they can be programmed on a digital computer. Even then the task can be a formidable one.

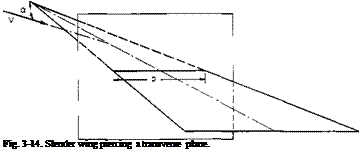Instead of an exact lifting theory, some approximate developments can be quite useful. First, consider the limiting case of the slender pointed wing

shown in Fig. 3-14. Slender wing theories, developed in Refs. 7 and 8, assume that the flow is essentially two-dimensional in any transverse plane.

For this development it is convenient to write the equations of motion (2-11) by using a repeated subscript notation.

The repeated subscript j indicates that this term is to be summed over the indicated range of j. In terms of the velocity potential, the above equation becomes

Multiplying the above by dxt and integrating, we obtain

-г – + t(u2 + v2 + w2) + – = constant. (3-48)

ct 2 p

Now consider two points on the wing, one on the lower surface and the other on the upper surface directly above the first. In view of the assumption of two-dimensional flow in a transverse plane, и and w at the two points are equal, whereas v and the velocity potential ф on the lower surface are the negative of their values on the upper surface. Hence, if Ap is the pressure difference from the upper to lower surface, it follows from (3-48) that

(3-49)

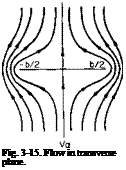
дф/dt is evaluated by calculating the flow in the transverse plane of Fig. 3-13. This flow is illustrated in Fig. 3-15. As the wing penetrates this plane, the dimension b varies, thereby causing a change in ф. Hence (3-49) can be expressed as

. „ дф dx

Ap = 2p^- — dx dt

db дф

pV dx db

The problem is that of finding the velocity potential for two-dimensional flow past a flat plate normal to the flow with a free-stream velocity of Fa.

This problem can be easily solved by the methods of conformal mapping. However, the solution is beyond the scope of this book, and instead we shall obtain it by recalling that the downwash produced by a vortex sheet trailing from an elliptic Г-distribution is a constant. Thus, if an equal and opposite velocity is added to this flow field, the velocity along the vortex sheet will be zero; but this is identical to the boundary condition along the flat plate. Hence, if the Г-distribution is

the constant downwash induced in the ultimate wake is

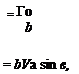w

But this is to equal Fa; thus

Г

where

У

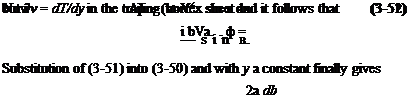
"Г’*

From (3-52) it can be observed that Ap varies linearly with the longitudinal rate of change of b. Note also that Ap is constant on rays emanating from

the apex which pass through constant values of y/(b/2). It can also be seen that Ap is infinite at the edges of the sheet. It is argued in Ref. 9 that (3-52) holds only up to the maximum value of b; fimax, and that beyond that, if b diminishes, the surface contributes nothing to the wing characteristics.

If (3-52) is integrated over the wing surface, it will be found that the span – wise loading is elliptical so that

^ AR a, 2	(3-53)
C2.
я AR	(3-54)

From (3-52) we can also determine the center of pressure for the slender wing. If this is done, we shall find that the center of pressure shifts forward for low aspect ratios. Unlike CL and CDi, the center of pressure depends on the planform shape.

The results, of course are strictly applicable only for the limiting case of zero aspect ratio. However, the result that the spanwise loading is elliptical suggests an approximate model that may hold throughout a wide range of aspect ratios.