STRUCTURE OF UNSTEADY VORTICAL WAKES BEHIND BLADES OF MUTUAL-MOVING ROWS OF AN AXIAL TURBOMACHINE

V. E. Saren, S. A. Smirnov

The Central Institute of Aviation Motors Aviamotornaya st. 2, Moscow, 111116, Russia *

Abstract At research of effects of mutual circumferential shift of stators in system of rows stator-rotor-stator of an axial turbomachine (clocking effects, [1-3]) signif­icant periodic velocity pulsations were found out in the field of turbulent vortical wakes behind rotor blades [3]. The basic frequency of these pulsations is equal to stators vanes passing frequency past rotor blade, and the amplitude of veloc­ity pulsations essentially depends from stators clocking position. According to the Thomson theorem it is necessary to expect that the specified pulsations are caused by the free vortexes, descending from rotor blades owing to change on them of fbw velocity circulation at interaction of rows. Clearly, that presence in vortical wakes of progressing waves of vorticity causes acoustic disturbances and additional aerodynamical losses owing to energy dissipation. As dissipa­tive function depends on distribution of vorticity, for an estimation of the losses, caused a rotor-stator interaction, the description of vortical wakes structure at presence in them of free vortexes is necessary.

This paper contains results of free vorticity measurements in relative flow be­hind a rotor at its interaction with next stators. For the description of experimen­tal results the semiempirical theory, based on model of linear isotropic turbulent diffusion at presence of a velocity gradient, caused by stationary (time-averaged) vortical wakes, is offered.

Keywords: Unsteady vortical wakes, mutual-moving rows

1. Experimental results

Measurements were carried out on large-dimensional (the external diame­ter 1,2m) low-speed (frequency of rotation 2000rev/min) the axial compressor,

*Work is executed at financial support of International scientific and technical centre (ISTC), the grant number 672.2.

189

K. C. Hall et al. (eds.),

Unsteady Aerodynamics, Aeroacoustics and Aeroelasticity of Turbomachines, 189-201. © 2006 Springer. Printed in the Netherlands.

containing system of rows stator (IGV) – a rotor (R) – stator (S), and in de­tail described in [4]. Numbers of stators vanes are identical, and axial gaps are determined so that clocking effect were significant. The velocity field was measured two-component laser anemometer on various radiuses in various ax­ial sections between R and S.

On Fig.1 cascades of airfoils on mean radius and the line LL along which measurements were carried out are shown. At the fixed point of measurement registration of two velocity components (axial and tangential) is carried out on the PC in 129 moments of time in regular intervals allocated on the R blade passing period T = hr /u, where hr and u, accordingly, are a pitch and linear speed of R blade. During data recording the information on velocity compo­nents for each of 129 moments of time are collected. Common time of regis­tration and sampling of representative data were determined by total number of the data (3000) for everyone velocity component.

Let W = W(r, x,y, t) is a velocity vector, determined in the R system of reference, where r, x, y is, accordingly, radial, axial and tangential (along front) coordinates, and t is time. To investigate change W along front, it is necessary to execute measurements in points with tangential coordinates yn(n = 0,1, 2,… ,N),yN = yo + hr. In experiments measurements were carried out in the S system of reference (r, x, yо, to). Thus coordinate yo is fixed, and velocity W is described as a variable on time 10 with period T and conformity of coordinates is

Thus, the stated technique allows to receive a relative fbw velocity in the R system of reference according to measurements in the S system (i. e. in a motionless point) at sufficient number N of measurement points on a S pitch hs in M = 129 points of measurements on period T.

Let the projection of instant relative velocity behind R W = W(r, x, y, t) on averaged on period T0 and a pitch hr velocity W00 is presented as

(IF • Woo) , , , , „

,TT7 ,2 = Wo{r, x, y) + w{r, X, y, t),

| W001

where W00 is a zero member of expansion (3), and w0 is averaged on period T0 the velocity, referred to |W00|. Then distribution w0 = w0(r, x, y) along a line LL on a pitch hr describes time-averaged flow behind a R in a core of a flow and in the field of a vortical wake.

The value w0 is presented on Fig.2 for section LL, distant from the R trailing front on distance of A = 8, 75mm on mean radius of a flawing path. The full axial gap between R and S made thus A23 = 20,5mm. Coefficients of expansion 3) were calculated according to the measurements executed for N = 10 and M = 129. It allows to determine 20 harmonics on coordinate y and no more than 2 harmonics on time t. It is hereinafter supposed that coordinates x and y are referred to a R pitch hr, and time to period T0.

For comparison on Fig.2 values w0 are put, received under the theory of an automodelling vortical wake [5]. Apparently, experimental data well are coor­dinated to the theoretical values, received for value of profile losses Z = 0,051. Design velocity |W001 = 91,5m/s at exit fbw angle в = 42° (see Fig.1). The appropriate values, received in experiment, are equal: | W001 =91,1m/s; в = 41,9°. Thus vector W00 does not depend from clocking position of IGV and S [8].

The value w = w(r, x,y, t) describes distribution along a line LL of the pulsation part of ft>w velocity, referred to | W 001. Examples of w distributions

are presented on Fig.3, where the data are received for two values of parameter v = n/N (n = 0,1,…,N), equal referred to hs shift of IGV be relative S in a positive direction of axis Oy (against R rotation). The chosen values v = 0,2 and 0,6 correspond to clocking positions of stators, providing the greatest and least levels of velocity pulsations behind a R. The moments of time t = 0,5 and t = 0,8 correspond to a situation, when velocity pulsations are in an antiphase.

Apparently from the received distributions w, periodic velocity pulsations are rather small in a fbw core and rather significant in a zone of a vortical wake and essentially depend on interaction of rows. It testifies to existence in the field of vortical wakes behind R blades significant flow vorticity, which periodically varied in time and in space. Specified vorticity may be approxi­mately determined, if the expansion (3) is received for sections LL, distant on various distances x from R trailing front. In this case vorticity may be pre­sented as

n = Пкі^ ФіМІ+к^)у ■ e~i27Tkt (4)

l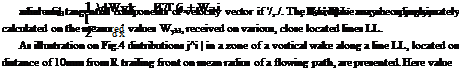
к

20

fit (r, X,y) = elMl+h^)y

l=—20

corresponds to 1-st harmonic on time of the expansion (4), and values dWykl/dx are received as a half-sum of the left and right relations of finite differences according to measurements on lines LL1 and LL2, located, accordingly, on distances A = 9mm and A = 11mm from R leading front. All values |fi 1| on Fig.4 are referred to |fi110, received on an axis of a vortical wake y = y0. The data are presented for various values of parameter v = 0, 2; 0,4; 0,6.

Prominent feature of the received distribution is its nonmonotonic change at removal from an axis of a vortical wake that in a fbw core values of | 11, as

a rule, do not exceed0,1 |fi1|0. Results of experiment allow to conclude that periodic vortexes behind a R, drifted by a flow, intensively diffuse in a zone of vortical wakes behind blades. However, as against classical diffusion with

monotonous decrease (as an exponent) at removal from a source, diffusion of free vortexes has nonmonotonic character of distribution across a layer.