Coupling Integral Formulations of both Inviscid and Viscous Flow Regions

9.1.1.1 Analysis of Internal Flows in Variable Area Ducts

The governing equations for the quasi-one dimensional flows in the inviscid core are

given by the conservation laws, assuming perfect gas:

continuity

energy

dpEA dpuHA

ПГ + JLaXT =0 (9.9)

where H = E + р/р = yp/(y — 1)p + u2/2.

H = CpT is the total enthalpy, E = CvT the total internal energy, T the total temperature and A(x) denotes the effective cross-sectional area. The average inviscid velocity in the slowly varying area duct, u, denotes also the velocity ui at the edge of the boundary layer.

The above equations can be solved with artificial viscosity or using upwind schemes. For the latter, see Chattot [2].

For the steady case, the time dependent terms vanish and the continuity and energy equations reduce to the integrals of motion in terms of the algebraic relations:

pu A = m = const. (9.10)

2

Y P u

+ = H = const. (9.11)

Y — 1 P 2

The momentum equation can be rewritten in one variable, say u (or p) by elim­inating the other two variables using the two algebraic relations. Again, artificial viscosity or upwind scheme can be used for this nonlinear scalar ordinary differen­tial equation, see Hafez et al. [3].

On the other hand, the von Karman integral momentum equation for the steady compressible boundary layer is given by:

where

n = 0 for planar flow n = 1 for axisymmetric flow

In the above equation, S* is the displacement thickness, and в is the momentum thickness, while Cf is the skin friction coefficient.

A special case is the incompressible flow through a channel of width b, where p is constant and the effective area is simply A(x) = Ageo(x) — 2bS*(x).

where Ageo is the geometric area. Moreover, uA = mIp = C = const., hence

The problem thus reduces to solving the integral momentum equation. There are however three variables in this equation: 6*, в and Cf. Following Pohlhausen’s approximate method, a form of the velocity profile in the boundary layer is assumed, where

u y

= f (n) and n = (9.14)

ui 6(x)

where 6(x) is the total thickness of the boundary layer and y is the distance from the duct wall. The function f should satisfy some boundary conditions on u:

d2u	ui	dui	d 3ui
at y = 0, u = 0, dy2	v	dx	dx3	= 0,…	(9.15)
	du		d 2u
as y ^ ж, u ^ ui,	dy	0,	dy2	0	(9.16)

The conditions at infinity are only approached asymptotically. These conditions will be applied at y = 6 instead.

The conditions on f (n) become:

f (0) = 0, f" (0) = —Л, f(0) = 0,… f (1) = 1, f ‘(1) = f "(1) = f ‘"(1) = 0

where

62 dui v dx

From the assumed velocity profile, 6*, в and Cf are related to 6 as follows:

* = 6 / V — f )dn 0	(9.20)
= 6 ( f — f2)dn 0	(9.21)
2v Cf = f ‘(0) ui 6	(9.22)

Pohlhausen used a fourth order polynomial for f (n), satisfying five conditions, two at y = 0 and three at y = S. Hence

U 3 4 1 3

– = f (n) = 2n – 2n3 + n + 7Лп(1 – n) (9.23)

Ui 6

With this form, the following relations can be obtained:

S* 3 Л в 1 / 37 Л Л3 Cf ui S Л

— =————— , – = , = 4 + – (9.24)

S 10 120 S 63 5 15 144 2^ 3

Thus, a nonlinear ordinary differential equation in Л must be solved numerically. A step by step technique can be used in a standard way.

Obviously, other forms of the velocity profile can be chosen. Variations of Pohlhausen method are available in the literature. Notably is Thwaites’ method which is based on an empirical relation to simplify the algebra.

Methods based on two equations, integral momentum equation and integral mechanical energy equation, are known to produce more accurate results. In particu­lar, a family of profiles with two parameters are introduced in the work of Wieghardt [4], see also Walz [5], Truckenbrodt [6] and Tani [7].

In their work, the mechanical energy equation is derived by multiplying the momentum equation by U and upon integration across the boundary layer, an integral relation is obtained in terms of a mechanical energy thickness.

The final results for incompressible flow is

where

(9.26)

Alternatively, Listsianski and Dorodnitsyn used their method of integral relations, where the boundary layer is divided into strips and approximates are obtained via weighted averaging over each strip. For more details see Holt [8].

In this regard, one should mention that over the last century, boundary layer equations have been solved by the methods of weighted residuals, including the method of collocation, the method of moments and the Galerkin method. See for example Schlichting [9], Rosenhead [10].

For compressible flows, an integral based on the total energy equation is used, see Ref. [11].

Following Stewartson [11], a total temperature is defined as:

u2

T = t + (9.27)

2Cp

where t represents the static temperature. In terms of the total temperature, the energy equation reads:

d T d T + "" sy	d fi d dy _ Pr dy
(9.28)
T +

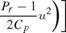
Upon integration w. r.t. y, one obtains

d pu (T – Ti) dy dx 0	i d T Pr dy ly=0
(9.29)

where

ui

T, = ti + b (9.30)

2Cp

Pr is the Prandtl number. If the wall is insulated (i. e. dT/dy = 0 at y = 0), the equation is integrated to give (see [12])

pu (T – Ti) dy = const. (9.31)

For the special case, Pr = 1, Crocco and Busemann noticed independently, that T = const. The total temperature is thus constant everywhere across the boundary layer and the wall temperature is given by:

(9.32)

In general, the total energy thickness is defined as:

(9.33)

The integral mechanical energy equation becomes:

d C qw

dx pi ui Ti

where qw is the heat flux through the wall

qw = k |y=0 (9.35)

dy

Gruschwitz [13] introduced a method similar to that of Pohlhausen to calculate compressible flows with arbitrary Prandtl numbers, using both the momentum and total energy integral equations and in this case a temperature profile is assumed satisfying the temperature boundary conditions.

On the other hand, Oswatitsch [14] developed a simpler method assuming Pr = 1. Since the pressure is constant across the boundary layer, one can show that, see [15]

Hence, only the momentum integral equation for compressible flow with a quartic polynomial for the velocity in terms of Л, may be used, where the density and the temperature distributions are related to the velocity profile.

The above development is for steady laminar flows. For steady and unsteady compressible turbulent flows, the reader is referred to [16], where several empirical formulae are employed.