The Prandtl Boundary-Layer Equations

The derivation of the Prandtl boundary-layer equations for a two-dimensional, incompressible flow is carried out in detail in this section because it is the basis for many practical aerodynamics applications. The resulting equations describe a laminar boundary layer. These equations are modified in later sections to represent turbulent boundary layers.

The shape of the velocity distribution in a boundary layer is deduced from physical reasoning in Chapter 2, as shown in Fig. 2.3, which is repeated here. In Fig. 8.9, the boundary-layer thickness is given the symbol 5 and represents the dis­tance from the wall at which the velocity in the boundary layer approaches that of the flow outside the boundary layer. It must be emphasized that this symbol, as used throughout this chapter, always denotes a length in physical units (e. g., inches or centimeters). A more precise definition of this thickness is established after the solu­tion for the velocity profile is obtained in Section 8.6 and interpreted in Section 8.7.

Two assumptions are made in deriving the boundary-layer equations, as follows.

1. The boundary-layer thickness, 5, is very small compared to a streamwise distance, x, along a body; thus, 5/x << 1.0.

2. The Re number is very large; Re >> 1.

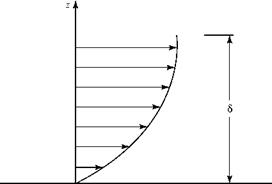Figure 8.9. Velocity distribution in a boundary layer.

The assumption that a typical boundary layer is very thin is confirmed by experi­mental data. For example, at a distance of 1 foot back from the leading edge of a thin airfoil at zero angle of attack in a 50-ft/s freestream at standard sea-level conditions, the boundary-layer thickness is 5 = 0.009 ft (0.11 in). The value of the Re number for this example (based on a characteristic length of 1 foot) is Re = 322,500. Of course, near the leading edge, where x approaches zero, the assumption 5/x << 1.0 fails. How­ever, this occurs only over a length that is a minute fraction of an airfoil chord length and therefore is of little concern from a practical point of view.