General Slender Body

For a general slender body we assume that the body surface may be defined by an expression of the following form

B(x, y, z) = B(x, у/є, z/e) = 0, (6-41)

where e is the slenderness parameter (for example, the aspect ratio in the case of a slender wing and the thickness ratio in the case of a slender body of revolution). With the definition (6—41), a class of affine bodies with a given cross-sectional shape is studied for varying slenderness ratio e, and the purpose is to develop the solution for the flow in an asymptotic series in € with the lowest-order term constituting the slender-body approxima­tion. In the stretched coordinate system

V = 2//e, 2 = z/« (6-42)

the cross-sectional shape for a given x becomes independent of e. From the results of Section 6-4 it is plausible that the inner solution would be of the form

Ф* = Ux[x + Є2Ф2ІХ, У, z) + ■ • • ], (6-43)

that is, there will be no first-order term. The correctness of (6-43) will become evident later from the self-consistency of the final result. For a steady motion the condition of tangential flow at the body surface requires that the outward normal to the surface be perpendicular to the flow velocity vector:

Or, upon introducing (6-42) and (6-43) and dropping higher-order terms,

Bx + B&iy + ВІФІі = 0. (6-45)

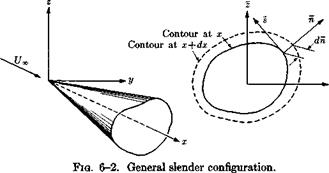
This relation may be put into a physically more meaningful form in the following manner. Introduce, temporarily, for each point on the contour considered, a coordinate system n, s such that n is in the direction normal to, and 5 tangential to, the contour at the point, as shown in Fig. 6-2. Obviously, (6-45) then takes the form

Bx + ВкФІк = 0. (6-46)

Let dn denote the change, in the direction of n, of the location of the contour when going from the cross section at ж to the one at x + dx. Moving along the body surface with dS = 0 we then have

dB = Bx dx + Bn dn = 0. (6-47)

Upon combining (6-46) and (6-47) we obtain

*;, = g. (e-48)

a condition that simply states that the streamline slope must equal the surface slope in the plane normal to the surface.

By introducing (6-43) into the differential equation (1-74) for Ф we find that Ф2 must satisfy the Laplace equation in the 5, г-plane:

^2vv + Фггг = 0. (6-49)

A formal solution may be obtained by applying (2-124). (This solution was deduced by using Green’s theorem in two dimensions.) Thus

where index 1 denotes dummy integration variables as usual, and

As in the body-of-revolution case the function $2(x) must be obtained by matching. Note that (6-50) is in general not useful for evaluating Ф2, since only the first term in the integrand is known from the boundary condition on the body. Nevertheless, it can be used to determine Ф2 for large F, since then d/dnlnr1 may be neglected compared to In Fx and, furthermore, fi may be approximated by F. Hence the outer limit of the inner solution becomes

(6-53)

which is the same as the solution (6-11) for an axisymmetric body having the same cross-sectional area distribution as the actual slender body. We shall, following Oswatitsch and Keune (1955), term this body the equivalent body of revolution. By matching it will then follow that <?2(я) must be identical to that for the equivalent body of revolution. We have by this proved the following equivalence rule, which was first explicitly stated by Oswatitsch and Keune (1955) for transonic flow, but which was also implicit in an earlier paper by Ward (1949) on supersonic flow:

(a) Far away from a general slender body the flow becomes axisymmetric and equal to the flow around the equivalent body of revolution.

(b) Near the slender body, the flow differs from that around the equivalent body of revolution by a two-dimensional constant-density crossflow part that makes the tangency condition at the body surface satisfied.

Proofs similar to the one given here have been given by Harder and Klunker (1957) and by Guderley (1957). The equivalence rule allows great simplifications in the problem of calculating the perturbation velocity potential

First, the outer axisymmetric flow is immediately given by the results of the previous section. Secondly, the inner problem is reduced to one of two-dimensional constant-density flow for which the methods of Chapter 2 may be applied. The following composite solution valid for the whole flow field has been suggested by Oswatitsch and Keune [cf. statement (b) above]: Let <pe denote the solution for the equivalent body of revolution and <P2 the inner two-dimensional crossflow solution that in the outer limit becomes <p2 ~ (1/27Г)S'(x) In r. Then the composite solution

<pc = <Pe + <p2 — lnr (6-55)

holds in the whole flow field (to within the slender-body approximation).

As in the case of a body of revolution, quadratic terms in the crossflow velocity components must be retained in the expression for the pressure, so that (cf. Eq. 6-23)

Cp = — 2<(>x — ip% — ip. (6-56)

In view of the fact that the derivation given above did not require any specification of the range of the free-stream speed, as an examination of the expansion procedure for the inner flow will reveal, it should also be valid for transonic flow. As will be discussed in Chapter 12, the difference will appear in the outer flow which then, although still axisymmetric, must be obtained from a nonlinear equation rather than from the linearized (6-21) as in the sub – or supersonic case. The form of the differential equation for the outer flow does not affect the statements (a) and (b) above, how­ever, and it turns out that the validity of the equivalence rule is less restricted for transonic than for sub – or supersonic flow so that it can then also be used for configurations of moderate aspect ratio provided the flow perturbations are small.