Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Reentrant Jet Flow Near the Leading Edge with a Deflected Tip
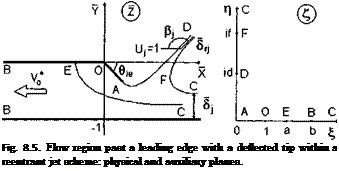
In order to analyze the possibilities of controlling the lifting capacity of a winged vehicle in the ground effect and of enhancing the efficiency of power- augmented modes of operation, the local problem of practical interest is that of flow past a leading edge with a deflected tip. Herein, this problem is treated within the reentrant jet formulation. The anticipated effect consists of generating of pressure thrust on a deflected part of the leading edge with the goal of reducing the drag and, consequently, the installed thrust required to provide power-augmented lift of a vehicle of a given weight. As in the previous analysis, the problem is solved by the method of singular points. The flow patterns in the physical plane £ and the auxiliary plane ( are shown in Fig. 8.5.
Stretching of coordinates is carried out in the following way:
x — 1
The complex conjugate velocity in the auxiliary plane ( is
(8.19)
The exponent accounts for the violation of conformity at point О with C = 1, where the angle between the adjoining parts of the solid surface changes when passing from Z to the auxiliary plane £. The derivative of the complex velocity potential in auxiliary plane £ is
dttf C(C2-a2)
dC (C2+d2)«2-b2)’
 |
Therefore,
a. b.
![Подпись: The unknown constants are determined from the following conditions: 1 / dw TTN a2+ d2 = ~ 2i Jid dC _ 2 b2 + d2 ~ rJ’ (8.22) 1 f dw nN b2 — a2 ~ 2i Л dC ^ “ 2 b2 + d2 ~ V°' (8.23) It follows from the condition of mass conservation that Qc = QB + QD, Qc = Sy (8.23a) Therefrom ■= „ _ irN = QB + QD = — (8.24) and, in addition, x b2 + d? * 5> 62 — a2 V°’ (8.25) because ^(id) = ехр[-(тг - /3j)i] = - exp(i/?j), (8.25a) — arctan d + arctan — = ^le ——, 7Г a 2 (8.26) and * dw/l4 b — a/b— Іч^іе/тг V° = iZm = b + aU + l) ' (8.27) The velocity v* is determined by matching with the channel flow, for example, with a solution of equation (4.95). Because ZA = bef exp(—іве) and а N = —28-Jit, there is one more equation](/img/3131/image904.gif) |
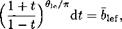(8.28)
where bief is the chord of the deflected part of the leading edge (as a fraction of the distance of the hinge point above the ground). Thus, the system of three equations (8.25),(8.27), and (8.28) was obtained for determining the three unknowns a, 6, and d. The magnitude of /3j is determined from (8.26). The coefficient of the normal force acting on the deflected part is given by
 |
 |
Щ Ґ t(t-a)2 /1
7Гbef Jq (t2 + d2)(b2 – t2) VI + t)
These results facilitate the analysis of the influence of parameters of the deflection of the leading edge tip upon the efficiency of power-augmented regimes.
