Future Perspectives

Scaling laws have clearly established that MAVs and small natural flyers cannot operate with the same lift and thrust generation mechanisms as larger aircraft and that moving wings are needed to conduct the flight mission. Furthermore, these flyers can substantially benefit from active and passive morphing for flight performance enhancement and control, just like the flyers that we see in nature. For example, Lee et al. [530] investigated longitudinal flight dynamics of a bio-inspired ornithopter with a reduced-order aerodynamics model, including wing flexibility effects. They showed that this ornithopter is robust to external disturbances due to its trimmed flight dynamic characteristics that limit cycle oscillation. Furthermore the mean forward flight speed increases almost linearly with the flapping frequency. Inspiration from nature will give us insight on how to manage the complex flight environment. Still we need to keep in mind there is no single perfect mechanism that all flyers should adopt. It is ultimately up to the scientists and engineers to figure out the best combination of the available techniques for a given flyer to suit its size, weight, shape, and flight environment, including wind gust, and mission characteristics.

A variety of wing kinematics and body/leg maneuvers are observed in flyers of various sizes and even in different flight missions of the same flyer; these include takeoff, forward flight of varying speeds, wind gust response, hover, perch, threat avoidance, station tracking, and payload variations. Flying insects are known to execute aerial maneuvers through subtle manipulations of their wing motions. For example, as reported by Bergou et al. [531], fruit flies asymmetrically change the spring rest angles to generate rowing motion of their wings during sharp-turning flight. Also, Combes and Dudley [532] have observed that bees flying in outdoor turbulent air become increasingly unstable about their roll axis as air speed and flow variability increase. The bees are reported to extend their hind legs ventrally at higher speeds, improving roll stability, but also increasing body drag and associated power requirements by 30 percent. Our knowledge of these complex dynamics and our understanding of the influence of the wing-body interaction in the flapping wing aerodynamics are largely incomplete.

A topic that needs to be addressed more comprehensively is the interaction between fore – and hindwings, and the resulting force and flight control implications. For example, it is well known that dragonflies have the ability to control aerodynamic performance by modulating the phase lag between forewings and hindwings. Wang

Figure 5.1. The relative sizes of the insect’s fore – and hindwings are different between species, and consequently, the phase relationship of their wing movement also varies.

and Russell [533] filmed the wing motion of a tethered dragonfly and computed the aerodynamic force and power as a function of the phase. They found that the out – of-phase motion as seen in steady hovering uses nearly minimal power to generate the required force to balance the weight, and the in-phase motion seen in takeoffs provides an additional force for acceleration. Thomas et al. [258] and Wang and Sun [534] studied the fore- and hindwing interactions via flow visualization and computational simulations, respectively. Brackenbury [535] used high-speed flash photography to analyze wing movements in more than 30 species of butterflies. He observed that early in the upstroke the wings show pronounced ventral flexure that, combined with inertial lag in the posterior parts of both wing pairs and delayed supination in the hindwing, leads to the formation of a funnel-like space between the wings. As shown in Figure 5.1, the relative sizes of fore – and hindwings differ between species, and consequently, the phase relationship of their wing movement also varies. The wing-to-wing interactions are complicated due to a large number of parameters involved. Depending on the flight mission (takeoff, climbing cruising, landing), the fluid physics, such as the leading-edge vortex, wake, and tip vortices; the wing geometry including size, shape, and aspect ratio; and the structural flexibility of the wings all play roles in coupled manners. Wings and body interactions and relative movement are so far largely open research topics.

Much work remains to be done in modeling robust aerodynamics models that can be used to develop control strategies and designs of flapping wing MAVs. As discussed in Chapter 3 most investigations attempting to reduce the complex aero­dynamics of flapping wings are fragmented. There is a need for a coherent sys­tematic effort involving simplified analytic theories, high-fidelity numerical simu­lations, and experimentalists to discern the “quasi-steadiness” of the forces on a flapping wing and to document the validity of these simplified models for various configurations and dimensionless parameters, such as k, St, phase lag, and so on. As already reviewed, Gogulapati and Friedmann [367] offer an updated approach to treat the flapping wing aerodynamics in a simplified framework. Progress is being made in this direction; however, more comprehensive guidelines are needed to estab­lish viscous flow features such as leading-edge vortices and wakes, which depend on these dimensionless parameters. Furthermore, if a wing is flexible, then the viscous and flexible structure interaction becomes significantly more complicated. Gogulap – ati et al. [536] have made an effort in this direction while awaiting the development of a more rigorous approach.

As discussed in Chapter 4, the translational forces resulted from the quasi-steady model for the uncorrected (based on nominal AoA), corrected with the downwash for the flexible, and the rigid wings are highlighted in the figure as well. Clearly, the modifications in (i) effective AoA and (ii) shape deformation affect the aerody­namic performance significantly. The quasi-steady model’s performance can improve noticeably if we know how to correct the effective, instantaneous AoA as well as shape deformation. Without knowing the instantaneous flow field comprehensively, such corrections are difficult to make. More efforts are needed in establishing better guidelines to use such simplified aerodynamic models more effectively.

Regarding the dynamics and stability of a flight vehicle in association with flap­ping wing aerodynamics, Orlowski and Girard [537] presented a recent overview of various analyses of flight dynamics, stability, and control. Although efforts are being made to use the multi-body flight dynamics model to predict the behavior of flapping wing MAVs, the majority of the flight dynamics research still involves the standard aircraft (6DOF) equations of motion [537]. Furthermore, the investigations of the stability of flapping wing MAVs have so far been essentially limited to hover and steady forward flight, with most studies focusing on linear, time-invariant aspects on the basis of reference flight conditions. Based on such approaches, flapping wing MAVs have been found to be intrinsically unstable in an open loop setting [538]-

[544] . Moreover, control of flapping wing MAVs has been largely investigated by neglecting the mass of the wings on the position and orientation of the central body

[545] -[549]. As summarized in Chapter 1, the ratio between the wing mass and the mass of the vehicle can be of order 0(1), which means that the motion of the wing can affect vehicle dynamics and stability. Natural flyers are often sensor-rich, and these sensors can offer necessary information needed for real-time flight maneu­vers in uncertain and unpredictable flight environments. For example, vision-based sensing techniques can be very helpful for flight control as well as for estimating the aeroelastic states of the vehicle.

It is established in Chapter 4 that local flexibility can significantly affect aero­dynamics in both fixed and flapping wings. Preliminary research has been reported the vehicle stabilization via passive shape deformation due to flexible structures [550]. Furthermore, as already discussed, insects’ wing properties are anisotropic, with the spanwise bending stiffness about 1 to 2 orders of magnitude larger than the chordwise bending one. As the vehicle size changes, the scaling parameters cannot all maintain invariance due to the different scaling trends associated with them. This means that, for measurement precision, instrumentation preference, and the like, one cannot do laboratory tests of a flapping wing design using different sizes or flap­ping frequencies [450] [ 551]. A closely coordinated computational and experimental framework is needed to facilitate the exploration of the vast design space (which can have O(102) or more design variables including geometry, material properties, kinematics, flight conditions, and environmental parameters) while searching for optimal and robust designs.

Natural flyers and swimmers share many similarities in terms of the physical mechanisms for locomotion. Although lift is more important for flight than for swimming, pitch, plunge, and flexible structures are all clearly observable in force generation. The difference in density between air and water directly influences the structural properties and some other parameters. However, from a scaling viewpoint, these differences can be linked via the same dimensionless parameters. Much of the same scaling laws are equally applicable to swimming as to flight [37] [38] [41] [186]

[552] [553].

Further, cross-fertilization has been regularly achieved in areas related to loco­motion, energetics, morphology, and hydrodynamics. Of course, more interactions are to be expected. For example, Weihs [554] used the slender body theory from aerodynamics to study the turning mechanism in fishes. He showed that the turning process includes three stages, distinguished by different movements of the center of mass. In the first and third stages the center of mass moves in straight lines in the initial and final directions of swimming, whereas in the middle period it moves along an approximately circular connecting arc. The forces and moments acting on the fish can be satisfactorily predicted by treating the vortex wake by the circulation shed from the fins. Weihs [555] also showed that fish can swim more efficiently by alternating periods of accelerated motion and powerless gliding. His analysis of the mechanics of swimming showed that large savings of more than 50 percent in the energy required to traverse a given distance can be obtained by such means. In cal­culations based on measured data for salmon and haddock, the possibility of range increases of up to three times the range at constant speed was demonstrated. Wu

[553] reviewed the forward swimming motion, bird flight approximated by oscillating wings, and insect flight with the high-lift generation via LEVs. From these studies he derived mechanical and biological principles for unified studies on the energetics in deriving metabolic power for animal locomotion. Studies such as these can offer significant insight into analyzing and designing flapping wing flyers.

Biological and physical scientists and engineers can benefit from close collabo­ration to better understand features that enable natural flight. For instance, although wings produce lift required for flight in natural systems, not all wing features are flight related. Honeybees employ short-amplitude high-frequency strokes when they hover [556]; these strokes generate high lift as these flyers consume floral nectar contain­ing high energy, which enables them to carry loads or perform high-power-required maneuvers or missions. However, these strokes are shown to be aerodynamically inefficient [216] [351] [404]. Furthermore, bird wings, bat wing membranes, insect wings, and the like have some interesting but widely varied material properties, which can be used for flapping MAV development. These bio-inspired mechanisms include, for example, joints and distributed actuation to enable flapping and morph­ing. Another topic of much interest but that has so far not been adequately covered is the flight envelope from takeoff to landing. For flapping flyers, key advantages are the variable speed and flapping kinematics along with shape deformation, resulting in highly maneuverable flight characteristics. Furthermore, depending on each species’

Figure 5.2. The Formosan (Taiwan) Barbet (Megalaima nuchalis), nests in tree cavities, and, as shown in the upper row, forms a noticeably inflated body contour immediately prior to takeoff from the cavity. As shown in the lower row, it also balances delicately between speed and position control to land.

biological development, its key features in takeoff and landing/perching can vary more than the flapping patterns in comparison to other flyers of comparable sizes. An interesting example is the Formosan (Taiwan) Barbet (Megalaima nuchalis), which nests in tree cavities (see Fig. 5.2). Consequently, they take off with distinctive body movement to generate the initial thrust, including a noticeably inflated body contour immediately prior to take-off. The landing requires a balancing act between speed and position control (see Fig. 5.2.).

Birds enjoy maneuvering flexibility by combining wings and tails, especially dur­ing take-off and landing. However, depending on the individual cases, the relative size of the tail varies significantly. While the parrot and magpie exhibit relatively longer tails than most birds, some birds feature noticeably short tails. These char­acteristics have major implications on aerodynamics, active and flexible structures, and above all, flight control. Figure 5.3 highlights these features. Another interesting features related to bird flight are that the shape of a wing can be apparently less than streamlined. As shown in Figure 5.4, during takeoff, feather can intrude into the surrounding flows, whose impact is yet to be quantitatively characterized.

Another example is related to hovering. In Chapter 1, it is reported that many birds and bats hover with the stroke plane inclined about 30 degrees to the horizon­tal, and the resultant downward stroke force with a lift-to-drag ratio of 1.7 is vertical [70]. However, flight environment, especially wind, and vehicle size, can cause strong impact on the hovering pattern. Two small birds, the Ruby-throated Hummingbird (7-9 cm total length) and the Light-vented Bulbul (Pycnonotus sinensis), also known as the Chinese Bulbul (15-20 cm long), can be used as examples here. The flap­ping of a hummingbird is on a horizontally-inclined stroke plane with a symmetric

Figure 5.3. Birds enjoy maneuvering flexibility by combining wings and tails, especially during take-off and landing. However, depending on the individual cases, the relative size of the tail varies significantly. While parrot and magpie exhibit relatively longer tails, some birds feature noticeably shorter tails, resulting in apparently different flight control patterns.

Figure 5.4. During takeoff, feather in certain portion of the bird wing can intrude into the surrounding flow field.

Figure 5.5. Hummingbirds utilize wing-wail coordination and varied inclination toaccommo – date wind.

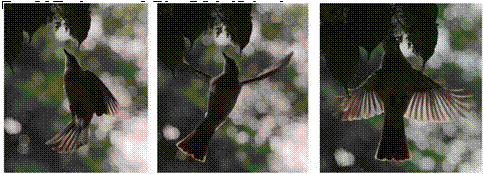
figure-eight pattern. Furthermore, as shown in Figure 5.5, due to its small size and light weight, a hummingbird frequently utilizes wing-tail combination and flexi­ble wing structures to accommodate wind gust. Unlike the hummingbird, the bulbul flaps asymmetrically while hovering. As shown in Figure 5.6, in the downward stroke, bulbuls’ horizontally inclined wings move while being rotated. During the upward stroke, the wings are initially flexed and then spread out horizontally. Consequently, lift is generated during the downward stroke; the forward and backward thrusts cre­ated during different strokes cancel each other. In short, hummingbirds and bulbuls, both small in size, use distinctively different flapping kinematics, wing morphology and structural flexibility while hovering. Many outstanding issues related to flapping

wing aerodynamics are to be investigated even for commonly observed phenomena. Such complex and varied flight patterns vividly show how much room we have to further learn from nature. By working across the scientific disciplines, scientists and engineers will be able better equipped to uncover the magic of natural flyers’ amaz­ing performance as well as contribute to the advancement of the human-engineered MAVs.

[1] The Passeriform model (herons, falcons, hawks, eagles, and owls): s ~ m078

[2] The Shorebird model (doves, parrots, geese, swans, and albatross): s ~ m071.

[3] The Duck model (grebes, loons, and coots):s ~ m078.

These relations are consistent with those presented in Table 1.3 for all birds other than hummingbirds.