Elementary Beam Theory

Now that we have considered the fundamental aspects of structural dynamics analysis for strings, these same concepts are applied to the dynamics of beam torsional and bending deformation. The beam has many more of the characteristics of typical aeronautical structures. Indeed, high-aspect-ratio wings and helicopter rotor blades are frequently idealized as beams, especially in conceptual and preliminary design. Even for low-aspect-ratio wings, although a plate model may be more realistic, the bending and torsional deformation can be approximated by use of beam theory with adjusted stiffness coefficients.

2.3.1 Torsion

In an effort to retain a level of simplicity that promotes tractability, the St. Venant theory of torsion is used and the problem is idealized to the extent that torsion is uncoupled from transverse deflections. The torsional rigidity, denoted by GJ, is taken as given and may vary with x. For homogeneous and isotropic beams, GJ = GJ, where G denotes the shear modulus and J is a constant that depends only on the geometry of the cross section. To be uncoupled from bending and other types of deformation, the x axis must be along the elastic axis and also must coincide with the locus of cross-sectional mass centroids. For isotropic beams, the elastic axis is along the locus of cross-sectional shear centers.

For such beams, J can be determined by solving a boundary-value problem over the cross section, which requires finding the cross-sectional warping caused by

torsion. Although analytical solutions for this problem are available for simple cross­sectional geometries, solving for the cross-sectional warping and torsional stiffness is, in general, not a trivial exercise and possibly requires a numerical solution of Laplace’s equation over the cross section. Moreover, when the beam is inhomo­geneous with more than one constituent material and/or when one or more of the constituent materials is anisotropic, we must solve a more involved boundary-value problem over the cross-sectional area. For additional discussion of this point, see Section 2.4.

Equation of Motion. The beam is considered initially to have nonuniform properties along the x axis and to be loaded with a known, distributed twisting moment r(x, t). The elastic twisting deflection, в, is positive in a right-handed sense about this axis, as illustrated in Fig. 2.3. In contrast, the twisting moment, denoted by T, is the structural torque (i. e., the resultant moment of the tractions on a cross-sectional face about the elastic axis). Recall that an outward-directed normal on the positive x face is directed to the right, whereas an outward-directed normal on the negative x face is directed to the left. Thus, a positive torque tends to rotate the positive x face in a direction that is positive along the x axis in the right-hand sense and the negative x face in a direction that is positive along the – x axis in the right-hand sense, as depicted in Fig. 2.3. This affects the boundary conditions, which are discussed in connection with applications of the theory in Chapter 3.

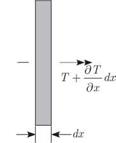
Letting p Ipdx be the polar mass moment of inertia about the x axis of the differential beam segment in Fig. 2.4, we can obtain the equation of motion by equating the resultant twisting moment on both segment faces to the rate of change of the segment’s angular momentum about the elastic axis. This yields

d T d 2 в

T + – dx – T + r(x, t)dx = pIpdx —2 (2.39)

d x d t2

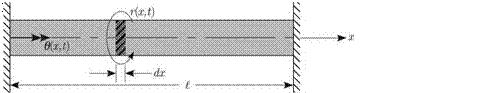Figure 2.4. Cross-sectional slice of beam undergoing torsional deformation

or

d T __

– + r (x.<) = p I, ^ (2.40)

where the polar mass moment of inertia is

p Ip = jJ p (y2 + z2) dA (2.41)

A

Here, A is the cross section of the beam, y and z are cross-sectional Cartesian coordinates, and p is the mass density of the beam. When p is constant over the cross section, then p I, = p Ip, where I, is the polar area moment of inertia per unit length. In general, however, p I, may vary along the x axis.

The twisting moment can be written in terms of the twist rate and the St. Venant torsional rigidity GJ as

T = GJ —

д X

-д 2в

Substituting these expressions into Eq. (2.40), we obtain the partial-differential equation of motion for the nonuniform beam given by

Strain Energy. The strain energy of an isotropic beam undergoing pure torsional deformation can be written as

1 7 ^ / дв 2

U = 2 I GJ{~x)dx (2.44)

This is also an appropriate expression of torsional strain energy for a composite beam without elastic coupling.

Kinetic Energy. The kinetic energy of a beam undergoing pure torsional deforma­tion can be written as

Virtual Work of Applied, Distributed Torque. The virtual work of an applied dis­tributed twisting moment r (x, t) on a beam undergoing torsional deformation may be computed as

S W = f r (x, t)SO (x, t)dx (2.46)

J0

where SO is the variation of O (x, t), the angle of rotation caused by twist. Note that SO may be thought of as an increment of O (x, t) that satisfies all geometric constraints.