The Quartic Characteristic Equation
We consider first the case when the cruise speed remains constant
in otherwise perturbed motion. In what follows, all quantities and functions will be rendered nondimensional with respect to the cruise speed U0 and the root chord C0 of the main wing of the vehicle. In this case, the equations of perturbed uncontrolled motion of the WIG can be written as
![]()
![]()
![]() d2h 1 dt2 =
d2h 1 dt2 =
d20
Mb-^2 = mz’
where /j, is relative density of the vehicle defined by the formula
2 M pSC0′
|
Iz MC02’ |
In (11.1) and (11.2), Cy and fhz are the perturbed lift and the moment coefficients, h and 6 represent the perturbed relative ground clearance and the pitch angle, M is the vehicle’s mass in cruise, S is the wing’s reference area, p is the specific density of air, and iz is a coefficient of the longitudinal moment of inertia of mass, determined by the relationship
where Iz is the longitudinal moment of inertia of mass with respect to the center of gravity. To track the relationship between the dynamic stability of the vehicle and its cruise speed, it is sometimes convenient to use the following obvious equation:
P — Fr2Cyo, Fr = -^p, (11.5)
where Fr and Cyo are correspondingly the cruise Froude number and the cruise lift coefficient. Accounting for (11.1), (11.2), and (11.5), the (two)
equations of motion with respect to the perturbations of pitch and ground clearance can be written in an alternative form as
|
Fr2C A2’h _ c pr2C – fh *Г ^Уо “ ^2/’ ^yo %z ^2 ~т*’ |
(11.6) |
|
Using a representation of the perturbed lift and the moment coefficients in terms of the derivatives with respect to the principal kinematic parameters 9 and /1, we can write |
|
|
cy = c9e + с* К + с9 ё + c% |
(11.7) |
|
rhz = mez9 + m^h + raf 0 + m^h. (11.8) Now we can rewrite the equations (11.7), (11.8) in the following form: |
|
|
^ ^ Г1*1 ^ Гh — ^ 1 г*о Л ^~Су1л~Сук-СуЦ + Сув’ |
(11.9) |
|
d 4 id6 idh,, "**d?“m’d?“m-e = m’ ii+m’h- |
(11.10) |
|
Excluding h or 9 from the equation set (11.9), (11.10), we obtain the following fourth-order (quartic) characteristic equation of the system: |
|
|
D4 + A1D3 + A2D2 + AZD + A4 = 0, |
(11.11) |
|
where the coefficients Ai(i = 1,2,3,4) are |
|
|
A‘ = – A(mi + i, Ci), ftLz |
(11.12) |
|
A2= 2. [c9mhz СуГп9 + ц(т9 +1 zCy), ft I’z |
(11.13) |
|
A3 = 4~(тІСу + <СІ – С№ – СУ<)> ft 1Z |
(11.14) |
|
■4. = -4гКс;-С,»тЙ, ft Lz |
(11.15) |
The last coefficient can be rewritten to be expressed in terms of the abscissas of the aerodynamic centers of height xh and of pitch 9:
The stability of motion for the fourth-order equation will be ensured if
Ai, Л-2-, Аз, A* > О A2A3 — A1A4 — A3 > 0. (11.17)
It can be shown from consideration of formulas (11.12)-(11.17) that conditions Ai > О, A2 > 0 and As > 0 always hold. Hence, the requirements of stability in proximity to the ground can be reduced to the two inequalities A4 > 0 and A1A2AS — A1A4 — A > 0. Satisfaction of the former condition provides aperiodic stability to the craft, i. e., the absence of positive real roots of the characteristic equation. Meeting the latter requirement ensures oscillatory stability, i. e., the absence of positive real parts of the complex roots of the characteristic equation. It follows immediately from these considerations and observation of (11.16) that to secure the aperiodic stability of wing-inground-effect vehicle, one should select an aerodynamic configuration of the craft for which the center of height is located upstream of the center of pitch, i. e., — xq > 0 (note that here the x axis is directed upstream).











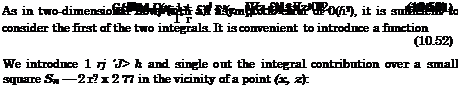
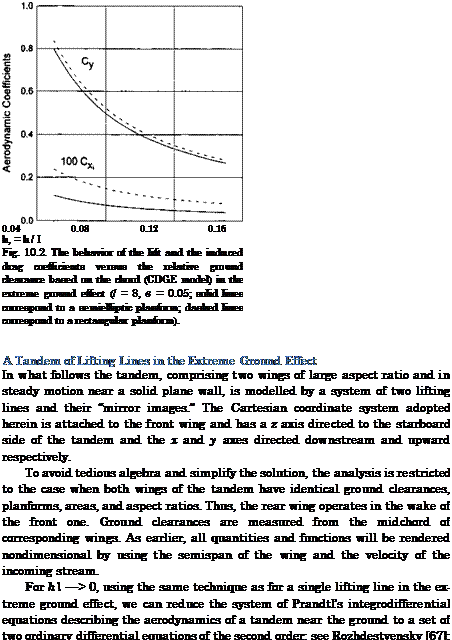
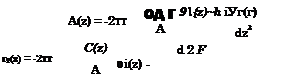

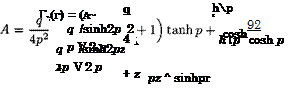
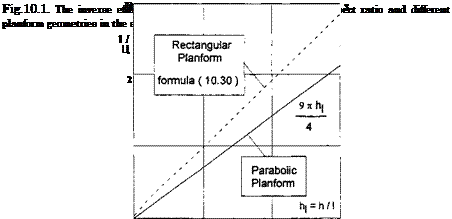
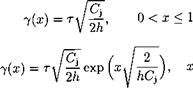 (10.17)
(10.17)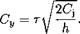 (10.19)
(10.19)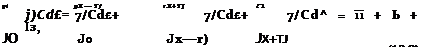 1 =
1 =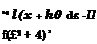 For I2, the variable of integration varies in the range of x – r? <£< x + rj. We introduce a new stretched variable £ = (£ — x)/h, which has the order of 0(1). Then, _ _
For I2, the variable of integration varies in the range of x – r? <£< x + rj. We introduce a new stretched variable £ = (£ — x)/h, which has the order of 0(1). Then, _ _