Category An Introduction to THE THEORY OF. AEROELASTICITY

13.1 THE PROBLEM SPECIFIED

The problem of an oscillating thin airfoil in a two-dimensional incom­pressible flow will be considered in this chapter. The mean motion of the airfoil is a rectilinear translation of speed U with respect to the fluid at infinity. To this mean motion a simple-harmonic oscillation of infinitesimal amplitude is superposed. Since an arbitrary motion of an airfoil can be analyzed into harmonic components by means of a Fourier analysis, and, conversely, by a synthesis of the simple-harmonic com­ponents, any general motion can be established, the analysis of harmonic oscillations actually forms the basis of a general airfoil theory in unsteady motion (of small amplitude).

The airfoil to be considered is a planar system. The treatment will be limited to a linearized theory.

Within the framework of a linearized theory, solutions may be super­posed to generate another solution. The solution of an oscillating airfoil with finite but small thickness and camber at a given mean angle of attack can be obtained by a superposition of an unsteady solution for an oscill­ating airfoil of zero thickness and zero camber at zero mean angle of attack, and a steady-state solution for an airfoil of the given thickness and eamber at the given mean angle of attack. The steady-state solution can be found in many textbooks on aerodynamics. Therefore, in discussing the aerodynamics of an oscillating airfoil, it is sufficient to consider an airfoil of zero thickness and zero camber at zero mean angle of attack.

The two-dimensional nonstationary airfoil theory was first formulated by Birnbaum and Wagner. A short historical review of the earlier works of Kiissner, Glauert, and Theodorsen is given in § 5.8. More recent developments were made by Cicala, Kiissner, Schwarz, von Karman, Sears, Dietze, W. P. Jones, Biot, and others. See bibliography.

The thin-airfoil problems can be treated by the usual methods of mathematical physics. The three commonly used methods are:

1. Superposition of proper singularities. Examples are the super­position of sources and sinks to obtain a symmetric body or of the source-sink doublet layer to obtain a lifting surface.

2. By a transformation which reduces a given boundary-value problem to an easier problem, or to a problem the solution of which is known. For two-dimensional incompressible flow, the conformal transformation is a powerful method, because the theory of functions of a complex variable furnishes the necessary tool to carry out the operations. The well-known Lorentz transformation for the wave equation may be regarded as an example of conformal transformation in a four-dimensional space whose metric is defined as

ds2 = r&2 + dy2 + dz2 — a2 dt2

3. Operational methods, such as Laplace transformation or the Fourier transformation.

Each method has its advantages and difficulties. Most of the airfoil problems can be (and have been) solved by all three methods. In the following presentation, however, we shall choose only the shortest ones.

An indisputable boundary condition is that the fluid must not penetrate the solid body so that the flow must be tangent to the solid surface at all times.[34] The other boundary condition, which in the case of a fluid of infinite extent refers to the conditions of flow at infinitely large distance from the airfoil, requires a careful consideration.

In an incompressible fluid, the influence of any disturbance is instantly transmitted in all directions to infinity. Consider the motion of a fluid generated by the motion of an airfoil which begins at a time t0. During a finite time interval t tQ9 the airfoil moves about and sweeps out a region of space, every point of which is occupied by the airfoil at one time or another. Let this region be denoted by s4. Consider now a spherical surface with radius R0 so large that the entire region, я/ is enclosed in the sphere. Introduce the spherical polar coordinates, R, в, y>, where в and ip are the polar and azimuth angles, respectively. Let the resultant velocity at a point (R. в, ip) be V(R, в, ip). The total kinetic energy of the fluid in the region outside the sphere R0 is

і |*co |*it f*2it

K. E. = 2 Jb Jo Jo pr’:’Ri sin 6 dR d0 cbp

This must be a finite quantity because it is only part of the energy imparted to the fluid by the motion of the airfoil. Therefore the improper integral must be convergent, and it is seen that the velocity V must decrease to zero as R increases indefinitely at a rate faster than l/Jtk Thus the condition at infinity for an incompressible fluid is that the velocity dis­turbance decreases to zero, or that the velocity potential tends to a con­stant. The relation between the velocity and acceleration then shows that the acceleration of the fluid particles decreases to zero at infinity, while the acceleration potential and pressure tend to constant values at infinity.

If the airfoil is moving in a compressible fluid, the disturbance in the fluid due to the motion of the airfoil is propagated with the speed of sound. If the speed of motion of the airfoil (relative to a coordinate system which is at rest with respect to the fluid at infinity) is less than the speed of sound, the disturbance will be felt in all directions. After a sufficiently long period of time, the region of the fluid influenced by the motion of the airfoil will be much larger than the region sd swept out by the airfoil, and the argument of the last paragraph can be used again to conclude that the velocity disturbances decrease to zero at infinity.

If the motion of the airfoil is a small oscillation about a rectilinear translation, which has taken place for an indefinitely long time, the region ja/ named above consists of the volume occupied by the airfoil and a wake which extends indefinitely behind the airfoil. The conclusions reached above may be so stated that the velocity disturbances must vanish at least as fast as 1 /Rsl2 as R -» со, where R is the shortest distance between the point in question and the airfoil and its wake.

Consider finally the case in which the airfoil is moving at a supersonic speed. The speed of motion being higher than the speed of propagation of sound, the disturbances cannot be felt in front of the envelope of Mach waves generated by the leading edge of the airfoil. Hence, there is no disturbance upstream of the airfoil. On the other hand, at supersonic speeds, energy can be propagated to infinity in the form of shock waves. In a two-dimensional supersonic flow, the region influenced by the shock waves may be limited in extent, and the strength of disturbance does not necessarily diminish toward zero when the distance from the airfoil increases indefinitely. However, we may impose the condition that there are no sources of disturbance in the fluid other than the airfoil under consideration. This, together with the condition of no disturbance in front of the Mach-wave envelope from the leading edge, suffices to determine a unique solution in the supersonic case.

In the following discussions, we shall restrict ourselves to the linearized theory, which is valid only if the disturbance caused by the airfoil is infinitesimal. This implies that the wing is infinitely thin and of infinitesimal camber and has an infinitesimal angle of attack. Such a wing is said to be planar.

Let the free-stream direction be parallel to the mean position of the planar wing, which lies on the (хъ хг) plane. Then the distance from the wing surface to the (хъ x2) plane is an infinitesimal quantity of the first order. On the assumption that the quantities p’, p, щ, a{, etc., are continuous functions of space and time, it is easy to see (by expansion into power series) that the difference of any of these quantities on the surface of the airfoil from that on the (aq, ж2) plane is an infinitesimal of higher order than that quantity itself. Therefore, in the linearized theory it is permissible to apply the boundary conditions of a planar system in a region on the (xlt a:2) plane that represents the projection of the airfoil on that plane, instead of oh the actual airfoil surface.

Euler’s equations of motion for a piezotropic fluid

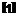Dut 1 3p Э Cp dp

Dt p dx{ dxt Jp, p

indicate that the acceleration vector of a fluid particle,

is a gradient of a scalar quantity. Hence at can be derived from a scalar function ф(хг, x2, x3, t) so that

The function ф is then called an acceleration potential of the flow field.

Substituting Eqs. 3 into Eq. I and integrating with respect to space, we obtain

ф + f — = const (4)

Jp о p

The integration constant may be a function of time. But, if there is no change in the conditions at infinity, it is a pure constant.

і

— is a continuous function of p

Pa P

which is continuous everywhere except at a shock wave. In the thin – airfoil theory, shock waves of finite intensity do not exist; hence, in view of Eq. 4, the acceleration potential is continuous everywhere. This is in sharp contrast with the velocity potential, which does not exist in the wake. When the wake is idealized into a surface without thickness, the velocity potential is discontinuous across that surface, but the acceleration potential is continuous. This advantageous fact is very important in the application of the acceleration potential to the airfoil theory.

Further simplifications are obtained by linearization. Let us consider small disturbances in an otherwise uniform, rectilinear, steady flow. Let the deviation of each velocity component щ (і = 1, 2, 3) from the uniform, steady value be so small that щг is negligible in comparison with |и,-| itself. The corresponding changes in pressure and density of the fluid, according to the equations of motion, are also infinitesimals of the first order. Hence writing

P = Po + P’y P=Po+P’

where p0, p0 are the initial density and pressure and p, p’ are the small perturbations, then p’2 p’, p’2 p’. We have, from Eq. 4, taking ф

at p0 to be zero,

when small quantities of the second and higher orders are neglected. Hence, the acceleration potential ф is directly proportional to the pressure disturbance in the fluid. This offers a simple physical interpretation of the acceleration potential in a linearized theory.

The linearized field equations of the acceleration potential can be derived as follows.

Let us take a reference coordinate system which has no relative motion with respect to the fluid at infinity, and consider small perturbations of the velocity field so that the squares and higher powers of the velocity com­ponents or their space and time derivatives are negligible in comparison with their first power. Let p0 and p0 be the pressure and density of the undisturbed fluid, p’, p the small perturbations, and let щ be the velocity components; then the Eulerian equations of motion and the equation of continuity are linearized into

respectively. Differentiating Eq. 6 with respect to x{, and Eq. 7 with

32w.

respect to t, and eliminating the sum – we obtain

dt dxt

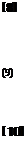эу эу

dxt dxi dt2

For a piezotropic fluid, if we write

dp

dp

then Eq. 8 can be written as

эу і эу

dxidxi a2 dt2

which is the fundamental wave equation in acoustics for a fluid stationary at infinity.

When small perturbations are considered,

If we neglect the second – and higher-order terms, Eq. 10 becomes

The quantity a0 is the speed of propagation of a sound wave in the un­disturbed fluid. Generally a0 is written as

to signify that the derivative dpjdp is taken at the condition of constant entropy. That a0 is the velocity of propagation of a sound wave can be seen from the following example. Consider a spherical disturbance. By spherical symmetry, Eq. 11 can be transformed in spherical polar co­ordinates to

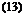
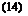2дф

C>2 r dr a02 сП’1

A general solution of this equation is

ф = lf(r – a0t) + ^g(r + Oq0

where / and g are arbitrary functions. Clearly the first term represents a wave radiating out from the origin, and the second term one converging toward the origin, both propagating with a speed a0.

Since the acceleration potential is proportional to the perturbation pressure pr, the acceleration potential ф is governed by the same equation

дЧ 1 д2ф

Эх{ дх,; ~ V2 д?- ~ ° (15)

Differentiating Eq. 15 with respect to xjy we see that the components of acceleration = дф/дх} satisfy the same equation. Further, since p = (1 /a0V + higher-order infinitesimals, we see that p is governed by the same equation. Finally, since at = du^dt in the linearized theory, the wave equation for the components of acceleration can be integrated to show that the velocity components ut as well as the velocity potential Ф are also governed by the same equation.

If the fluid is incompressible, p = const; then a is infinity, and Eq. 15 becomes

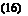34

Эxt 2>x{

For an incompressible fluid in a two-dimensional flow, the governing equation

(17)

shows that there exists a function ip conjugate to ф so that w = ф + jip is an analytic function of z=x + jy. w(z) may be called a complex acceleration potential. Similar to the complex velocity function of § 12.4, the complex acceleration function

dw

— = ax-jay (18)

is the reflection of the acceleration vector in the x axis

If the velocity vector can be derived from the gradient of a scalar function Ф:

u = УФ

then the flow field is irrotational,

У X u = 0 (i. e., curl u = 0) (2)

since У X УФ = 0 is an identity for any scalar function Ф. The function Ф is called the velocity potential of the flow field.

If the fluid is incompressible, the equation of continuity

Эи,-

Эх,

which is the equation governing Ф for an incompressible fluid.

According to § 12.2, in thin airfoil theory the flow outside the airfoil and its wake is irrotational and the velocity potential exists.

12.1 COMPLEX POTENTIAL IN TWO-DIMENSIONAL IRROTATIONAL FLOW OF AN INCOMPRESSIBLE FLUID

In a two-dimensional flow in the x, у plane, the velocity has two com­ponents u, v in the x and у direction, respectively. If the fluid is incompressible, the equation of continuity

Эм Эи дх ^ Эу

can be satisfied identically if m, v are derived from an arbitrary function Y so that

The function Y is called a stream function. The reason for this name follows the definition of a streamline, which is a curve the tangent of which is everywhere parallel to the local velocity vector. Thus a stream­line is defined by the equation.

Thus, along a streamline, Y(a:, y) is a constant. Conversely, every con­stant defines a streamline

Y(x, y) = const (5)

If, in addition to being incompressible, the flow is irrotational, then

Э и dv

dy dx

Substituting Eqs. 2, we see that

3aY 9aY dx2 dy2

Thus, for an irrotational incompressible flow, both the velocity potential and the stream function satisfy the Laplace equation.

By definitions of the velocity potential Ф and the stream function Y, we have

и

Hence,

9Y

dy

which shows that the streamlines (Y = const) and the potential lines (Ф = const) are orthogonal to each other.

Equations 9 are the Cauchy-Riemann differential equations of a function of a complex variable. If W(z) = Ф + /’Y is an analytic func­tion of a complex variable z = x + jy, then Eqs. 9 are satisfied. Con­versely, if Eqs. 9 are satisfied, and if the partial derivatives are continuous in a region, then there exists a function W(z) = Ф + у Y which is analytic

in that region. In other words, the velocity potential and the stream function are the real and imaginary parts of an analytic function. Further­more, the real or imaginary part of any arbitrary analytic function can be taken as a velocity potential or a stream function. Ф and Y are said to be conjugate to each other, so that still another way of stating the above fact is that, to every solution of the Laplace equation,

V2<£ = 0

there corresponds a conjugate function Y(a;, у) satisfying the same equation, and that the system of curves Ф = const and Y = const are orthogonal.

In some occasions, Ф may be associated with other physical quantities, such as an acceleration potential or pressure. The corresponding conju­gate function Y may not have an apparent physical meaning, but it serves as a useful medium in the analysis.

The derivatives of the complex potential W(z) — Ф + y’Y has a simple interpretation. Since

dW _ ЭФ,3Y_ dz dx ^ dx

when Ф is interpreted as a velocity potential, dW/dz is the image in the x axis of the velocity vector ux + ]uv. Hence, dWjdz is often called a complex velocity function.

The circulation I(c^) in any closed circuit ґ€ is defined by the line integral

І(Щ = f u • dl (1)

where is any closed curve in the fluid, and the integrand is the scalar product of the velocity vector u and the vector dl, which is tangent to the

curve and of length dl (Fig. 12.1). Clearly, the circulation is a function of both the velocity field and the chosen curve.

Using Stokes’s theorem, the line integral can be transformed into the surface integral

m~j{4xu)ndo (2)

where S is any surface in the fluid bounded by the curve e6, provided that there is no discontinuity in the velocity field. The symbol V means the Id Э Э

vectorial operator I — > —> — I. The vector product V X u, some-

d3lj C%2

times written as curl u, has the following three components in the direction of Xj, Xg, Xg!

If the direction cosines of the outer normal of the surface element do are denoted as пг, n2, n3; then the normal component of the vector V X и is

We define the vorticity at a point in a velocity field as the quantity

X = V x u = curl u (3)

The law of change of circulation with time, when it is taken around a fluid line, i. e., a curve formed by definite fluid particles, is given by the theorem of Lord Kelvin:

Theorem: If the fluid is nonviscous and the body force is conservative, then

Df_ _[ dp Dt J’r p

If, in addition to the above conditions, the fluid is piezotropic, then the last integral vanishes because is a closed curve, and we have the Helmholtz theorem that

(5)

The flow is said to be irrotational if the vorticity is zero throughout the region under consideration.

In the thin-airfoil theory, the conditions of the Helmholtz theorem are satisfied. Hence, the circulation I about any fluid line never changes with

Curve formed by fluid particles that constitute the original curve C-^

Fig. 12.2. Fluid line V enclosing an airfoil
and its wake.

time. Since the motion of the fluid is caused by the motion of the airfoil and since at the beginning the fluid is at rest and I = 0, it follows that I vanishes at all times. Note, however, that the volume occupied by the airfoil is exclusive of the fluid. A fluid line la enclosing the boundary of the airfoil becomes elongated when the airfoil moves forward as shown in Fig. 12.2. According to the Helmholtz theorem, the circulation about ^ is zero, so that the total vorticity inside (<d vanishes, but one cannot conclude that the vorticity actually vanishes everywhere inside. Hence, in the region occupied by the airfoil, and in the wake behind the airfoil,
vorticity may exist, However, the Helmholtz theorem applies to the region outside the airfoil and its wake, and the vanishing of circulation about every possible fluid line clearly shows that the flow is irrotational outside the airfoil and its wake.

In the following the tensor notation will be used. The components of a vector or a tensor are referred to a system of rectangular Cartesian coordinates (xv x2, xj which will be written as (x, y, z) if convenient. The components of a velocity vector will be denoted by (ult u2, us) or by (;и, v, w). Similarly the components of other quantities will be represented either by a subscript or by a self-explanatory triplet of letters, whichever be the more convenient in special instances. The Roman indices always range through 1, 2, 3, unless otherwise stated. The summation con­vention (p. 5) will be used: Any index repeated twice in the same term indicates a summation over the total range of that index.

In the tensor notation the fundamental equations of aerodynamics of a nonviscous fluid are

1. The Eulerian equation of motion (law of conservation of momentum)

2. The equation of continuity (law of conservation of mass)

where p is the fluid density, p the pressure, Fi the force per unit volume acting on the fluid (such as gravitation), щ the velocity components, and /the time. DuJDt denotes the acceleration of a particle of the fluid. To express DuJDt in terms of the space and time derivatives of the velocity field, note that, if the position of an element of the fluid is described by xjt), the velocity of the fluid element is

dx{

//, = — = щ(х1г x2, x3; t)

which is a function of space and time. By the usual rule of differentiation we obtain

Dui Э щ Э u{ dxs Эи і Э uf ~Dt = It + Э*, It = Э? + Щ Ц

The acceleration of a fluid particle is written as DuJDt to distinguish it from the partial derivative dujdt. Using Eq. 3, the equation of motion can be written as

The derivation of these equations can be found in any book on theoretical aerodynamics (e. g., Ref. 1.46). In the airfoil theory con­sidered below, the body force iq can be omitted, since the only significant body force, the gravitation, introduces only a field of hydrostatic pressure which does not concern us. The assumption that the fluid is nonviscous will be made throughout the following discussion, not because the effect of viscosity is unimportant, but because we shall consider only the flow over a thin airfoil at a small angle of attack without separation, in which case the boundary-layer theory shows that the fluid outside the boundary layer may be regarded as nonviscous and the effect of viscosity can be stated in a phenomenological rule that the velocity must remain finite and tangent to the airfoil at the sharp trailing edge. This assumption was put forward by M. Wilhelm Kutta (1867-1944) and Nikolai E. Joukowski (1847-1921) independently, and is called the Kutta-Joukowski condition.

For a compressible fluid, Eqs. 1 and 2 do not suffice in defining uniquely the flow. It is necessary to know also the thermal and caloric states of the fluid and the heat transfer. For an example of the ideal gas, the thermal equation of state is р/р = RT, and the caloric equation of state is given by the relationship between the internal energy and the tempera­ture. These, in addition to an equation expressing the balance of heat and mechanical energy (the first law of thermodynamics), define a flow uniquely for proper boundary conditions.

The analysis can be greatly simplified if it is possible to assume that the fluid is piezotropic, for which the density p is a unique function of pressure. For a piezotropic fluid the potential energy of the fluid can be defined by pressure alone and an integration of the equation of motion along a stream line defines the energy balance completely. Then Eqs. 1 and 2 are sufficient to determine the flow. Fortunately, this is the case in thin airfoil theory, which deals with airfoils of infinitesimal thickness at small angle of attack performing motions of infinitesimal amplitude. The disturbances caused by the airfoil in a flow is thus infinitesimal, and shock waves, if any, will be of infinitesimal strength. No external heat source will be considered. Under these circumstances it can be shown that the change of entropy in the entire field of flow is an infinitesimal quantity of higher order of smallness. Thus the flow may be correctly regarded as isentropic, and the relation

p/pY = const (5)

holds for the entire field, у being the ratio of the specific heats cjcv. c„ is the specific heat of the gas at constant pressure, and c„ is that at constant volume.

Four distinctive ranges of speed, classified according to the magnitude of the free-stream Mach number M, are considered in aerodynamics. These are:

1. The “incompressible” speed range, in which M2 1 and the fluid may be considered incompressible.

2. Subsonic-speed range, M < 1, and below the Mach number of “divergence” (cf. § 4.3).

3. Transonic-speed range, M ~ 1.

4. Supersonic-speed range, M> 1.

This classification is based on a theoretical point of view, particularly on the method of analysis. There is no essential difference in the flow patterns between ranges 1 and 2. But, in range 1, which is a limiting case of 2, considerable mathematical simplifications are possible.

Since airfoil characteristics vary with the speed ranges, the most efficient configuration of an airplane is likely to be different for different design speeds. Thus sweptback wings become favorable for airplanes designed for high subsonic and transonic speeds, and thin delta wings and wings of small-aspect ratios are favored in transonic and supersonic designs. Such differences in the geometrical configurations and the corresponding differences in the structural constructions have important effects on the method of aeroelastic analysis.

In this and the next three chapters the aerodynamics of an oscillating airfoil is studied. We shall consider harmonic oscillations of small amplitudes only, so that in most cases the principle of superposition is applicable. In case the aerodynamic equations may be linearized, the aerodynamic response to an arbitrary motion can be obtained from the response to harmonic oscillations by an integration.

Of the four speed ranges mentioned above, the aerodynamics of an incompressible flow has been exhaustively developed; that of the sub­sonic and supersonic flows is also developed to certain extent. But, in the transonic-speed range, much theoretical and experimental work remains to be done before a reliable analysis can be made.

A step that is always involved in an aeroelastic analysis is the determin­ation of elastic deformation from exterior forces. This can be expressed in operational form by an equation:

= Ostru {Paero + Oiner – f F„} (1)

where % is a vector describing the elastic deformation, Ostru is a structural operator giving % for any system of exterior forces. Oaer0 and 0lner are, respectively, the aerodynamic and inertia operators winch give the exterior forces caused by a given elastic deformation, and F„ is the acting exterior force that is independent of the elastic deformation. The operators Ostru, Oaero can be expressed as integrals with proper kernels.

When % represents the true elastic displacement in the body, the struc­tural operator (or rather the kernel of the integral representing the structural operator) is symmetric according to Maxwell’s reciproca relation, which states that the elastic displacement % at a point A causer by a force F acting at a point В is equal to the displacement (in the direc tion of F) at В caused by a force (acting in the direction of?) at A. Bu the kernel of the aerodynamic operator is in general unsymmetric, becaus the aerodynamic force at A caused by a displacement at В is, in general, different from that at В caused by a “corresponding” displacement at A. Thus Eq. 1, as an integral equation, has an unsymmetrical kernel.

The unsymmetry of the kernel of the basic integral equation 1 may also arise from the structural operator when % represents the displacement in generalized coordinates. For example, the deformation of a slender wing may be described by a deflection w perpendicular to the plane of the wing and a rotation в about a reference axis. Then f may be considered as having two components w and в. Correspondingly the exterior forces are generalized forces: a lift corresponding to w and a moment corres­ponding to в. In this case the structural operator, which consists of integrals of the products of the exterior forces with proper influence functions, becomes unsymmetric in general, because the deflection at a point A due to a couple acting at a point В is in general unequal to the deflection at В due to a couple at A. In other words, the influence function connecting w with the moment about the elastic axis is unsym­metric.

An integral equation with a real symmetric kernel possesses many nice properties that are lost when the symmetry of the kernel is lost. The main mathematical difficulty in aeroelasticity lies in the unsymmetry of the kernels of the governing integral equations.

Symmetric cases are exceptions rather than the rule in aeroelasticity. It is interesting to consider the conditions under which the operators become symmetric. For concreteness let us consider a slender wing. The structural operator becomes symmetric when bending and torsion are “separated,” i. e., when a torsional moment about the elastic axis induces no deflection of that axis and vice-versa. This occurs for an unswept cantilever wing with a straight elastic axis. The aerodynamic operator is simplified when the strip assumption is introduced, according to which the aerodynamic forces and moments at any section of the wing simply depend on the local % and wing chord. The inertia operator is symmetric if linearized. Thus the kernel of Eq. 1, as an integral equation, may become symmetric when bending and torsion of the wing are elastically uncoupled and when the strip assumption for aerodynamic forces is introduced. The problems of torsional divergence and aileron reversal of a normal wing as given in § 3.2 and § 4.2 are examples of this case. The divergence and reversal of a swept wing (§ 4.5) are examples of the unsymmetric case. In the flutter problem, the aerodynamic forces are complex functions of the reduced frequency; the kernel is no longer real valued.

Most problems in aeroelasticity can also be formulated as boundary – value problems in differential equations, which are connected with the integral equations by proper Green’s functions (i. e., the influence func­tions). It is well known that the Green’s functions are symmetric if the boundary-value problem defined by the differential equation is “self – adjoint” (see Collatz).11® The unsymmetry of the aeroelastic operators is associated with the non-self-adjointness of the boundary-value problem.

The stability problems in aeroelasticity are eigenvalue problems. Under certain conditions, among which the Hermitian self-adjointness or the Hermitian symmetry of the kernel is the most important, it can be shown that eigenvalues always exist and are real valued, that the eigenfunctions form a “complete” set of functions, that the iteration procedure for the calculation of the eigenvalues and eigenfunctions is valid, and that the bounds to the eigenvalues can be estimated. The same is not all true with regard to non-self-adjoint problems. The eigenvalues are in general complex and may not always exist. The completeness of the eigenfunctions is questionable, and, consequently, ordinary proofs of the convergence of the iteration procedure requires re-examination. A simple estimation of the bounds of the eigenvalues is yet unknown.

It is beyond the scope of this book to discuss the mathematical problems connected with the non-self-adjoint equations in aeroelasticity. There exists an extensive mathematical literature, but simple and decisive theorems useful for practical calculations are few. It may be pointed out, however, that recent studies initiated by the flutter research have already yielded many significant results. Of greatest importance is Wielandt’s proof1124 that the classical iteration procedure can be used to find the eigenvalues (if they exist at all) and eigenfunctions of non-self-adjoint equations. Of practical methods of calculation, Lanczos’s “minimized iterations” method1113,11-14 is powerful and labor-saving, particularly when several eigenvalues and eigenfunctions are desired. Wielandt’s “iterative transformation” procedure11-24-11-26 is applicable to flutter and similar eigenvalue problems. (A partial but much more readable account of Wielandt’s method is given by Gossard11-9.) By a simple extension, Wi. elandt also gives a “broken (gebrochene) iteration” procedure11-24 which can be used to correct a given approximation for any arbitrary higher eigenvalue and the corresponding eigenfunctions without the knowledge of the preceding eigenvalues. Both Wielandt’s and Lanczos’s methods are applicable to algebraic (matrix), differential, or integral operators, and are of importance in studying the fundamental questions in aeroelasticity and in checking approximate solutions. On the other hand, solution of nonhomogeneous equations on the basis of expanding an arbitrary function in series of biorthogonal functions, the concept of “adjoint energy function,” and a variational principle which leads to a procedure of the Rayleigh-Ritz type have been introduced by Flax.7-1 A

method of calculating the eigenvalues of complex matrices is given by Wielandt,11-24 who gives also a simple algorithm to determine whether some of the eigenvalues have a positive imaginary part. A different form of the last mentioned generalization of Routh’s rules for discriminating the pseudo-negative roots of a polynomial with complex coefficients is given independently by Sherman, DiPaola, and Frissell.11-20,11-21

An aerodynamic operator relates the aerodynamic force acting on an airfoil and the motion of the airfoil. In aeroelasticity, the drag force and the skin friction being generally neglected, the most important aerody­namic operator is concerned with the aerodynamic lift and moment.

The complexity of the aerodynamic operator is the major difficulty in aeroelasticity. The explicit form of the aerodynamic operator, giving pressure distribution as a function of arbitrary wing deflection, is yet unknown even for such an idealized surface as an infinitely thin rectangular plate of finite span. It is here that a number of simplifying assumptions must be introduced. First, linearization of the hydrodynamic equations is imperative; hence, the theory will be applicable only to thin flat lifting surfaces. Second, it is necessary to tabulate the results numerically; hence, the form of the wing-deflection surface must be specified.

It is therefore evident why the method of generalized coordinates and the method of iteration are particularly suitable for aeroelastic problems. In each step of the iteration, as well as for each degree of freedom in generalized coordinates, the elastic deformation is known. Hence, the aerodynamic problem can be solved beforehand and the results tabulated.

It is also evident why the strip assumption on aerodynamic-force distribution across the span of a finite wing is so often made. When the strip assumption is used, it is necessary to tabulate only the two-dimen­sional flow cases. Without the strip assumption, it would be necessary to tabulate the results for every particular planform and every mode of motion of the wing.