Category Flight Vehicle Aerodynamics

The ideal gas law

p = pRT (1.7)

R = 287.04 J/kg-K (for air)

is an example of an equation of state, and is accurate for all common gases over a wide range of temperatures and pressures. The specific gas constant R is inversely proportional to the average molecular weight.

An additional important state variable is the specific internal energy e, which together with p and p also defines the specific enthalpy h.

(1.8)

(for ideal gas) (1.9)

For a thermally perfect gas, both e and h depend only on the temperature, and are respectively defined via the specific heat at constant volume cv (T), and the specific heat at constant pressure cp (T).

fT )

e(T) = eref + cv(T’) dT

(thermally perfect gas) (1.10)

h(T) href + °p(T/) dT

Tref

The reference values are arbitrary, since only changes in e and h are physically meaningful. The ideal-gas h definition (1.9) implies the following relation between the specific heats and the specific gas constant.

For a calorically perfect gas, both cv and cp are constant, which makes e and h directly proportional to T,

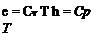(calorically perfect gas) (1.12)

where zero reference values have been chosen. Air at ordinary temperatures is very nearly calorically perfect. Hence, definitions (1.12) are appropriate for external aerodynamic flows, and here it is more natural to work directly with the enthalpy rather than the temperature. A more convenient form of the ideal gas law (1.7) is then given in terms of the enthalpy and the specific heat ratio 7.

YP Y	(Y -1)Ph	(1.13) (1.14)
(for air) (for air)
1.4 1004.6 J/kg-K
cp

In extreme conditions, such as those inside gas turbine hot sections, cv and cp can no longer be assumed to be independent of temperature, so the more general e(T) and h(T) definitions (1.10) must be used. Also, y then depends on temperature and thus has limited applicability. However, R is still nearly constant and the temperature form of the ideal gas law (1.7) still applies.

This chapter will describe the properties of atmospheric air, summarize key physical relations between these properties, and derive the equations of fluid motion which form the basis of aerodynamics.

1.1 Atmospheric Properties

A typical dimension t of any common aircraft is vastly greater than the molecular mean free path A of the air at any practical operating altitude, as quantified by the Knudsen number Kn = A/t ^ 1. Consequently the air can be considered to be a continuum fluid having a density p, pressure p, temperature T, and speed of sound a at every point in space and time. There are also viscous stresses and heat conduction at each point, which are quantified by the fluid’s viscosity p and heat conductivity k. The US Standard Atmosphere [1] has the following values for these properties for air at sea level.

Reference [1] also gives equations for these quantities at other altitudes, and tabulated values are also avail­able from many sources. Alternatively, the following curve-fit formulas for the pressure and temperature may be more convenient for numerical work, with the altitude z in kilometers and temperatures in Kelvin.

These approximations are accurate for z< 47 km, and are shown in Figure 1.1 for z< 26 km.

With p(z) and T(z) known, the atmospheric density p(z) can then be obtained from the ideal gas law (1.7), and the speed of sound can be obtained from expression (1.70) given in Section 1.7.3. The viscosity is accurately given by Sutherland’s Law with TS = 110 K for air,

which can also be used to relate the local viscosity to the local temperature at any point in a flow-field.

For gases, the heat conductivity k can be most easily obtained from the viscosity via the Prandtl number, Pr = cp p/k, which is very nearly constant across a wide range of temperatures. The specific heat cp will

be defined in the next section.

k = Op p/Pr (1.5)

Pr = 0.72 (for air) (1.6)

This book assumes that the reader is well versed in basic physics and vector calculus, and already has had exposure to basic fluid mechanics and aerodynamics. Hence, little or no space is devoted to introduction or discussion of basic concepts such as fluid velocity, density, pressure, viscosity, stress, etc. Chapter 1 on the Physics of Aerodynamics Flows is intentionally concise, since it is intended primarily as a reference for the underlying physical principles and governing equations of fluid flows rather than as a first introduction to these topics. The author’s course at MIT begins with Chapter 2.

Some familiarity with aerodynamics and aeronautics terminology is assumed on the part of the reader. How­ever, a summary of advanced vector calculus notation is given in Appendix A, since this is not commonly seen in basic vector calculus texts.

Acknowledgments

The author would like to thank Doug McLean, Alejandra Uranga, and Harold Youngren for their extensive comments, suggestions, and proofreading of this book. It has benefited considerably from their input. Ed Greitzer, and Bob Liebeck have also provided comments and useful feedback on earlier drafts, and helped steer the book towards its final form. Also very helpful have been the comments, suggestions, and error corrections from the numerous students who have taken the Flight Vehicle Aerodynamics course at MIT.

Mark Drela

Objective

This book is intended as a general reference for the physics, concepts, theories, and models underlying the discipline of aerodynamics. An overarching theme is the technique of velocity field representation and modeling via source and vorticity fields, and via their sheet, filament, or point-singularity idealizations. These models provide an intuitive feel for aerodynamic flow behavior, and are also the basis of aerodynamic force analysis, drag decomposition, flow interference estimation, wind tunnel corrections, computational methods, and many other important applications.

This book covers some topics in depth, while offering introductions or summaries of others. In particular, Chapters 3,4 on Boundary Layers, Chapter 7 on Unsteady Aerodynamics, and Chapter 9 on Flight Dynamics are intended as introductions and overviews of those topics, which deserve to be properly treated in separate dedicated texts. Similarly, there are only glancing mentions of the related topic of Propulsion, which is its own discipline.

Computational Fluid Dynamics (CFD) and computational methods in general are indispensable for today’s practicing aerodynamicist. Hence a few computational methods are described here, primarily the vortex lat­tice and panel methods which are based on the source and vorticity flow-field representation. The main goal is to provide improved understanding of the concepts and physical models which underlie such methods.

Most of this book is based on the lecture notes, handouts, and reference materials which have been devel­oped for the course Flight Vehicle Aerodynamics (course number 16.110) taught by the author at MIT’s Department of Aeronautics and Astronautics. This course is intended for first-year graduate students, but has also attracted a significant number of advanced undergraduates.