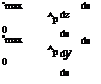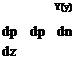Category Flight Vehicle Aerodynamics

For a general wake shape, the force integrals must be evaluated using numerical integration. A relatively sim­ple method is to discretize the wake into i = 1. ..N panels as shown in Figure 5.12, with each panel i hav­ing a length A Si, and a piecewise-constant potential jump Api, The sideforce and lift integrals (5.64),(5.65) then become sums over all the panels. The convenient panel inclination angle 9i is also introduced, so that

Ayi = cos 9i Asi and Azi = sin 9i Asi.

Figure 5.12: Wake paneling for evaluation of Trefftz-plane forces.

To evaluate the induced drag integral (5.47) it is necessary to first determine the normal velocity Vpi ■ ni at each panel midpoint. This is the velocity of all the trailing vortices resulting from the discrete steps in the potential jump. Referring to Figure 5.12, each trailing vortex strength is

ri — 1/2 — Api — 1 Api

defined positive about the x axis, or counterclockwise in the yz plane. The velocity at each panel midpoint is then the discrete counterpart of the 2D velocity superposition (5.38).

Its normal component can then be condensed into a convenient Aerodynamic Influence Coefficient (AIC) matrix Aij which depends only on the wake geometry,

and allows calculation of др/диг for any panel Aрг distribution by the simple summation. The induced drag integral (5.47) is then approximated by a second sum over the panels.

1 ^ д

Di = –p^^ALpi-^-Asi (5.82)

i= 1 г

5.8.1 Fourier series method for flat wake

The mathematical technique used in the lifting-line wing analysis described in Appendix E also provides a convenient means of computing lift and induced drag for the flat-wake case if we make the substitution Г ^ Ap. The potential jump is first expanded as a Fourier sine series in the angle coordinate d.

The Trefftz-plane lift integral (5.65) is seen to be the same as the lifting-line result (E.19), and depends only on the first coefficient Ai.

rb/2 і

L = A<pd у =

-b/2 2

The flat-wake normal velocity dp/dz defined by (5.41) is seen to be twice the lifting-line downwash wwake definition (E.2). This is evaluated in terms of the Fourier coefficients by result (E.10).

The flat-wake induced drag integral (5.48) can now be expressed in terms of the Fourier coefficients, by the lifting-line result (E.20).

The factor 1+5 in (5.71) is sometimes replaced by the inverse of the span efficiency, 1/e.

By choosing some suitable reference area Sref, with corresponding aspect ratio AR = b2/Sref, the above lift and induced drag can be put into convenient dimensionless forms.

For a given specified lift and span, the above results show that the minimum induced drag is obtained if 5 = 0, or e = 1, or equivalently A2 = A3 … = 0, and the potential jump has an elliptical distribution on the wake. For this case the normal velocity is also constant everywhere across the wake.

The induced drag definition (5.31) is awkward or impractical to evaluate as written because of the infinite double integral. It can be simplified considerably by using the identity

V/ – V/ = V-(/ V/) — / V2/ (5.42)

which is valid for any differentiable function /. Choosing / = p, which satisfies the continuity requirement V2p = 0, simplifies the identity to the following form.

Vp ■ Vp = V-(p Vp) (5.43)

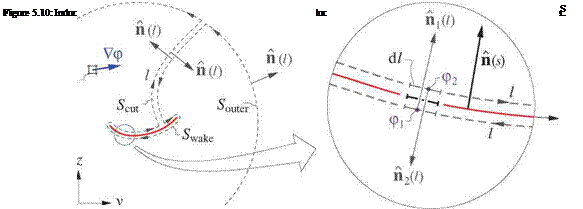
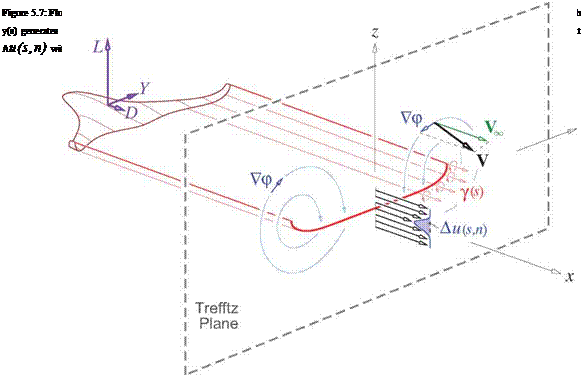
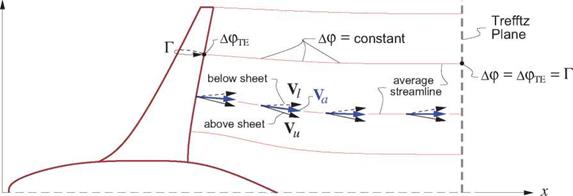
Referring to Figure 5.10, the induced drag integral (5.31) then becomes

A = JJ ^ pc* Ур • Ур dS = JJ ^ Pc* V • (p Vp) dS

= J ^ pVp ■ n dl (5.44)

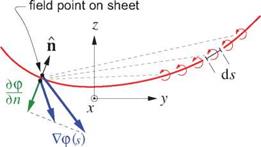
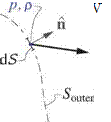
where the last step (5.44) was obtained via the Gauss Theorem. This integral is over the outer contour with arc length l, and its unit normal vector n points out of the domain.

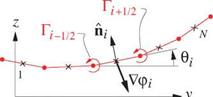
The outer contour must have p continuous inside, otherwise Gauss’s Theorem or identity (5.43) would not be valid. This requires placing the vortex sheet (and its Ap jump) topologically outside the contour, as shown in

Figure 5.10. For the three contour pieces Swake, Scut, Souter, the contribution of Souter to integral (5.44) will vanish when it’s taken out to infinity, and the contribution of piece Scut is always zero by antisymmetry of its two n vectors. For the only remaining piece Swake wrapped around the wake vortex sheet, the following relations hold for the two opposing points 1,2:

П i(i) = —П 2(1) = П (s) (5.45)

dl = ds (5.46)

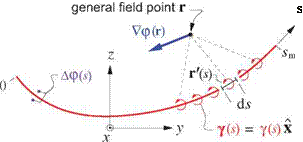
The Swake surface integral around the wake can now be replaced by an integral along the wake, and further simplified using the potential jump definition Ap = p2 — pi.

p Vp ■ П dl

wake

[ [ pi Vpi ■ n 1 + p2 Vp2 ■ П2 ] ds [ pi Vpi ■ n — P2 Vp2 ■ n ] ds

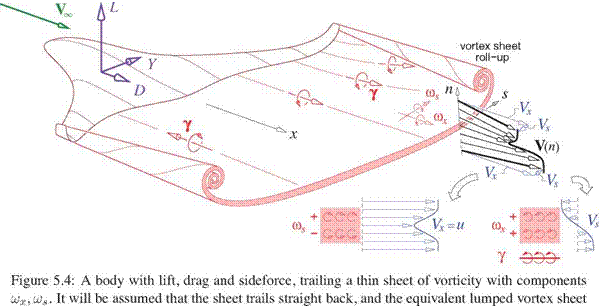
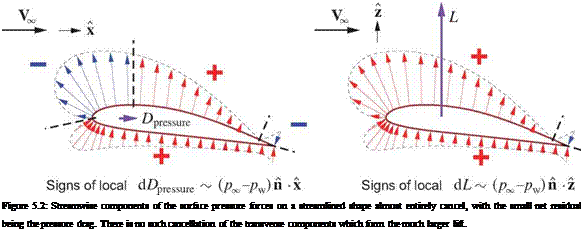
The profile drag expression (5.34) or (5.36) based on the momentum defect, together with the induced drag expression (5.47) based on the crossflow kinetic energy, provide a relatively simple and quite accurate means of calculating or estimating the overall drag of a general 3D configuration.

The required input for the profile drag expression is the spanwise momentum defect distribution P(s). The input for the induced drag expression is the wake sheet potential jump distribution Ap(s). Both of these can be obtained with a suitable combination of inviscid and boundary layer calculation methods, preferably coupled via one of the displacement-effect models. The overall far-field drag is relatively accurate, since it does not suffer from pressure drag cancellation errors which make near-field pressure drag calculation unreliable.

The first righthand side term in the identity (5.57) vanished inside the contour integrals via Gauss’s Theorem and the div(curl) = 0 identity.

£ [Vx(flv)| ■ n dS = V-[Vx(av)]dV = 0 dV = 0

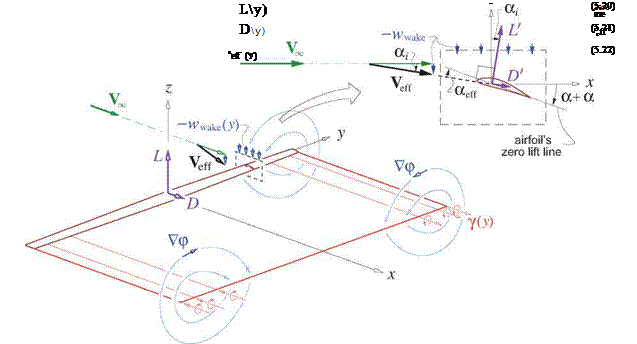
For the final step in simplifying the sideforce and lift expressions (5.58), (5.59) we note that their integrands are nonzero only on the Trefftz plane where the trailing vorticity и exits the control volume. Here we have П = X, so that и ■ П = wx where the scalar шх is the streamwise vorticity component along x. As in the drag derivations, we now also make the assumption that the viscous wake is thin, so that the area element can be recast using the sheet’s coordinates, dS = dn ds, which permits lumping of the vorticity шх into the equivalent vortex sheet strength 7 = J шх dn.

I Smax

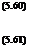Y = P^C —zwx dn ds = pTOL -27 ds

JJtp J 0

l Smax

L = PlL ушх dn ds = pTOL VY ds

TP 0

Next, using relation (5.37), the vortex sheet strength is replaced by the potential-jump derivative, and the resulting expressions are integrated by parts.

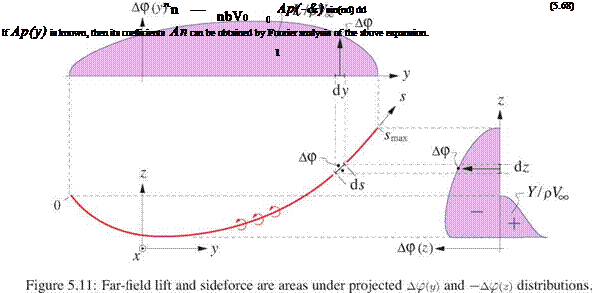
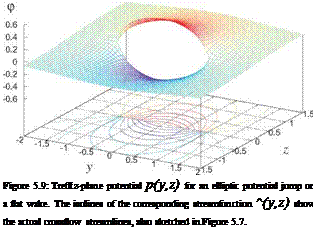
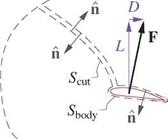
Since the potential jump is zero at each end of the sheet, the first term in (5.62) and (5.63) disappears. The final expressions for the far-field sideforce and lift are then simple integrals of the potential jump or jumps over the — z and у projections of the vortex sheet, as shown in Figure 5.11.

For this reason, the wake potential jump Ap is frequently called “the loading," since the quantity pL Vl Ap is in effect the load/span acting on the body which is shedding the wake, and acts normal to the wake.

The profile drag (5.32) can be rewritten as

Dp = Ц pu (VA – u) dS

5.6.1

Trefftz-plane velocities

As stated earlier, the perturbation potential velocity Vp is associated with the wake vortex sheet strength 7 = y X. Based on the equivalence between vortex and doublet sheets presented in Section 2.5, this 7(s) is related to the sheet’s potential jump Ap(s) as follows.

Referring to Figure 5.8, this vortex sheet defines the 2D perturbation velocity field Vp in the Trefftz plane, via the usual 2D superposition integral,

where r is the yz field point, and r'(s) = y'(s) y + z'(s) Z parametrically defines the shape of the sheet.

Figure 5.8: Vortex sheet in Trefftz plane, with associated perturbation velocity field Vp, which has normal velocity component dp/dn on the sheet itself.

For the particular field point locations on the sheet itself, we can also define the normal component of this velocity.

One of the two velocities (or their average) on either side of the sheet can be used here, since they have the same normal component, which is continuous across any vortex sheet.

Many applications consider the simpler situation of a flat wing of span b, where the vortex sheet is also flat and lies on the y axis from —b/2 to b/2. The above relations then simplify as follows.

The potential field p(y, z) for this flat-wake case, with an elliptic potential jump Ap = /l — (2y/b)2, is shown in Figure 5.9. The corresponding streamfunction shows the crossflow streamlines.

The exact far-field drag is defined as the streamwise component of the total far-field force (5.13).

D = F ■ X = S [ (Po – p) n ■ x – p(V ■ n)(n – Vo) ] dS (5.26)

outer

Appendix C evaluates the 2D form of this relation by integrating around a circular contour far from the airfoil, using potential 2D far-field approximations for p and V, with a special treatment of the viscous wake velocity defect. For a lifting 3D body, the integrand in (5.26) is negligible everywhere except in the downstream Trefftz-plane part of Souter, where the trailing wake exits. Hence, for 3D cases the drag integral above can be conveniently restricted to only this yz Trefftz plane, which has П = X.

D = [ pTO – p – pu(u – Vco)]dy dz (5.27)

TP

We will now decompose the effectively-exact far-field drag expression (5.27) into profile and induced com­ponents. This requires an idealization of the flow in the Trefftz plane, as sketched in Figure 5.7. Specifically, the vortex sheet thickness is assumed to be small compared to its span, and its net strength y(s) is assumed to be in the x direction. The wake roll-up will also be neglected to simplify the sheet’s yz shape. The roll-up issue in drag calculations is discussed in more detail by Kroo [47].

As shown in Figure 5.7, the total velocity at the Trefftz plane is broken down into the freestream VOX, a potential perturbation velocity Vp associated with the streamwise vorticity 7(s), and a streamwise viscous defect An associated with the transverse vorticity (shown in Figure 5.4) inside the viscous wake.

V = (Vo + Au)X + Vp (5.28)

The pressure is related to the potential part of the velocity (excludes An) by the incompressible Bernoulli equation (1.109). This is valid since Trefftz-plane velocities are typically low even for high-speed vehicles.

P = Pc* + pc^V^ – p^ |KoX + V<p|2

= Pc* – Poo Ко Px – 3P00 {pi + Py + pi) (5.29)

Substituting the velocity (5.28) and pressure (5.29) expressions into the drag integral (5.27) and simplifying the result produces a natural decomposition of the drag into its induced-drag and profile-drag components.

The induced drag expression (5.31) is seen to be the crossflow kinetic energy (per unit distance) deposited by the body. This energy is provided by part of the body’s propelling force working against the induced drag. The remaining part works against the profile drag, considered next.

A practical approach to lift and drag calculation for high aspect ratio 3D wings is based on the lifting line approximation. This is actually a hybrid far-field/near-field model which uses the idealized straight-wake approximation together with specified spanwise wing chord and twist distributions c(y) and aaero(y). The lifting-line model hinges on the following assumptions, illustrated in Figure 5.6.

• The near-field flow is 2D, but with an effective freestream velocity Veff. This is the freestream plus the velocity of wake, so that it contains all contributions except those of the vortices and sources representing the local 2D wing section. These are intentionally not shown in Figure 5.6, since they do not contribute to Veff. The wake vorticity lies mostly along the freestream, so its downwash velocity wwake at the wing is in the vertical z direction, and therefore acts primarily to tilt the freestream by

For a positive (upward) lift, the downwash is typically negative and hence a is positive.

• At each spanwise location, wwake is assumed to be nearly uniform over the chordwise extent of the wing’s airfoil, so that a acts to modify the airfoil’s angle of attack at that location.

• At each spanwise location y, wwake(y) is related to the wake vortex sheet strength 7 at all spanwise locations y’ by considering the wake to be a row of semi-infinite vortex filaments of strength j(y’) dy’. The vertical velocity at у, of a filament at y’, is then dwwake = 7 dy’/4n(y—y’). Integrating this over the span y = — b/2 … b/2 gives the overall local downwash.

dp 1 fb/2 Y(y’)

-^(0,y,0) = Wwake(y) = — – jdy’ (5.19)

dz 4n – b/2 y—y’

The physical lifting-line model described here does not require that the wing be unswept. However, classical wing analysis methods based on the lifting-line model, like the one given in Appendix E, do assume zero sweep. For simplicity, the rest of this section will also assume zero sweep.

A very attractive feature of the lifting-line approximation is its ability to exploit known airfoil characteristics. Because the near-field flow is effectively 2D, its lift/span and drag/span are described by the usual 2D airfoil coefficients в£, Cd corresponding to the wing-section airfoil shape at that location.

Here aeff is the effective local angle of attack, which is less than the overall aerodynamic angle a+aaero(y) because of the induced angle ai(y). The induced angle also rotates the local lift and drag forces L’, D’ as indicated in Figure 5.6. Projecting L’, D’ onto the global freestream-aligned z, x axes and integrating over the span gives the overall lift and drag L, D.

The rightmost (approximate) forms of the L, D expressions assume

ai 1 , cd c£

which is valid for high aspect ratio wings with unstalled airfoils.

Although the above lifting-line analysis has defined the overall wing lift and the wing drag components in terms of the relevant quantities, it is not fully predictive as given. Actual calculation of the forces would require knowledge of the vortex sheet strength distribution y(y) as a starting point. This is determined from the wing geometry using the classical procedures given in Appendix E.

The lifting-line force analysis summarized by equations (5.23) and (5.24) is seen to naturally produce a breakdown of the total drag D into profile drag and induced drag components Dp and Di, and gives them in terms of the sectional D’, L’ forces and the induced angle ai by relations (5.25). The main limitation of this analysis is that it assumes a high aspect ratio wing. For low aspect ratio wings, or more general lifting body shapes like the one shown in Figure 5.4, the vertical velocity wwake of the vortex wake cannot be assumed to be uniform across the chord. In this case a locally-constant induced angle ai and hence a local effective angle of attack aeff(y) cannot be defined, so that the lift and drag coefficient cg and Cd in (5.20) and (5.21) cannot be uniquely determined. Furthermore, with low aspect ratios the locally-2D flow assumption becomes invalid, so that the 2D coefficients cg and Cd would become questionable even if some suitable chord-averaged aeff could be assumed. The overall consequence is that lift and drag expressions (5.23), (5.24) become inapplicable for general body geometries. These limitations will be avoided with the more general far-field force analyses considered in the subsequent sections.

The idealized far-field force components will be seen to depend on the potential jump Ap(s) across the ideal­ized wake sheet in the far-downstream Trefftzplane. The objective here is to relate Ap(s) to the aerodynamic parameters of the lifting body which generated the sheet.

A thin wake layer must have a zero static pressure jump Ap = pi — pu across it. Assuming the total pressure is the same on the upper and lower sides of the wake vortex sheet, the compressible or incompressible steady Bernoulli equation then implies a zero jump in the velocity magnitude. We then have,

0 0 0

0 (5.15)

V0 = i(V„+V/)

AV = Vu—Vi

where V denotes the average sheet velocity, shown in Figure 5.5. In the final relation (5.15), the velocity jump AV was replaced by the equivalent surface gradient of the potential jump Ap.

Equation (5.15) states that the gradient of the sheet potential jump Ap(s, e) is perpendicular to Va, or equiv­alently that Ap(s, e) is constant along the average sheet streamlines which are everywhere parallel to Va, as shown in Figure 5.5. Hence, the ApTE value at the body’s trailing edge where the sheet is shed persists downstream along the average streamline into the Trefftz plane. This ApTE is also the circulation Г about a contour around the wing or body section which contains that trailing edge point.

In principle one could trace the average wake streamlines from the wing trailing edge to determine its shape y(s),z(s) and loading Ap(s) in the far-downstream Trefftz plane. This would require tracking the roll-up process in detail which for most cases is impractical. A more common and much simpler approach is to trace the wake streamlines straight back along the freestream direction. This is equivalent to assuming

Va ~ Vo (straight-wake assumption) (5.16)

which is the model shown in Figures 5.6 and 5.7 in the following sections.

The objective now will be to simplify the lift, drag, and sideforce components of the far-field force expres­sion (5.13) into forms which involve only the wake trailing from the lifting body. This will provide physical insight into the links between forces and flow-field properties, and will also result in practical calculation methods for the forces which will enhance aerodynamic configuration design and optimization procedures.

Consider the rather complicated but typical vortex wake shed by an aerodynamic body, sketched in Fig­ure 5.4. The following simplifications and idealizations of the wake vortex sheet will be made:

• The wake vortex sheet is assumed to trail straight back from the trailing edge where it is shed, along the freestream direction (i. e. along the x-axis). The yz cross-sectional shapes of the sheet will therefore be the yz-shape of the wing trailing edge. In effect this neglects the roll-up of the vortex sheet which typically begins at the sheet edges and eventually involves the entire sheet. The straight-wake assumption will be modified slightly in Section 5.9 where a fuselage affects the wake trajectory.

• Only the streamwise vorticity wx is assumed to have a nonzero lumped vortex sheet strength 7. This will be used to construct the perturbation velocity field Vp outside of the sheet, which is associated with the lift, sideforce, and induced drag. The transverse vorticity ws is associated with the viscous velocity defect within the sheet which determines the remaining profile drag component.

Consider the indented control volume shown in Figure 5.3, which has the body outside of it topologically. Since the volume is empty, the integral momentum equation (1.28) can be applied to it. Assuming steady flow, and eliminating the gravity force pf by re-defining p to exclude the hydrostatic pressure, we have

where the contour integral can be broken up into three Sbody, Souter, and Scut pieces indicated in Figure 5.3. §[]dS = § [ ]dS + § [ ]dS + []dS = 0

body outer cut

Since the two parts of Scut have equal and opposite П vectors its contribution vanishes.

[]dS = 0 (5.11)

cut

Furthermore, Sbody is defined to lie on the body’s solid surface where V ■ П = 0, so that the Sbody integral upon comparison with (5.1)-(5.3) is seen to be the body force F.

§ [ p(V■ n)V + pn – T ■ n ] dS = § [ Pwn – rw ] dS = F (5.12)

body body

The integrand above differs in sign from (5.1)-(5.3) because here n is in the opposite direction, as can be seen by comparing Figures 5.1 and 5.3.

Combining (5.11) and (5.12) with (5.10) then gives an expression for the body force which involves only the Souter contour. This is known as the Integral momentum theorem.

Figure 5.3: Body force F is related to quantities on any contour Souter enclosing the body. Third dimension y and sideforce Y are not shown.

The viscous stress r ■ n was assumed to be negligible on Souter and has been left out of (5.13), although it could be retained if appropriate. As before, — p was replaced with p»—p in (5.13) with no effect. Also, V was replaced with V—V» which is allowed because of the mass conservation integral for the volume.

S P(V■ П) dS = 0 (5.14)

outer

The integral (5.13) gives an alternative way to calculate the force F on the body, using flow quantities on any surface Souter surrounding the body. Although it’s called the “far-field force." the integral is valid for any distance of the Souter contour, provided only that r ■ П on it is negligible as assumed. A distant placement is required only if far-field models are used to estimate the p and V needed to evaluate the integral.

In grid-based CFD solutions of the Navier-Stokes equations, the D, Y, L force components are calculated directly from the above definitions via numerical integration over the surface. But in simplified flow models, such as inviscid panel methods with or without viscous displacement models, this approach works only for the transverse Y, L components. If the streamwise D component were computed in this manner, it would be simply incorrect or very inaccurate. The main reason is that the Dpressure integral has relatively large positive and negative integrand contributions over the surface which mostly cancel, as shown in Figure 5.2.

In fact, for 2D inviscid flow the cancellation is theoretically perfect, which constitutes d’Alembert’s paradox.

D = Dpressure = 0 (2D inviscid flow) (5.9)

For 3D inviscid lifting flows the cancellation is not total, but is still extensive so that the pressure drag force is small. Even small errors in the surface pressures, or in the discrete integration method, then become very large when compared against the small (but crucial) remaining pressure drag.

The pressure-drag calculation difficulties remain for viscous flow simulations which employ the displace­ment effect. Such methods were discussed in Chapters 3 and 4, and two computed examples are shown in Figures 3.6 and 3.7. The modification of pw by the viscous displacement has the following effects:

• The modified lift L is now much more accurate than what’s predicted by the simple inviscid model. In particular, it can capture the effects of flow separation and stall as was shown in Figure 3.5.

• The modified Dpressure is now correctly nonzero in 2D flow. However, its accuracy is still relatively poor due to the streamwise-component pressure force cancellation shown in Figure 5.2. One reason is that errors associated with numerical integration over the discretized surface are still present. This is most severe for simple panel methods with relatively coarse paneling. Another reason is that even with the displacement effect corrections, the computed wall pressures pw still have residual errors. These may be small relative to lift, but are very significant relative to the much smaller pressure drag. These errors in pw are caused by the neglect of streamwise flow curvature in the viscous displacement-effect models, and are not easily removed.