Category MECHANICS. OF FLIGHT

The pressure in a stationary fluid increases with depth. This variation is rather complicated in the case of air because the density also increases with depth. These variations are very important for aircraft flight and in the next chapter the changes of pressure, density and temperature with height are described in some detail.

In a liquid, matters are much simpler; the density remains almost constant, and the pressure change is directly proportional to the change in depth. In a liquid, the pressure variation is given by the simple expression:

Change in pressure = density X gravity constant X change in depth

Or: Ap = p X g X (change in depth)

This book is about the mechanics of aircraft flight, so you might wonder why we should have any interest in the way that pressure varies in a liquid. The answer is that it is because the easiest way to measure pressure is to use a U – shaped tube containing liquid. This is known as a U-tube manometer, and is described in the next chapter.

Density is defined as the mass per unit volume of a substance, so it has the units ofkg/m3 in the SI metric system. Notice that it is mass and not weight that is used in this definition. The symbol commonly used for density is the Greek letter p.

As we will see later, the density of air changes with height and with the weather conditions. The density of water is conveniently 1000 kg/m3, and hardly varies at all, even if subjected to a very high pressure.

ІЛЛ

Fig 1.8 Force due to pressure acts at right angles to the surface

In the mechanics of flight we shall be chiefly concerned with fluid pressure, that is, the pressure in a liquid or gas, and the force that it produces. The reason why a fluid exerts a force is because its molecules are in rapid motion and bombard any surface that is placed in the fluid; each molecule exerts only a tiny force on the surface but the combined effect of the bombardment of mil­lions upon millions of molecules results in an evenly distributed force on the surface.

Pressure is a scalar quantity. That means that it has a magnitude, but unlike a vector, there is no direction involved. When a fluid at rest is in contact with

a surface, the pressure produces a force which acts at right angles to the surface (Fig. 1.8). Note that the force does have a direction whereas the pressure that causes it does not. It is not surprising that people often confuse cause and effect when talking about pressure. You will find many old books that say that pressure acts equally in all directions. It does not. Pressure does not act in any direction; it is the force due to pressure that acts in a direction. The direction of the force is always at right angles to the surface that the pressure is exposed to. We measure pressure in terms of the force that it will produce on an area, so pressure has the units of newtons per square metre (N/m2).

A pressure of 1 N/m2 is also called a pascal (Pa). Another common metric unit of pressure is the millibar (mb), which is 1/1000 th of a bar: a bar being 105 N/m2. This seemingly odd unit comes about, because 1 bar is very close to the standard atmospheric pressure at sea level. It had been adopted by meteo­rologists many years before the metric SI units were introduced, and the reader may often encounter atmospheric pressure given in millibars, particularly in flying manuals. In this book, we will use millibars for atmospheric pressure when appropriate, as in dealing with the effects of altitude. However, for most straightforward aerodynamic calculations we will use N/m2 since this is normal practice in European educational institutions.

Let us be sure that we understand the differences between energy and momentum, because we shall be concerned with this later on.

Energy is 1/2 mv2. Momentum is mv.

So the mass of 2 kg, moving at 10 m/s, has 100 units of energy (joules), but 2 X 10, i. e. 20 units, of momentum (kg X m/s).

Yes, but there is more to it than that.

Consider two bodies colliding, e. g. billiard balls.

The total momentum after the collision is the same as the total momentum before; the momentum lost by one ball is exactly the same as the momentum gained by the other. This is the principle of the conservation of momentum. (In considering this it must be remembered that momentum has direction, because velocity has direction.) The law will apply whether the balls rebound, or whether they stick together, or whatever they do.

But the total mechanical energy after the collision will not be the same as before; energy will be dissipated, it will go into the air in the form of heat, sound, etc.; the total energy of the universe will not be changed by the colli­sion – but that of the balls will be.

So momentum is a more permanent property than energy, the latter is often wasted and we shall sometimes find it unfortunate that in order to give a body momentum we must also give it energy.

A knowledge of the principles of mechanics – and particularly of the signifi­cance and meaning of the force of gravity, of accelerated motion, of centripetal and centrifugal force and motion on curved paths – will help us to understand the movements and manoeuvres of an aeroplane. This knowledge will also help us to understand the movement of satellites and spacecraft.

Work, power and energy [2] 2 heat, light, sound, electrical, chemical, magnetic, atomic – and, most useful of all, mechanical. A little thought will convince us how much of our time and energy is spent in converting, or trying to convert, other forms of energy into mechanical energy, the eventual form which enables us to get somewhere. The human body is simply a form of engine – not a simple form of engine – in which the energy contained in food is converted into useful, or useless, work. Unfortunately there is a tendency for energy to slip back again, we might almost say deteriorate, into other forms, and our efforts to produce mechan­ical energy are not always very efficient.

Even mechanical energy can exist in more than one form; a weight that is high up can do work in descending, and it is said to possess potential energy or energy of position; a mass that is moving rapidly can do work in coming to rest, and it is therefore said to have kinetic energy or energy of motion; a spring that is wound up, a gas that is compressed, even an elastic material that is stretched, all can do work in regaining their original state, and all possess energy which is in a sense potential but which is given various names according to its application.

In figures, a weight of 50 newtons raised to a height of 2 metres above its base has 100 joules of potential energy or, to be more correct, it has 100 joules more potential energy than it had when at its base. This was the work done to raise it to the new position, and it is the work that it should be able to do in returning to its base.

In symbols, W newtons at height b metres has – Wb joules of energy.

What is the kinetic energy of mass of m kg moving at z’m/s?

We don’t know, of course, how it got its kinetic energy, but the actual process is unimportant, so let us suppose that it was accelerated uniformly at a metres per second per second from zero velocity to v metres per second by being pushed by a constant force of F newtons.

If the distance travelled during the acceleration was s metres, then the work done, i. e. its kinetic energy, will be Fs joules.

But Vі = u1 + las (and и = 0)

.’. Vі = las s = VіЦ a

But F = та

So K. E. = Fs = та X iP-Ha = тіР joules.

Fig 1В Power (opposite) (By courtesy of the Lockheed Aircraft Corporation, USA)

The Lockheed C-5 Galaxy with four turbofan engines, each of 183 kN thrust represents a total power of 183 000 kW at the maximum level speed of about 900 km/h.

Thus the kinetic energy of 2 kg moving at lOm/s

1 О

= – mvL = tX2X 102 = 100 joules

We have managed to arrive so far without mentioning the term centrifugal force. This is rather curious because centrifugal force is a term in everyday use, while centripetal force is hardly known except to the student of mechanics.

Consider again the stone rotating, on a table, in a horizontal circle. We have established the fact that there is an inward force on the stone, exerted by the string, for the set purpose of providing the acceleration towards the centre – yes, centripetal force, however unknown it may be, is a real, practical, physical force. But is there also an outward force?

The situation is similar to that of the accelerating aircraft towing a glider that we described earlier. There is an outward reaction force on the outer end of the string caused by the fact that it is accelerating the stone inwards: an inertia force, and we could call this a centrifugal reaction force. This keeps the string in tension in a state of equilibrium just as if it were tied to a wall and pulled. Note, however, that there is no outward force on the stone, only an inward one applied by the string to produce the centripetal acceleration. As with the accelerating glider described previously and shown in Fig. 1.2, the forces on the two ends of the string are in balance, but the forces on the object, the stone or the glider are not, and hence acceleration occurs.

The concept of inertia forces is a difficult one. In a free-body diagram of the horizontally whirling stone, the only externally applied horizontal force is the inward force applied by the string. This force provides the necessary accelera­tion. There may be outward forces on the internal components of the system like the string, but not on the overall system. Note that if you let go of the string, the stone will not fly outwards, it will fly off at a tangent.

To sum up motion on curved paths. There is an acceleration (v2/r) towards the centre, necessitating a centripetal force of miP-lr.

At this stage, the reader is advised to try some numerical questions on motion on curved paths in Appendix 3.

We all know the direction of the force as a result of practical experience. Swing a stone round on the end of a piece of string. In what direction does the string pull on the stone to keep it on its circular path? Why, towards the centre of the circle, of course, and since force and acceleration are in the same direction, the acceleration must also be towards the centre.

We know too that the greater the velocity of the stone, and the smaller the radius of the circle on which it travels, the greater is the pull in the string, and therefore the greater the acceleration. The acceleration is actually given by the simple formula v2lr, where v is the velocity of the body and r the radius of the circle.

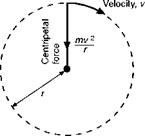
The force towards the centre is called centripetal force (centre-seeking force), and will be equal to the mass of the body X the centripetal accelera­tion, i. e. to m X v2lr (Fig. 1.4).

We have made no attempt to prove that the acceleration is v2lr – the proof will be found in any textbook on mechanics – but since it is not easy to con­ceive of an acceleration towards the centre as so many metres per second per second when the body never gets any nearer to the centre, it may help if we

Fig 1.4 Centripetal force

translate the algebraic expression into some actual figures. Taking the simple example of a stone on the end of a piece of string, if the stone is whirled round so as to make one revolution per second, and the length of the string is 1 metre, the distance travelled by the stone per second will be 2ттr, i. e. 2-тт X 1 or 6.28 m. Therefore

v = 6.28 m/s, r = 1 m

.’. acceleration towards centre = v2lr

= (6.28 X 6.28)/l = 39.5 m/s2 (approx)

Notice that this is nearly four times the acceleration of gravity, or nearly 4g. Since we are only using this example as an illustration of principles, let us sim­plify matters by assuming that the answer is 4g, i. e. 39.24 m/s2.

This means that the velocity of the stone towards the centre is changing at a rate 4 times as great as that of a falling body. Yet it never gets any nearer to the centre! No, but what would have happened to the stone if it had not been attached to the string? It would have obeyed the tendency to go straight on, and in so doing would have departed farther and farther from the centre.

What centripetal force will be required to produce this acceleration of 4g? The mass of the stone X 4g.

So, if the mass is 1/2 kg, the centripetal force will be 1/2 X 4g = 2 X 9.81 = 19.62, say 20 newtons.

Therefore the pull in the string is 20 N in order to give the mass of 1/2 kg an acceleration of 4g.

Notice that the force is 20 newtons, the acceleration is 4 g. There is a hor­rible tendency to talk about ‘g’ as if it were a force; it is not, it is an acceleration.

Now this is all very easy provided the centripetal force is the only force acting upon the mass of the stone. However, in reality there must be a force of gravity acting upon it.

If the stone is rotating in a horizontal circle its weight will act at right angles to the pull in the string, and so will not affect the centripetal force. But of course a stone cannot rotate in a horizontal circle, with the string also hori­zontal, unless there is something to support it. So let us imagine the mass to be on a table – but it will have to be a smooth, frictionless table or we shall introduce yet more forces. We now have the simple state of affairs illustrated in Fig. 1.5.

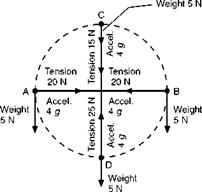
Now suppose that we rotate the stone in a vertical circle, like an aeroplane looping the loop, the situation is rather different (Fig. 1.6). Even if the stone were not rotating, but just hanging on the end of the string, there would be a tension in the string, due to its weight, and this as near as matters would be very roughly 5 newtons, for a mass of 1/2 kg. If it must rotate with an accel­eration of 4g the string must also provide a centripetal force of 20 newtons. So when the stone is at the bottom of the circle, D, the total pull in the string will be 25 N. When the stone is in the top position, C, its own weight will act towards the centre and this will provide 5 N, so the string need only pull with an additional 15 N to produce the total of 20 N for the acceleration of 4g. At the side positions, A and B, the weight of the stone acts at right angles to the string and the pull in the string will be 20 N.

To sum up: the pull in the string varies between 15 N and 25 N, but the acceleration is all the time 4g and, of course, the centripetal force is all the time —20 N. From the practical point of view, what matters most is the pull in the string, which is obviously most likely to break when the stone is in position D and the tension is at the maximum value of 25 N.

To complicate the issue somewhat, suppose the stone rotates in a horizontal circle, but relies on the pull of the string to hold it up (Fig. 1.7), and that the string has been lengthened so that the radius on which the stone is rotating is

Direction of rotation

Fig 1.5 Stone rotating in a horizontal circle, supported on a table

Fig 1.6 Stone rotating in a vertical circle

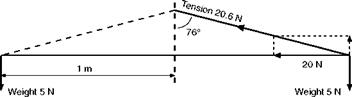
still 1 metre. The string cannot of course be horizontal since the pull in it must do two things – support the weight of the stone and provide the centripetal force.

Here we must introduce a new principle.

A force of 5 N, vertically, is required to support the weight.

A force of 20 N, horizontally, is required to provide the centripetal force. Now five plus twenty does not always make twenty-five! It does not in this example, and for the simple reason that they are not pulling in the same direc­tion. We must therefore represent them by vectors (Fig. 1.7), and the diagonal will represent the total force which, by Pythagoras’ Theorem, will be

V(202 + 52) = V425 = 20.6 N

The tangent of the angle of the string to the vertical will be 20/5 = 4.0. So the angle will be approx 76°. Expressing the angle, 0, in symbols –

Centripetal force

tan 0 = . = (m X v2/r)IW

Weight

Fig 1.7 Stone rotating in a horizontal circle, with string support

(mg being the weight expressed in newtons)

= v[1]lrg

This angle 0 represents the correct angle of bank for any vehicle, whether it be bicycle, car or aeroplane, to turn a corner of radius r metres, at velocity v metres per second, if there is to be no tendency to slip inwards or to skid out­wards.

It will help us in working examples if we summarise the relations which apply in kinematics, that is, the study of the movement of bodies irrespective of the forces acting upon them.

We shall consider only the two simple cases, those of uniform velocity and uniform acceleration.

Symbols and units will be as follows –

Time = t (sec)

Distance = s (metres)

Velocity (initial) = и (metres per sec) Velocity (final) = v (metres per sec)

Acceleration = a (metres per sec per sec)

Uniform velocity

If velocity is uniform at и metres per sec clearly

Distance travelled = Velocity X Time
or s = ut

Uniform acceleration

Final velocity = Initial velocity + Increase of velocity or v = и + at

Distance travelled = Initial velocity X Time

+ t Acceleration X Time squared

i. e. s = ut + at2

Final velocity squared = Initial velocity squared

+ 2 X Acceleration X Distance

or Vі = ur + las

With the aid of these simple formulae – all of which are founded on first prin­ciples – it is easy to work out problems of uniform velocity or uniform acceleration (see Examples 1.2 to 1.4).

EXAMPLE 1.2

If, during a take-off run an aeroplane starting from rest attains a velocity of 90 km/h in 10 seconds, what is the average acceleration?

SOLUTION Initial velocity и = 0 Final velocity v = 90 km/h = 25 m/s Time t = 10 sec a = ?

Since we are concerned with u, v, t and a, we use the formula v = и + at 25 =0 + 10d a = 25/10 = 2.5 m/s2

EXAMPLE 1.3

How far will the aeroplane of the previous example have travelled during the take-off run?

SOLUTION

и = 0, v = 25 m/s, t = 10 sec, a = 2.5 m/s2 To find s, we can either use the formula Final velocity squared = Initial velocity squared

+ 2 X Acceleration X Distance

s = ut + at2 = 0 + у X 2.5 X 102

= 125 m

or у1 = и2 + las 25 X 25 = 0 + 2 X 2.5 X s :.s = (25 X 15)1(1 X 2.5)

= 125 m

EXAMPLE 1.4

A bomb is dropped from an aeroplane which is in level flight at 200 knots at a height of 3500 m. Neglecting the effect of air resistance, how long will it be before the bomb strikes the ground, and how far horizontally before the target must the bomb be released?

SOLUTION

To find the time of fall we are concerned only with the vertical velocity, which was zero at release, с. и = 0

a = acceleration of gravity = 9.81 m/s2 s = vertical distance from aeroplane to ground = 3500 m t = ?

We need the formula connecting u, a, s and t, i. e. s = ut + at2

3500 = 0 + і X 9.81 X t2

t2 = (3500/9.81) X 2 = 713 .’. t = 27 sec (approx)

Since we are neglecting the effect of air resistance, the horizontal velocity of the bomb will, throughout the fall, remain the same as it was at the moment of release, i. e. the same as the velocity of the aeroplane, namely 200 knots or, converting into metres per second, (200 X 1852)73600 = 103 m/s (approx).

Therefore the distance that the bomb will travel forward during the falling time of 27 s will be 103 X 27 = 2781 m.

This, of course, is the distance before the target that the bomb must be released.

Note that in Example 1.4 we have neglected air resistance. Since we are interested in flying this may seem rather a silly thing to do, because we are only able to fly by making use of the same principles that are responsible for air resistance. In fact, too, the effects of air resistance on bombs are of vital importance and are always taken into account when bombing. But it is better to learn things in their most simple form first, then gradually to add the com­plications. As these complications are added we get nearer and nearer to the truth, but if we are faced with them all at once the picture becomes blurred and the fundamental principles involved fail to stand out clearly.

Other examples on kinematics will be found in Appendix 3, and the reader who is not familiar with examples of this type is advised to work through them.

Motion on curved paths

It has already been emphasised that bodies tend to continue in the same state of motion, and that this involves direction as well as speed. It is clear, there­fore, that if we wish to make a body change its motion by turning a comer or travelling on a curved path, we must apply a force to it in order to make it do so, and that this will apply even if the speed of the body does not change. This is a force exactly similar to the one that is required to accelerate an aircraft, that is to say: the force must be proportional to the mass of the body and to the acceleration which it is desired to produce. But what is the acceleration of a body that is going round a corner? Is there, in fact, any acceleration at all if the speed remains constant? And in what direction is the acceleration?

Let us deal with the last question first. There is another part of Newton’s second law which has not so far been mentioned, namely that the rate of change of momentum of the body will be in the direction of the applied force. If the mass of the body does not change as it goes round the corner the accel­eration must be in the direction of the force. But is there any acceleration if the speed does not change? Yes – because velocity is what we call a vector quan­tity, that is to say, it has both magnitude and direction, while speed has only magnitude. Thus if the direction of motion changes, the velocity changes even though the speed remains unaltered. But at what rate does the velocity change? – in other words, what is the acceleration? and in what direction is it?

The mass of a body depends on the amount of matter in it, and it will not vary with its position on the earth, nor will it be any different if we place it on the moon. The weight (the force due to gravity) will change, however, because the so-called gravity constant will be different on the moon, due to the smaller mass of the moon, and will even vary slightly between different points on the earth. Also, therefore, the rate at which a falling object accelerates will be dif­ferent. On the moon it will fall noticeably slower, as can be observed in the apparently slow-motion moon-walking antics of the Apollo astronauts.

Units

The system of units that we use to measure quantities, feet, metres, etc., can be a great source of confusion. In European educational establishments and most of its industry, a special form of the metric system known as the Systeme International or SI is now in general use. The basic units of this system are the kilogram for mass (not weight) (kg), the metre for distance (m) and the second for time (s).

Temperatures are in degrees Celsius (or Centigrade) (°С) when measured relative to the freezing point of water, or in Kelvin (K) when measured relative to absolute zero; 0°C is equivalent to 273 K. A temperature change of one degree Centigrade is exactly the same as a change of one degree Kelvin, it is just the starting or zero point that is different. Note that the degree symbol ° is not used when temperatures are written in degrees Kelvin, for example we write 273 K.

Forces and hence weights are in newtons (N) not kilograms. Beware of weights quoted in kilograms; in the old (pre-SI) metric system still commonly used in parts of Europe, the name kilogram was also used for weight or force. To convert weights given in kilograms to newtons, simply multiply by 9.81.

The SI system is known as a coherent system, which effectively means that you can put the values into formulae without having to worry about conver­sion factors. For example, in the expression relating force to mass and acceleration: F = m X a, we find that a force of 1 newton acting on a mass of 1 kilogram produces an acceleration of 1 m/s2. Contrast this with a version of the old British ‘Imperial’ system where a force of 1 pound acting on a mass of 1 pound produces an acceleration of 32.18 ft/sec2. You can imagine the prob­lems that the latter system produces. Notice how in this system, the same name, the pound, is used for two different things, force and mass.

Because aviation is dominated by American influence, American Federal units and the similar Imperial (British) units are still in widespread use. Apart from the problem of having no internationally agreed standard, the use of Federal or Imperial units can cause confusion, because there are several alternative units within the system. In particular, there are two alternative units for mass, the pound mass, and the slug (which is equivalent to 32.18 pounds mass). The slug may be unfamiliar to most readers, but it is commonly used in aeronautical engineering because, as with the SI units, it produces a coherent system. A force of 1 pound acting on a mass of one slug produces an acceleration of 1 ft/sec2. The other two basic units in this system are, as you may have noticed, the foot and the second. Temperatures are measured in degrees Fahrenheit.

You may find all this rather confusing, but to make matters worse, in order to avoid dangerous mistakes, international navigation and aircraft operations conventions use the foot for altitude, and the knot for speed. The knot is a nautical mile per hour (0.5145 m/s). A nautical mile is longer than a land mile, being 6080 feet instead of 5280 feet. Just to add a final blow, baggage is nor­mally weighed in kilograms (not even newtons)!

To help the reader, most of the problems and examples in this book are in SI units. If you are presented with unfamiliar units or mixtures of units, convert them to SI units first, and then work in SI units. One final tip is that when working out problems, it is always better to use basic units, so convert millimetres or kilometres to metres before applying any formulae. In the real world of aviation, you will have to get used to dealing with other units such as slugs and knots, but let us take one step at a time. Below, we give a simple example of a calculation using SI units (see Example 1.1).

EXAMPLE 1.1

The mass of an aeroplane is 2000 kg. What force, in addition to that required to overcome friction and air resistance, will be needed to give it an accelera­tion of 2 m/s2 during take-off?

SOLUTION Force = ma

= 2000 X 2 = 4000 newtons

This shows how easy is the solution of such problems if we use the SI units.

Many numerical examples on the relationship between forces and masses involve also the principles of simple kinematics, and the reader who is not familiar with these should read the next paragraph before he tackles the examples.

All objects near the surface of the earth have the force of gravity acting on them. If there is no opposing force, then they will start to move, to accelerate. The rate at which they accelerate is independent of their mass.

The force due to gravity (weight) F = m X g

but, from Newton’s second law, F = m X acceleration

By equating the two expressions above, we can see that the acceleration due to gravity will be numerically equal to the gravity constant g, and will be inde­pendent of the mass. Not surprisingly, many people confuse the two terms ‘gravity constant’ and ‘acceleration due to gravity’, and think that they are the same thing. The numerical value is the same, but they are different things. If a book rests on a table, then the weight is given by the product of the gravity

Fig 1.3 Aerodynamic and body forces constant and the mass, but it is not accelerating. If it falls off the table, it will then accelerate at a rate equal to the value of the gravity constant.

This brings us to the old problem of the feather and the lump of lead; which will fall fastest? Well, the answer is that in the vacuum of space, they would both fall at the same rate. In the atmosphere, however, the feather would be subjected to a much larger aerodynamic resistance force in relation to the accelerating gravity force (the weight), and therefore the feather would fall more slowly.

For all objects falling through the atmosphere, there is a speed at which the aerodynamic resistance is equal to the weight, so they will then cease to accel­erate. This speed is called the terminal velocity and will depend on the shape, the density and the orientation of the object. A man will fall faster head first than if he can fall flat. Free-fall sky-divers use this latter effect to control their rate of descent in free fall.