In the study of viscous flows, a flow field in which p, p, and к are treated as constants is sometimes labeled as “constant property” flow. This assumption is made in the present section. On a physical basis, this means that we are dealing with an incompressible flow, where p is constant. Also, since /і and к are functions of temperature (see Section 15.3), constant property flow implies that T is constant also. (We will relax this assumption slightly at the end of this section.)
The governing equations for Couette flow were derived in Section 16.2. In particular, the у-momentum equation, Equation (16.2), along with the geometrical property that Hp/Hx = 0, states that the pressure is constant throughout the flow. Consequently, all the information about the velocity field comes from the x-momentum equation, Equation (16.1), repeated below:
[16.1]
For constant д, this becomes
[16.4]
Integrating with respect to у twice, we obtain
и = ay + b
where a and b are constants of integration. These constant can be obtained from the boundary conditions illustrated in Figure 16.2, as follows:
At у = 0, и = 0; hence, b — 0.
At у = D, и = ue; hence, a = ue/D.
Thus, the variation of velocity for incompressible Couette flow is given by Equation (16.5) as
[16.6]
Note the important result that the velocity varies linearly across the flow. This result is sketched in Figure 16.3.
Once the velocity profile is obtained, we can obtain the shear stress at any point in the flow from Equation (15.1), repeated below (the subscript yx is dropped here because we know the only shear stress acting in this problem is that in the x direction):
[16.7]
From Equation (16.6),
3 и ue
з7 ~ z>
Hence, from Equations (16.7) and (16.8), we have
[16.9]
Note that the shear stress is constant throughout the flow. Moreover, the straightforward result given by Equation (16.9) illustrates two important physical trends— trends that we will find to be almost universally present in all viscous flows:
1. As ue increases, the shear stress increases. From Equation (16.9), r increases linearly with ue this is a specific result germane to Couette flow. For other problems, the increase is not necessarily linear.
2. As D increases, the shear stress decreases; that is, as the thickness of the viscous shear layer increases, all other things being equal, the shear stress becomes smaller. From Equation (16.9), r is inversely proportional to D—again a result germane to Couette flow. For other problems, the decrease in r is not necessarily in direct inverse proportion to the shear-layer thickness.
With the above results in mind, reflect for a moment on the quotation from Isaac Newton’s Principia given at the beginning of this chapter. Here, the “want of lubricity” is, in modem terms, interpreted as the shear stress. This want of lubricity is, according to Newton, “proportional to the velocity with which the parts of the fluid are separated from one another,” that is, in the context of the present problem proportional to ue/D. This is precisely the statement contained in Equation (16.9). In more recent times, Newton’s statement is generalized to the form given by Equation (16.7), and even more generalized by Equation (15.1). For this reason, Equations (15.1) and
(16.7) are frequently called the newtonian shear stress law, and fluids which obey this law are called newtonian fluids. [There are some specialized fluids which do not obey Equation (15.1) or (16.7); they are called non-newtonian fluids—some polymers and blood are two such examples.] By far, the vast majority of aeronautical applications deal with air or other gases, which are newtonian fluids. In hydrodynamics, water is the primary medium, and it is a newtonian fluid. Therefore, we will deal exclusively with newtonian fluids in this book. Consequently, the quote given at the beginning of this chapter is one of Newton’s most important contributions to fluid mechanics—it represents the first time in history where shear stress is recognized as being proportional to a velocity gradient.
Let us now turn our attention to heat transfer in a Couette flow. Here, we continue our assumptions of constant p, p. and k. but at the same time, we will allow a temperature gradient to exist in the flow. In an exact sense, this is inconsistent; if T varies throughout the flow, then p, p, and к also vary. However, for this application, we assume that the temperature variations are small—indeed, small enough such that p, p, and к are approximately constant—and treat them so in the equations. On the other hand, the temperature changes, although small on an absolute basis, are sufficient to result in meaningful heat flux through the fluid. The results obtained will reflect some of the important trends in aerodynamic heating associated with high-speed flows, to be discussed in subsequent chapters.
For Couette flow with heat transfer, return to Figure 16.2. Here, the temperature of the upper plate is Te and that of the lower plate is Tw. Hence, we have as boundary conditions for the temperature of the fluid:
At у = 0: At у = D:
The temperature profile in the flow is governed by the energy equation, Equation
(16.3) . For constant p and k, this equation is written as
[16.10]
Also, since p is assumed to be constant, Equations (16.10) and (16.1) are totally uncoupled. That is, for the constant property flow considered here, the solution of the momentum equation [Equation (16.1)] is totally separate from the solution of the energy equation [Equation (16.10)]. Therefore, in this problem, although the temperature is allowed to vary, the velocity field is still given by Equation (16.6), as sketched in Figure 16.3.
In dealing with flows where energy concepts are important, the enthalpy h is frequently a more fundamental variable than temperature; we have seen much evidence of this in Part 3, where energy changes were a vital consideration. In the present problem, where the temperature changes are small enough to justify the assumptions of constant p, p, and k, this is not quite the same situation. However, because we will need to solve Equation (16.10), which is an energy equation for a flow (no matter how small the energy changes), and because we are using Couette flow as an example to set the stage for more complex problems, it is instructional (but by no means necessary) to couch Equation (16.10) in terms of enthalpy. Assuming constant specific heat, we have
[16.11]
Equation (16.11) is valid for the Couette flow of any fluid with constant heat capacity; here, the germane specific heat is that at constant pressure cp because the entire flow field is at constant pressure. In this sense, Equation (16.11) is a result of applying the first law of thermodynamics to a constant pressure process and recalling the fundamental definition of heat capacity as the heat added per unit change in temperature, Sq/dT. Of course, if the fluid is a calorically perfect gas, then Equation (16.11) is
a basic thermodynamic property of such a gas quite independent of what the process may
Note that h varies parabolically with y/D across the flow. Since T = h/cp, then the temperature profile across the flow is also parabolic. The precise shape of the parabolic curve depends on hw (or Tw), he (or Te), and Pr. Also note that, as expected from our discussion of the viscous flow similarity parameters in Section 15.6, the
Prandtl number is clearly a strong player in the results; Equation (16.16) is one such example.
Once the enthalpy (or temperature) profile is obtained, we can obtain the heat flux at any point in the flow from Equation (15.2), repeated below (the subscript у is dropped here because we know the only direction of heat transfer is in the у direction for this problem):
д T
q = – k — [16.17]
dy
Equation (16.17) can be written as
k dh r,
g =——— [16.18]
cP dy
In Equation (16.18), the enthalpy gradient is obtained by differentiating Equation (16.16) as follows:
Inserting Equation (16.19) into Equation (16.18), and writing k/cp as /і/ Pr, we have
From Equation (16.20), note that q is not constant across the flow, unlike the shear stress discussed earlier. Rather, q varies linearly with y. The physical reason for the variation of q is viscous dissipation which takes place within the flow, and which is associated with the shear stress in the flow. Indeed, the last term in Equation (16.20), in light of Equations (16.6) and (16.9), can be written as
= ти
Hence, Equation (16.20) becomes
The variation of q across the flow is due to the last term in Equation (16.21), and this term involves shear stress multiplied by velocity. The term ти is viscous dissipation; it is the time rate of heat generated at a point in the flow by one streamline at a given velocity “rubbing” against an adjacent streamline at a slightly different velocity— analogous to the heat you feel when rubbing your hands together vigorously. Note that, if ue is negligibly small, then the viscous dissipation is small and can be neglected; that is, in Equation (16.20) the last term can be neglected (ue is small), and in Equation (16.21) the last term can be neglected (r is small if ue is small). In this case, the heat flux becomes constant across the flow, simply equal to
In this case, the “driving potential” for heat transfer across the flow is simply the enthalpy difference (he — h „ ) or, in other words, the temperature difference (Te — 7 „) across the flow. However, as we have emphasized, if ue is not negligible, then viscous dissipation becomes another factor that drives the heat transfer across the flow.
Of particular practical interest is the heat flux at the walls—the aerodynamic heating as we label it here. We denote the heat transfer at a wall as qw. Moreover, it is conventional to quote aerodynamic heating at a wall without any sign convention. For example, if the heat transfer from the fluid to the wall is 10 W/cm2, or, if in reverse the heat transfer from the wall to the fluid is 10 W/cm2, it is simply quoted as such; in both cases, qw is given as 10 W/cm2 without any sign convention. In this sense, we write Equation (16.18) as
where the subscript w implies conditions at the wall. The direction of the net heat transfer at the wall, whether it is from the fluid to the wall or from the wall to the fluid, is easily seen from the temperature gradient at the wall; if the wall is cooler than the adjacent fluid, heat is transferred into the wall, and if the wall is hotter than the adjacent fluid, heat is transferred into the fluid. Another criterion is to compare the wall temperature with the adiabatic wall temperature, to be defined shortly.
Return to the picture of Couette flow in Figure 16.2. To calculate the heat transfer at the lower wall, use Equation (16.23) with the enthalpy gradient given by Equation (16.19) evaluated at у = 0:
To calculate the heat transfer at the upper wall, use Equation (16.23) with the enthalpy gradient given by Equation (16.19) evaluated at у = D. In this case, Equation (16.19) yields
dh he – hw + Pr и2 Pr u] _ he – hw – Pr и2 By D D D
In turn, from Equation (16.23)
Let us examine the above results for three different scenarios, namely, (1) negligible viscous dissipation, (2) equal wall temperature, and (3) adiabatic wall conditions (no heat transfer to the wall). In the process, we define three important concepts in the analysis of aerodynamic heating: (1) adiabatic wall temperatue, (2) recovery factor, and (3) Reynolds analogy.
![]()
![]() P0,2 __ P0,2 Pi Pi Pi Pi
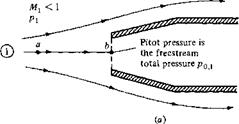
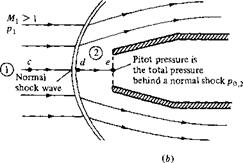
P0,2 __ P0,2 Pi Pi Pi Pi![]() A Pitot tube is inserted into an airflow where the static pressure is 1 atm. Calculate the flow Mach number when the Pitot tube measures (a) 1.276 atm, (b) 2.714 atm, (c) 12.06 atm.
A Pitot tube is inserted into an airflow where the static pressure is 1 atm. Calculate the flow Mach number when the Pitot tube measures (a) 1.276 atm, (b) 2.714 atm, (c) 12.06 atm.![]()
![]() Ps = Po,2 = (Pi) = 82.87(973.3) =
Ps = Po,2 = (Pi) = 82.87(973.3) =![]() _ 8.07 x 104
_ 8.07 x 104![]() p, = рог = ( — ) Po і = (0.8488 x 10~z)(9.502 x 106) =
p, = рог = ( — ) Po і = (0.8488 x 10~z)(9.502 x 106) =