Analogy of Lighthill

The key idea of Lighthill’s analogy [Lighthill (1952-54)] is to derive a wave equation starting from the exact mass conservation equation (5) and the momentum equation (9):

дР і і f dxi + dxj + fl

and

Taking the time derivative of (5) and subtracting from it, the divergence of (9) we obtain the exact equation:

d2p d2pVjVj = d^p _ d2Tij dfi

dt2 dxldxj dx2 dxldxj dxl

1 d 2

which is quite meaningless. By adding Ф on both sides and rearranging the terms, making use of the fact that we chose c0 to be a constant, we can write (38) as a wave equation:

1 = d2pVjVj – Tij dfi + p_

Cq dt2 dx2 dxldxj dxl dt2 c2 P)

This equation is still exact and still generally meaningless. We could have chosen co to be a millimetre per century or equal to the speed of light. In order to have a meaningful equation we now assume that we consider sound production by a flow bounded by a fluid displaying small perturbations from a uniform stagnant state with speed of sound equal to c0 (Figure 2). We furthermore define the perturbations in the pressure p’ = p — p0 and density p’ = p — p0 as deviations from the state (p0,p0) of this reference uniform

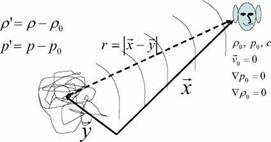
Figure 2. Sound sources and listener in the analogy of Lighthill

stagnant reference fluid. As the reference state is constant and uniform we can write (39) as:

1 dV _ Sy = d2pvjVj – Tij dfi + (P_ _ Л (40)

c° dt2 dx2 dxidxj dxi dt2 c2 P

We will see (section 4) that this equation describes the propagation of acous­tic waves in the uniform stagnant fluid when the right hand side of the equation (40) is negligible. In regions where the right hand side is not neg­ligible, it describes the generation of sound. However, because the equation of Lighthill is a single exact equation for many unknowns, we will not obtain any result without approximations. Lighthill has shown that these approxi­mations can best be introduced into an integral formulation of (40). We will now consider basic acoustic wave propagation allowing to understand some elementary aspects of the problem and to derive the integral formulation.

An interesting aspect of the analogy is that the sound source we find depends on the choice of the acoustic variable. Until now we have chosen pressure fluctuations p’ to describe the acoustic field. We could also have followed a similar procedure to obtain a wave equation for the density fluc­tuations p’. Starting from (38) we now subtract from both sides of the equation the term c0V2p’ to find:

In principle equations (40) and (41) are identical. However the pressure formulation (40) is most convenient when considering sound production by combustion processes in which the time-dependent combustion yields time- dependent fluctuations in the entropy. In contrast, when considering a flow in spatially non-uniform fluids with large variations in speed of sound the density formulation (41) will be the most suitable. An example of this is the sound generation by turbulence in bubbly liquids (section 5.2). In this case the sound production appears to be dominated by the effect of differences in the speed of sound.

Equation (41) is often written for convenience in terms of the stress tensor of Lighthill :

dV _ 2 dV = d2 Tij df

dt2 0 dx2 dxidxj dxi

where the stress tensor Tij is defined by:

Tij = pviVj – Tij + (p’ – clp’) Sij