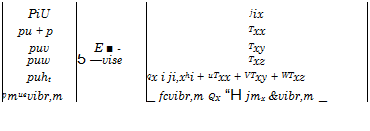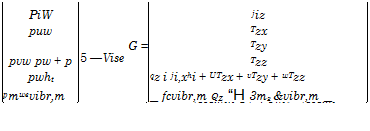Wall Shear Stress at Attachment Lines
The forward stagnation point is a singular point in which the wall shear stress is zero. In three-dimensional flow along an attachment line, like along a separation line, we have a finite wall shear stress, tw > 0. The direction of the wall shear-stress vector tw is coincident with the direction of the attachment line flow, which also holds for a separation line [1]. If we have an infinite swept situation, the wall shear stress is constant along the respective line.
We remember the discussion at the beginning of Sub-Section 7.2.2 and consider the wall shear stress along the attachment line of an infinite swept circular cylinder, ‘scy ’, with the reference-temperature extension in generalized form [16], assuming perfect-gas flow (This wall shear stress is constant in the z-direction, Fig. 6.37 b).):
Tw, scy scy 1 (7.149)
with
The wall shear stress of the laminar as well as of the turbulent boundary layer increases linearly with increasing dynamic pressure.
The larger T*/Tref, the smaller is the wall shear stress, like in the case of the boundary layer on flat surface portions. For the wall shear stress of the laminar boundary-layer holds tWi lam ж (T*/T^)-0’175 and for that of the turbulent boundary-layer tWi turb ж (T*/T^)-0’653.
For the influence of other factors see the summary for the wall shear stress on flat surface portions at the end of Sub-Section 7.2.3.
[108] In [10] it is shown that the Reynolds analogy is valid strictly only for constant wall temperature. For radiation-cooled surface it hence holds only approximately.
[109] Again we remember that for flat plates at zero angle of attack, and hence also at CAV’s at small angle of attack, except for the blunt nose region, we can choose ‘ref ’ = W, whereas at RV’s we must choose the conditions at the outer edge of the boundary layer: ‘ref ’ = ‘e’.
For both case 1 and case 2, laminar and turbulent flow, we have a linear dependence on the temperature difference Tr — Tw. The larger this difference, the larger is T4a or qgw. However, note that in case 2 Tw is given, whereas in case 1 Tw = Tra is the unknown.
7.2.6 The Thermal State of Stagnation Points and Attachment Lines
Remembering the discussion at the beginning of Sub-Section 7.2.2 we study the situation at a sphere, respectively the circular cylinder (2-D case), and
These are the basic dependencies of the thermal state of the surface. On actual configurations dependencies exist, which are inversely similar to the dependencies of the respective boundary-layer thicknesses, Sub-Section 7.2.1. Important is that super-critical wall roughness, waviness etc. increase either T^a or qgw if the flow is turbulent.
For formulations by other authors of qgw for stagnation points see, e. g., [36].
For laminar and turbulent flow, we have a linear dependence on the temperature difference Tr — Tra. The larger this difference, the larger is T4a. Note that in case 2 , which is not included in Table 7.8, Tw is given, whereas in Case 1 Tw = Tra is the unknown.
These are the basic dependencies of the thermal state of the surface. On actual configurations dependencies exist especially for the infinite swept circular cylinder, which are inversely similar to the dependencies of the respective boundary-layer thicknesses, Sub-Section 7.2.1. Important is that in the case of the infinite swept circular cylinder super-critical wall roughness, waviness etc. also increase either T4a or qgw if the flow is turbulent.
No exact and reliable non-empirical transition criterion is available today, see Section 8.4.
[116] In [2] it is reported that during a Space Shuttle Orbiter re-entry these are typically at most Me ~ 2.5, and mostly below Me ~ 2.
[117] The (unstable) boundary layer responds to disturbances which are present in flight or, but in general wrongly, in the ground-simulation facility, Sub-Section 8.3.
[118] The thermal state of the surface is seen especially important in view of research activities in ground-simulation facilities and in view of hypersonic flight experiments. In both so far the thermal state of the surface usually is either uncontrolled or not recorded.
[119] For a detailed discussion in a recent publication see [15].
[120] The radiation-adiabatic temperature and the heat flux in the gas at the wall with fixed cold wall temperature show a similar qualitative behavior.
[121] Tollmien-Schlichting waves can propagate with the wave vector aligned with the main-flow direction (“normal” wave as a two-dimensional disturbance, wave angle ф = 0) or lying at a finite angle to it (“oblique” wave as three-dimensional disturbance, wave angle ф = 0). The most amplified Tollmien-Schlichting waves in two-dimensional low-speed flows are usually the two-dimensional waves, and in two-dimensional supersonic and hypersonic flows the oblique waves.
[122] In [15] the branches I and II are, less idealized, divided into five stages : 1) disturbance reception (branch I ahead of xcr and part of sub-branch IIa), 2) linear growth of (unstable) disturbances (largest part of sub-branch IIa), 3) non-linear saturation (towards the end of sub-branch IIa), 4) secondary instability (towards the very end of sub-branch IIa), 5) breakdown (begin of sub-branch IIb). The term “breakdown” has found entry into the literature. It is a somewhat misleading term in so far as it suggests a sudden change of the (secondary unstable) flow into the turbulent state. Actually it means the “breakdown” of identifiable structures in the disturbance flow. In sub-branch IIb a true “transition” into turbulence occurs. Sub-branch IIb, i. e., the length xtr, u — xtr>l, can be rather large, especially in high-speed flows.
[123] If Ax’tr is large compared to a characteristic body length—a problem in particular appearing in turbo-machinery—, this must be taken into account in the turbulence model of an employed computation method, see, e. g., [20].
[124] This overshoot occurs, because the (viscous sub-layer) thickness of the turbulent boundary layer is initially smaller than what it would have been, if the boundary layer grew turbulent from the plate’s leading edge on.
[125] In reality this is allowed only for large Reynolds numbers, see Sub-Section 7.1.5, page 233. Situations exist in which this assumption is critical, because information of the mean flow is lost, which is of importance for the stability/instability behavior of the boundary layer (“non-parallel” effects [15]).
[126] Non-local stability theory and methods, see Sub-Section 8.4.1, are based on parabolized stability equations (PSE). With them the stability properties are investigated taking into account the whole boundary-layer domain of interest.
[127] Ovv etc. stands for twofold differentiation with respect to y etc.
[128] The boundary-layer thickness 5, found, for instance, with eq. (7.91), Sub-Section 7.2.1, defines the boundary-layer edge. For practical purposes usually the displacement thickness 51 or the momentum thickness 52 is employed.
[129] The reader is warned that this is a highly simplified discussion. The objective is only to arrive at insights into the basic instability behavior, not to present detailed theory.
[130] In this case the result holds only for an air or gas boundary layer, Section 7.1.5, page 231.
[131] Due to the larger amount of tangential momentum flux close to the surface, the turbulent boundary layer can negotiate a larger adverse pressure gradient than the laminar one.
[132] In [33] it is argued that these modes are not necessarily two distinct modes. The authors therefore recommend a new terminology.
Of course, this plot does not indicate how finally the disturbance amplification will behave.
[134] Three transition scenarios can be distinguished [15]: “fundamental breakdown”, i. e., transition due to secondary instability of Tollmien-Schlichting waves, “streak breakdown” due to secondary instability of stream-wise vortices, “oblique breakdown”, i. e., transition governed by the growth of oblique waves, which in general happens in two-dimensional supersonic and hypersonic flows.
[135] We remember from Section 6.4.2 that the entropy layer can have a slip-flow profile like shape or a wake-like shape. It appears that so far the wake-like shape has not found much attention.
[136] At a non-swept, infinitely long cylinder an attachment line exists, where the flow comes fully to rest. In the two-dimensional picture this attachment line is just the forward (primary) stagnation point.
[137] This can be a dent on the plate’s surface, or an insect cadaver at the leading edge of the wing.
[138] Remember in this context the global characteristic properties of attached viscous flow, Sub-Chapter 7.1.4.
[139] Regarding the general problems of flow past aerodynamic stabilization, trim and control surfaces see [1].
[140] In Section 1.4 surface catalycity is counted as permissible property. In general this means that it should be as small as possible in order to reduce the thermal loads. One can imagine, however, also to use wall catalycity purposefully in order to influence the boundary-layer behavior via the thermal state of the surface.
[141] In the literature a distinction between critical and effective roughness height can be found. The critical height is the height at which a first impact of the roughness can be determined, usually an upstream shift of the transition location. The effective height is that at which transition is observed directly downstream of the roughness. Yet another increase of the roughness height would not further affect the transition location.
[142] Major issues are the location of the boundary-layer tripping device (roughness elements, etc.) on the wind tunnel model, the effectiveness of the device, and the avoidance of over-tripping (e. g., too large roughness height), which would falsify the properties (displacement thickness, wall shear stress, heat flux) of the ensuing turbulent boundary layer, see, e. g., [83].
[143] The influence on instability (and transition) of the thermo-chemical nonequilibrium of the gas due to freezing phenomena in the nozzles of high-enthalpy simulation facilities, Sub-Section 8.2.7, is also an environment issue, although not a disturbance environment issue.
[144] The apparent dependence of transition on the unit Reynolds number Reu = pu/^ is a facility-induced effect.
[145] For a comparative study of second-mode instability in the Purdue and the Braunschweig facility see [100].
[146] Note that the result of linear stability theory is the relative growth of (unstable) disturbances of unspecified small magnitude, eq. (8.11) or (8.12), only.
[147] The transition zone in reality is an arbitrarily shaped zone with rather small downstream extent wrapped around the configuration. A low-speed example is discussed in [28].
[148] An alternative approach with a large potential also for optimization purposes, e. g., to influence the instability and transition behavior of the flow by passive or active means, is to use adjoint equation systems [131].
[149] At the (small) blunt nose of such a vehicle of course the edge temperatures are large, but there the boundary layer is not yet turbulent.
[150] We remember that the laminar Prandtl and Schmidt number are in fact functions of the temperature, Section 4.1.
[151] The situation in reality is much more complicated than that at the canonical flat plate/compression ramp configuration: flow through the gap in the hinge line region, surface radiation cooling with non-convex effects, and surface-tangential heat transport in the structure [1].
[152] Depending on the given problem it can be advisable in a computation scheme to take iteratively into account the displacement properties of the boundary layer with, for instance, perturbation coupling, see, Sub-Section 7.2.1.
(C Springer International Publishing Switzerland 2015 E. H. Hirschel, Basics of Aerothermodynamics,
DOI: 10.1007/978-3-319-14373-6 _9
[153] Only in such points streamlines actually terminate at (attach) or leave from (detach or separate) the body surface. This holds also in two dimensions, where the separation point is such a singular point, where the separation streamline leaves the surface under a finite angle [5].
[154] Sub-critical flight means without total-pressure loss due to an embedded shock wave. The schematic holds, however, also for flow with total-pressure loss due to shock waves.
[155] Local separation, however, can have a global influence on the aerodynamic forces and moments of a flight vehicle, if, for instance, the effectiveness of aerodynamic trim or control surfaces is affected.
[156] See also the BDW case study, Section 3.3.
[157] Remember that the lift to drag ratio of the Space Shuttle Orbiter was underestimated in the supersonic and the subsonic Mach number regime, Section 3.1. How far the insufficient simulation of separation played a role has not yet been determined.
[158] A detailed discussion of the aerothermodynamic issues of stabilization, trim and control devices can be found in [14].
[159] The type V interaction looks similar, but the reattachment shock below the triple point T would have a A like structure, and the expansion fan would originate below T.
[160] We remember from Section 3.1 that the recovery temperature is constant only in the frame of the flat-plate relation given there.
[161] Results of an experimental/numerical study of the length of the separation zone at different wall temperatures are given in Section 10.8.
With increasing angle of attack the excess thermal loads along the leading edge are becoming smaller [24].
[163] For the HERMES configuration it was demanded that the winglets were lying, for all re-entry flight attitudes, completely, i. e., without interference, within the bow-shock surface [40]. For the Space Shuttle Orbiter configuration, but also for a CAV as shown in Fig. 6.4, interactions cannot be avoided and suitable measures must be taken in order to ensure the structural integrity of the airframe.
As we have already noted, it may be necessary to take iteratively into account the displacement properties of the boundary layer, Sub-Section 7.2.1.
Argon is a monatomic gas, the bulk viscosity therefore is zero: к = 0.
[166] Remember that the weak and the strong interaction limits are asymptotic limits with an intermediate area between them, for which however also a description can be provided [47].
[167] However, we have noted above that experience shows that the Navier-Stokes equations can be strained to a certain degree.
This holds also for the experimental realization of the example in the preceding section.
Flow fields of this kind are present at RV’s and AOTV’s at large speeds and altitudes. Hence it is the rule that high-temperature real-gas effects are present in such flow fields.
[170] A major issue is the dependence of the catalytic surface recombination coefficients on the wall temperature, see, e. g., [2].
(C Springer International Publishing Switzerland 2015 E. H. Hirschel, Basics of Aerothermodynamics,
DOI: 10.1007/978-3-319-14373-6 _10
[171] We give the exponent of the relation for the viscous sub-layer and not that for the turbulent scaling thickness, Sub-Section 7.2.1.
[172] Remember that in boundary-layer theory the direction normal to the wall usually is defined as ^-coordinate.
[173] It is to be questioned whether it is permitted to use the boundary-layer approach in this case. Due to the very steep temperature gradient at the wall, the boundary-layer assumptions may not hold.
[174] The viability of the approach to influence the boundary layer by cooling/heating on the middle segment and to investigate the stability properties of the boundary layer downstream on the rear segment can be deduced from the theoreti – cal/numerical results given in [7].
[175] Note that the primary separation location is stronger shifted upstream than the attachment location is shifted downstream. This is also observed in laminar ramp flow, Section 10.8.
[176] The enlargement of the boundary-layer thicknesses is shown in the insets in the lower right corners of the figures. (The insets actually show the thicknesses of the temperature boundary layers.) Note that also the separation lengths around the corner point between the two ramps are enlarged. The influence of the wall temperature on both the boundary-layer thickness and the separation length was treated in previous sections.
[177]
q = c^TI-
The value of the constant c is determined at x = 1 m:
c = qx0’5 = 0.0617 MW/m1’5.
[178] We include only the transport of non-equilibrium vibration energy. Equations for other entities, like rotation energy, can be added. For a more general formulation
[179] We have not given the equation(s) for the transport of vibrational energy in Section 5.4, but have referred instead to [5].
[180] Details can be found, for instance, at
http://www. chemie. fu-berlin. de/chemistry/general/si_en. html.
http://physics. nist. gov/cuu/Units/units. html or at











 effective ratio of specific heats, p. 178, [-] recombination coefficient of atomic species, p. 132, [-] characteristic boundary-layer thickness, p. 44, [L] shock stand-off distance, p. 176, [L] flow (ordinary) boundary-layer thickness, p. 93, [L] ramp angle, p. 163, [°]
effective ratio of specific heats, p. 178, [-] recombination coefficient of atomic species, p. 132, [-] characteristic boundary-layer thickness, p. 44, [L] shock stand-off distance, p. 176, [L] flow (ordinary) boundary-layer thickness, p. 93, [L] ramp angle, p. 163, [°] aeroassisted orbital transfer vehicle, p. 3 ascent and re-entry vehicle, p. 3 blunt delta wing, p. 63 cruise and acceleration vehicle, p. 3 direct numerical solution, p. 296
aeroassisted orbital transfer vehicle, p. 3 ascent and re-entry vehicle, p. 3 blunt delta wing, p. 63 cruise and acceleration vehicle, p. 3 direct numerical solution, p. 296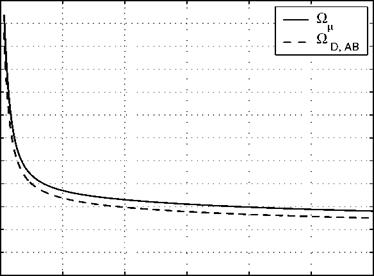 2.75
2.75