Inviscid, Incompressible Flow Past Circular Cylinders and Joukowski Airfoils
2.1 Background
2.1.1 Notation
In two-dimensions, the obstacles are cylinders of various cross sections, whose axis is perpendicular to the plane in which the flow is taking place (Fig. 2.1). When a wing will be considered, the wing span will be described by the variable y. Hence, the flow past cylinders will take place in the (x, z) plane, see Fig. 2.2. In this chapter we will consider steady, 2-D, inviscid, incompressible, adiabatic and irrotational flow, also called potential flow. The influence of gravity will be neglected.
2.1.2 Governing Equations
Lets (u, w) represent the perturbation velocity or deviation from the uniform flow V«, = (U, 0). At any point in the flow field the velocity is V = (U + u, w), the density is p = const. and the pressure p. The conservation of mass and irrotationality condition form a system of two linear first-order partial differential equations (PDEs) for (u, w) as
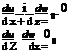 (2.1)
(2.1)
In local polar coordinates (r, в), the system reads in terms of V = (Vr, Ve)
dr Vr і dve 0
![]() dr + de =v
dr + de =v
dr ve dvr 0
dr дв = 0
© Springer Science+Business Media Dordrecht 2015
J. J. Chattot and M. M. Hafez, Theoretical and Applied Aerodynamics,
DOI 10.1007/978-94-017-9825-9_2
 Fig. 2.2 Coordinate system and notation
Fig. 2.2 Coordinate system and notation
where Vr = U cos в + vr and Ve = —U sin в + ve are the total velocity components, including the contribution of the uniform flow. The irrotationality condition is the consequence of conservation of momentum for inviscid, adiabatic, incompressible flow. In other words, a solution to the above system also satisfies the momentum equations. The energy equation (conservation of mechanical energy) is also satisfied, under the stated assumptions.
The pressure is obtained from Bernoulli’s equation, again a consequence of the momentum conservation (it can be interpreted also as a conservation of mechanical energy per unit mass, where the potential energy is constant):
![]()
l + 2 V2 = ^ + 1 V^= 1U2 = const.
p 2 p 2 p 2
Note that the Bernoulli’s equation is nonlinear. However, as we will see, it can be linearized under the assumption of small perturbation.











