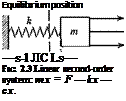
APPLICATION TO DIFFERENTIAL EQUATIONS

The Laplace transform finds one of its most important uses in the theory of linear differential equations. The commonest application in airplane dynamics is to ordinary equations with constant coefficients. The technique for the general case is given in Sec. 3.2. Here we illustrate it with the simple but important example of a spring-mass-damper system acted on by an external force (Fig. 2.3). The differential equation of the system is

* + 4<onx + co2x = f(t) (2.4,1)

2£(ои is the viscous resistance per unit mass, c/m, со,,2 is the spring rate per unit mass, kjm, and f(t) is the external force per unit mass. The Laplace

transform of (2.4,1) is formed by multiplying through by e~si and integrating term by term from zero to infinity. This gives

& И +

Upon using the results of Sec. 2.3, this equation may be written S2X + 2 lmnsx + 0Jn2x = / + x(0) + вж(0) + 2£conx(0)

or щв) = /+ *(0) + (« + 2Ccu>(0)

s2 + 2 t, a>ns + eon2

The original differential equation (2.4,1) has been converted by the trans­formation into the algebraic equation (2.4,3) which is easily solved (2.4,4) to find the transform of the unknown function. In the numerator of the right-hand side of (2.4,4) we find a term dependent on the excitation (/), and terms dependent on the initial conditions [i(0) and ж(0)]. The denomi­nator is the characteristic polynominal of the system. As exemplified here, finding the Laplace transform of the desired solution x(t) is usually a very simple process. The heart of the problem is the passage from the transform x(s) to the function x(t). Methods for carrying out the inverse transformation
are described in Sec. 2.6. Before proceeding to these, however, some general comments on the method are in order.

One of the advantages of solving differential equations by the Laplace transform is that the initial conditions are automatically taken into account. When the inverse transformation of (2.4,4) is carried out, the solution applies for the given forcing function f(t) and the given initial conditions. By contrast, when other methods are used, a general solution is usually obtained which has in it a number of arbitrary constants. These must sub­sequently be fitted to the initial conditions. This process, although simple in principle, becomes extremely tedious for systems of order higher than the third. A second convenience made possible by the transform method is that in systems of many degrees of freedom, represented by simultaneous differential equations, the solution for any one variable may be found independently of the others.