Conservation of Mass

Fluid passing through an area at a velocity of V has a mass flow rate equal to pAV. This is easily seen by reference to Figure 2.6. Here flow is

Reference

plane

Figure 2.6 Mass flow through a surface.

pictured along a streamtube of cross-sectional area A. The fluid velocity is equal to V. At time t = 0, picture a small slug of fluid of length, 1, about to cross a reference plane. At time //V, this entire slug will have passed through the reference plane. The volume of the slug is Al, so that a mass of pAl was transported across the reference plane during the time 1/V. Hence the mass rate of flow, m, is given by

pAl m (1/V)

= pAV

Along a streamtube (which may be a conduit with solid walls) the quantity pAV must be a constant if mass is not to accumulate in the system. For incompressible flow, p is a constant, so that the conservation of mass leads to the continuity principle

AV = constant

AV is the volume flow rate and is sometimes referred to as the flux. Similarly, pAV is the mass flux. The mass flux through a surface multiplied by the velocity vector at the surface is defined as the momentum flux. Generally, if the velocity vector is not normal to the surface, the mass flux will be

pAV • n

with the momentum flux written as

(pAV • n)V

here n is the unit vector normal to the surface and in the direction in which the flux is defined to be positive. For example, if the surface encloses a volume and the net mass flux out of the volume is to be calculated, n would

be directed outward from the volume, and the following integral would be evaluated over the entire surface.

pV ndS

Consider the conservation of mass applied to a differential control surface. For simplicity, a two-dimensional flow will be treated. A rectangular contour is shown in Figure 2.7. The flow passing through this element has velocity components of и and v in the center of the element in the x and у directions, respectively. The corresponding components on the right face of the element are found by expanding them in a Taylor series in x and у and dropping second-order and higher terms in Ax. Hence the mass flux out through the right face will be

Г, д(ри)Дл:1 A

Г+-Гт]А’

Writing similar expressions for the other three faces leads to the net mass flux

*

Га^+8£»)1

L to dy J

The net mass flux out of the differential element must equal the rate at which the mass of the fluid contained within the element is decreasing, given by

– (p Ax Ay)

у

м pv + dx/2

pi >

pu

Ax

Figure 2.7 A rectangular-differential control surface.

Since Ax and Ay are arbitrary, it follows that, in general,

dp d(pu) djpv) _ – dt dx dy

In three dimensions the preceding equation can be written in vector notation

as

!f + V-(pV) = 0 (2.17)

where V is the vector operator, del, defined by

Any physically possible flow must satisfy Equation 2.17 at every point in the flow.

For an incompressible flow, the mass density is a constant, so Equation 2.17 reduces to

VV = 0 (2.18)

The above is known as the divergence of the velocity vector, div V.

The Momentum Theorem

The momentum theorem in fluid mechanics is the counterpart of New­ton’s second law of motion in solid mechanics, which states that a force imposed on a system produces a rate of change in the momentum of the system. The theorem can be easily derived by treating the fluid as a collection of fluid particles and applying the second law. The details of the derivation can be found in several texts (e. g., Ref. 2.1) and will not be repeated here.

Defining a control surface as an imaginary closed surface through which a flow is passing, the momentum theorem states:

“The sum of external forces (or moments) acting on a control surface and internal forces (or moments) acting on the fluid within the control surface produces a change in the flux of momentum (or angular momentum) through the surface and an instantaneous rate of change of momentum (or angular momentum) of the fluid particles within the control surface.”

Mathematically, for linear motion of an inviscid fluid, the theorem can be expressed in vector notation by

– j pn dS + B = fsf PV(V ‘ n) dS + Yt f jy f PV dr (2.19)

In Equation 2.19, n is the unit normal directed outward from the surface,

S, enclosing the volume, V. V is the velocity vector, which generally depends on position and time. В represents the vector sum of all body forces within the control surface acting on the fluid, p is the mass density of the fluid defined as the mass per unit volume.

For the angular momentum,

Here, Q is the vector sum of all moments, both internal and external, acting on the control surface or the fluid within the surface, г is the radius vector to a fluid particle.

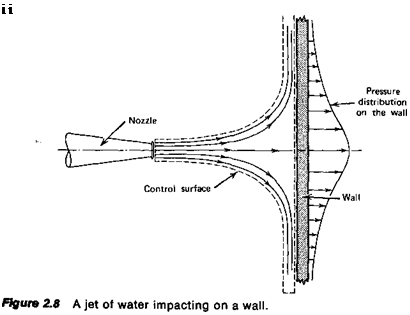As an example of the use of the momentum theorem, consider the force on the burning building produced by the firehose mentioned at the beginning of this chapter. Figure 2.8 illustrates a possible flow pattern, admittedly simplified. Suppose the nozzle has a diameter of 10 cm and water is issuing from the nozzle with a velocity of 60m/s. The mass density of water is approximately 1000 kg/m3. The control surface is shown dotted. Equation 2.19 will now be written for this system in the x direction. Since the flow is steady, the partial*derivative with respect to time of the volume integral given by the last term on the right side of the equation vanishes. Also, В is zero, since the control surface does not enclose any

bodies. Thus Equation 2.19 becomes

-JsfpndS = Jsf pV(V ■ n) dS

Measuring p relative to the atmospheric static pressure, p is zero everywhere along the control surface except at the wall. Here n is directed to the right so that the surface integral on the left becomes the total force exerted on the fluid by the pressure on the wall. К F represents the magnitude of the total force on the wall,

-IF = J / pV(V • n) dS

For the fluid entering the control surface on the left,

V = 60i n = — і

For the fluid leaving the control surface, the unit normal to this cylindrical surface has no component in the x direction. Hence,

– iF = – J j (1000)60i(-60) dS

= — 36 x 105i J J dS

The surface integral reduces to the nozzle area of 7.85 x 10“3 m2. Thus, without actually determining the pressure distribution on the wall, the total force on the wall is found from the momentum theorem to equal 28.3 kN.