LIFT AND PITCHING MOMENT OF THE WING

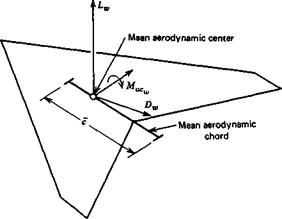
The aerodynamic forces on any lifting surface can be represented as a lift and drag acting at the mean aerodynamic center, together with a pitching couple independent of the angle of attack (Fig. 2.8). The pitching moment of this force system about the CG is given by (Fig. 2.9)[6]

Mw = MaCw + (Lw cos aw + Dw sin aj(h – hnJc

+ (Lw sin aw – Dw cos ajz (2.2,1)

We assume that the angle of attack is sufficiently small to justify the approximations

cos aw = 1, sin aw = aw

and the equation is made nondimensional by dividing through by pV2Sc. It then be­comes

C,„ = Cmacw + (CLn + C, KaJ(h – hnJ + (CLwaw – CDJzJc (2.2,2)

Although it may occasionally be necessary to retain all the terms in (2.2,2), experi­ence has shown that the last term is frequently negligible, and that CDwaw may be ne­glected in comparison with CLu. With these simplifications, we obtain

Cm„ ~ Cmacw + CLw(h – hnJ

= Стаск + “»aw(h ~ KJ (2.2,3)

where a„ = CLa is the lift-curve-slope of the wing.

Equation 2.2,3 will be used to represent the wing pitching moment in the discus­sions that follow.