Convective effects on reflection from an open pipe termina­tion

Until now it was assumed that the fluid in the pipe is stagnant. Now we will consider the influence of a uniform, steady outgoing flow Up in the pipe on the acoustic response of the pipe termination. For plane waves the convected d’Alembert solution is:

(168)

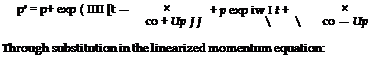du’ dp’

dx

we get: , p+ Л (, x u — ——- exp iu t—————- — poco V V co + Up
p —— exp PQCq
lu	(170)

Looking at a pipe of a uniform cross section Sp, terminated by an orifice plate with opening S0. The flow leaves the pipe through the orifice forming a free jet downstream of the pipe, which contracts slightly after leaving the orifice to reach a minimum cross section Sj before mixing with the surrounding air. The pressure at the minimum cross section in the jet is equal to the pressure of the surroundings. Typically, the contraction factor is Sj/Sq — 0.7 for a thin orifice plate with sharp edges. In the low frequency limit we can describe the flow by using the integral mass conservation law:

ppUpSp — pjUjSj .

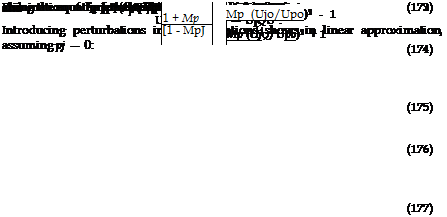
The integration of the momentum equation in the quasi-static approxima­tion, neglecting friction and heat transfer and assuming an irrotational flow (equations 35 and 37) yields:

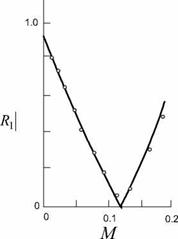
We observe that the pipe termination is anechoic (R — 0) for Mp — Up0/cp0 — (Upq/Uj0)2 ~ (Sj/Sp) (figure 12). This particular behaviour

Figure 12. Convective effects on the reflection and transmission at an ori­fice in a pipe (measurements [Hofmans et al. (2000)]). The upstream reflec­tion coefficient І Ді| displays a sharp minimum at a critical Mach number, as predicted by the theory (theory, 0 experiments).

was first observed and explained by Bechert [1980]. It is a consequence of sound absorption by vortex shedding (modulation of the shear layers of the jet). While the model does not explicitly take the effect of viscosity into account, the assumption that pj = 0 can only be explained by the presence of a free jet, which is a consequence of flow separation due to viscosity. Also we assume implicitly that all the kinetic energy in this jet is dissipated by turbulence without any pressure recovery.

Note that in the presence of flow the acoustical intensity is given by [Morfey (1971)]:

Where m’ is the fluctuation in mass flux:

m = pu

and B’ is the fluctuation in the total enthalpy:

(180)

Consequently a reflection coefficient R = —1 indicates energy losses. This is the limit found when Uj0 = Up0.