Definitions

The thermal state of a surface is governed by at least one temperature and at least one temperature gradient, Section 1.4. Hence both a wall temperature and a temperature gradient, respectively a heat flux, must be given to define it. Large heat fluxes can be present at low surface temperature levels, and vice versa. If a surface is radiation cooled, at least three different heat fluxes at the surface must be distinguished. In the slip-flow regime, which basically belongs to the continuum regime, two temperatures must be distinguished at the surface, Sections 4.3.2 and 9.4.

(C Springer International Publishing Switzerland 2015 E. H. Hirschel, Basics of Aerothermodynamics,

DOI: 10.1007/978-3-319-14373-6 _3

The heat transported per unit area and unit time (heat flux) towards a flight vehicle is

Qо povo hti (3,1)

with ро and vо being the free-stream density and speed (their product is the mass flux per unit area towards the flight vehicle), and ht the total enthalpy (per unit mass) of the free stream, i. e., of the undisturbed atmosphere. It is composed of the enthalpy of the undisturbed atmosphere h0 and the kinetic energy v^/2 of the flow relative to the flight vehicle:

v2

ht = /?co H—(3-2)

At hypersonic speed the kinetic energy is dominant, and hence the total enthalpy is more or less proportional to the flight velocity squared.

A considerable part of the heat transported towards the vehicle is finally transported by diffusive mechanisms towards the vehicle surface. This part is locally the heat flux, which we call the “heat flux in the gas at the wall”, qgw (this heat flux usually is called somewhat misleading the “convective” heat flux). It is directed towards the wall. However, qgw can also be directed away from the wall.

Literature is referring usually only to one heat flux qw = qgw, neglecting the fact that radiation cooling may be present. We distinguish the two fluxes and use qw to designate the wall heat flux.

A dimensionless form of the heat flux qgw is the Stanton number:

St = (3.3)

Q0

which simplifies for high flight speeds to

St =

PovSo

Other forms of the Stanton number are used, for instance

with hr and hw being the enthalpies related to the recovery temperature (r) and the actual wall (w) temperature.

For the following discussion first a wall with finite thickness and finite heat capacity is assumed, which is completely insulated from the surroundings, except at the surface, where it is exposed to the (viscous) flow. Without radiation cooling, the wall material will be heated up by the flow, depending on the heat amount penetrating the surface and the heat capacity of the material. The wall temperature will always be that of the gas at the wall: Tw = Tgw, apart from a possible temperature jump, which can be present in the slip-flow regime.

If enough heat has entered the wall material (function of time), the tem­perature in the entire wall and at the surface will reach an upper limit, the recovery temperature Tw = Tr (the heat flux goes to zero). The surface is then called an adiabatic surface: no exchange of heat takes place between gas and wall material. With steady flow conditions the recovery (adiabatic) temperature Tr is somewhat smaller than the total temperature Tt, but al­ways of the same order of magnitude. It serves as a conservative temperature estimate for the consideration of thermal loads and also of thermal-surface effects.

It was mentioned above that the total enthalpy at hypersonic flight is proportional to the flight velocity squared. This holds also for the total tem­perature, if perfect gas behavior (Chapter 5) can be assumed, which is per­mitted for vж ^ 1 km/s. The total temperature Tt then is only a function of the total enthalpy ht, eq. (3.2), which can be expressed as function of the flight Mach number Мж = vx/ax, аж being the speed of sound in the undisturbed atmosphere:

The recovery temperature Tr can be estimated with the flat-plate relation

[1]

Тг = Т^(і + г^-±М^, (3.7)

with the recovery factor r, and, like in eq. (3.6), the ratio of specific heats 7, and the free-stream Mach number M, x. For laminar boundary layers we have r = /Pr, Pr being the Prandtl number, Sub-Section 4.3.2. For turbulent boundary layers r = /Pr can be taken [1].

Eq. (3.7), like eq. (3.6), can also be formulated in terms of local parameters from the edge ‘e’ of the boundary layer at a body:

% =Te ^l + r^Me2J. (3.8)

Eqs. (3.7) and (3.8) suggest a constant recovery temperature on the surface of a configuration. Actually in general the recovery temperature is not constant and these equations can serve only to establish its order of magnitude.

At flight velocities larger than approximately 1 km/s they lose their va­lidity, because high-temperature real-gas effects appear, Chapter 5. The tem­perature in thermal and chemical equilibrium becomes a function of two variables, for instance the enthalpy and the density. At velocities larger than approximately 5 km/s, non-equilibrium effects can play a role, complicat­ing even more these relations. Since the above relations are of approximate character, actual local recovery temperatures will have to be obtained by numerical solutions of the governing equations of the respective flow fields.

If surface-radiation cooling is employed, the situation changes completely in so far, as a—usually large—fraction of the heat flux (qgw) coming to the surface is radiated away from it (qrad). For the case considered above, but with radiation cooling, the “radiation-adiabatic temperature” Tra will result: no heat is exchanged between gas and material, but the surface radiates heat away.[15]

With steady flow conditions and a steady (small) wall heat flux qw, Tra also is a conservative estimate of the surface temperature. Depending on the employed structure and materials concept (either a cold primary structure with a thermal protection system (TPS), or a hot primary structure), and on the flight trajectory segment, the actual wall temperature during flight may be somewhat lower, but in any case will be near to the radiation-adiabatic temperature, Sub-Section 3.2.3. This does not necessarily hold at structure elements with small surface radii (fuselage nose, leading edges, inlet cowl lip) and other locations, if surface-tangential heat flux is present [2, 3].

Interesting is the low-speed case after high-speed flight, where Tw in gen­eral will be larger than the momentary recovery temperature Tr (thermal reversal), due to the thermal inertia of the TPS or the hot structure. In [4] it is reported that the lift to drag ratio of the Space Shuttle Orbiter in the supersonic and the subsonic regime was underestimated during the design by up to 10 per cent. This may be at least partly attributable to the thermal reversal, which is supported by numerical investigations of J. M. Longo and R. Radespiel [5]. Turbulent skin-friction drag on the one hand depends strongly on the wall temperature. The higher the wall temperature, the lower is the turbulent skin friction, Sub-Section 7.2.3. On the other hand, the thickening of the boundary layer due to the presence of the hot surface may reduce the tile-gap induced drag, which is a surface roughness effect, Sub-Section 8.3.1.

Besides radiation cooling, which is a passive cooling means, active cooling, for instance of internal surfaces, but of course also of external ones, can be employed. In such cases a prescribed wall temperature is to be supported by a finite heat flux into the wall and towards the heat exchanger. Other cooling means are possible, see, e. g., [6].

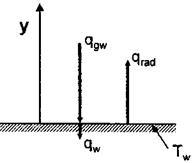
In the following we summarize the above discussion. We neglect possible shock-layer radiation and tangential heat fluxes [2], a possible temperature jump,[16] and assume, as above, that the radiative transport of heat is directed away from the surface. We then arrive at the situation shown in Fig. 3.1. Five cases can be distinguished:

1. Radiation-adiabatic wall: qw = 0, qrad = – qgw. The wall temperature is the radiation-adiabatic temperature: Tw = Tra, which is a consequence of the flux balance qgw = – qrad.

Fig. 3.1. Schematic description of the thermal state of the surface in the continuum regime, hence Tgw = Tw. Tangential fluxes and non-convex radiation cooling effects are neglected, y is the surface-normal coordinate. qgw: heat flux in the gas at the wall, qw: wall heat flux, qrad: surface radiation heat flux.

2. The wall temperature Tw without radiation cooling (qrad = 0) is pre­scribed (e. g., because of a material constraint), or it is simply given, like with a (cold) wind-tunnel model surface: the wall heat flux is equal to the heat flux in the gas at the wall qw = qgw.

3. Adiabatic wall: qgw = qw =0, qrad = 0. The wall temperature is the recovery temperature: Tw = Tr.

4. The wall temperature Tw in presence of radiation cooling (qrad > 0) is prescribed (e. g., because of a material constraint): the wall-heat flux qw is the consequence of the balance of qgw and qrad at the prescribed Tw.

5. The wall heat flux qw is prescribed (e. g., in order to obtain a certain amount of heat in a heat exchanger): the wall temperature Tw is a con­sequence of the balance of all three heat fluxes.