Determination of True Airspeed

During training, a pilot soon learns that the airspeed that appears on the airspeed indicator is not the true airspeed. Instead, in order to determine the true airspeed, the pilot must also read the altimeter and outside air tem­perature. The pilot then resorts to a small hand calculator or, in some instances, adjusts the dial on the airspeed indicator accordingly to allow for the atmospheric properties. ^

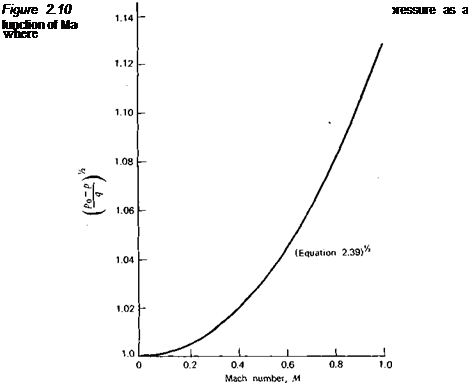
The airspeed indicator is nothing more than an accurate differential pres­sure gage calibrated according to Equation 2.31. This equation can be put in the form

The airspeed indicator measures the difference, p0-p (sometimes called the compressible dynamic pressure), but then is calibrated to obtain V by assuming standard sea level values for the acoustic velocity and the free-

/)>t f?’

stream static pressure. Thus the calibrated airspeed is defined by

where a subscript SL is used to denote standard sea level values. As a ratio to Vcai, the true airspeed can be written

_V_ { [[/(VcaQ/S + l^-‘^-ni1’2 Vca, Г L [f(VCil)+l](1’-Wl’-l JJ

^ = /(Vca,)

SL

and can be obtained from Equation 2.40. 0 and S are the temperature and pressure ratios, respectively.

If compressibility can be neglected, the airspeed will be given by Equa-

tion 2.36. In this case, using the standard sea level value of p to calibrate the airspeed indicator dial leads to the equivalent airspeed.

p(Po-p)T/2

L Psl J

where a is the density ratio.

Finally, the indicated airspeed, Viv is defined simply as the airspeed that the pilot reads from the airspeed indicator. If the indicator has no mechanical error (instrument error) and if the static source is located so that it samples the true free-stream static pressure (otherwise a position error incurs), Vf = Vcai – Furthermore, if the Mach number is net too high, V = Vcai = Ve.

As an example in determining true airspeed, suppose a pilot reads an indicated airspeed of 180 m/s for an OAT of 239 °K and an altimeter reading of 6000 m. This altitude, according to Equations 2.8 and 2.10, corresponds to a pressure ratio 8 of 0.466. The measured temperature ratio, в, is equal to 0.826. Hence <7 = 0.564. According to Equation 2.42, the true airspeed will be 239.7 m/s. From Equation 2.41, the true airspeed is calculated to be 231.6 m/s. Thus, using the incompressible relationship to estimate the true airspeed from the indicated airspeed results in a speed a few percent higher than that obtained from the calibrated airspeed relationship.

To be precise one calculates the true airspeed from the calibrated airspeed and then determines the equivalent airspeed from its definition, Equation 2.42. In the previous example, this results in a Ve of 173.9 m/s, a value 3.4% less than the calibrated airspeed.