METHOD OF SOLUTION

The continuity equation (Eq. (13.12)) is exactly the same as the corresponding steady-state equation and consequently solution methods similar to those presented in the previous (steady-state flow) chapters can be used. Recalling the formulation, based on Green’s identity (Section 3.3), the general solution to Eq. (13.12) can be constructed by integrating the contribution of the basic solutions of source a and doublet ц distributions over the body’s surface and its wakes:

Ф(х, у, z) = – і – f цп • v(-) dS – j – [ a(-) dS (13.15)

4ТГ Jbody+wake /*/ 4jT Jbody

This formulation does not include directly a vortex distribution; however it was demonstrated earlier (e. g., in Section 10.4.3) that doublet distributions can be exchanged with equivalent vortex distributions. Also, from this point and on, the velocity potential Ф is considered to be specified in terms of the body’s coordinate system.

This singular element solution automatically fulfills the boundary condi­tion of Eq. (13.5). To satisfy the boundary condition of Eq. (13.13), Eq. (13.15) is differentiated with respect to the body coordinates. The resulting velocity induced by the combination of the doublet and source distributions is then

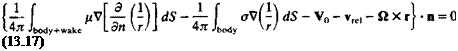In order to establish the Neumann form of the boundary value problem, the local velocity at each point on the body has to satisfy the zero-flow condition across the body’s surface (Eq. (13.13)) or, in the case of transpiration, Eq. (13.13b). By substituting Eq. (13.16) into Eq. (13.13a), the final integral equation is formed with the unknown ц and о distributions

For thick bodies, this condition of zero normal flow across solid boundaries can be defined by using the Dirichlet formulation of Section 9.2. In this case the inner perturbation potential is assumed to be constant such that

Ф; = const.

By selecting Ф, = 0 for the velocity potential (observe that the problem is formulated in the inertial X, Y, Z frame of reference where Ф* = 0, and the
magnitude of Ф corresponds to the perturbation potential[3] in the steady-state flow case) a formulation similar to that appearing in Eq. (9.11) is obtained:

Eqs. (13.17) and (13.18) still do not uniquely describe a solution, since a large number of source and doublet distributions will satisfy a set of such boundary conditions. It is possible to set the doublet strength or the source strength to zero, in a manner similar to the thick and thin airfoil cases (as in Chapter 11). A frequently followed choice for panel methods (e. g., PMARC96,97) is to set the value of the source distribution equal to the local kinematic velocity (the time-dependent equivalent of the free-stream velocity). In order to justify this, observe the Neumann boundary condition of Eq. (13.13a), which states that on the solid boundary,

дФ

— = (V0 + vrel + П X r) • n

But Eq. (3.12) states that the jump in the local normal velocity component is

дФ ЭФ,

° dn dn

and since Ф, = 0 then also ЭФJdn =0 on the solid boundary SB. Consequently, the source strength is

a= – n • (V0 + vre, + £2 X r) (13.19)