RATE OF CHANGE OF VORTICITY

To obtain an equation that governs the rate of change of vorticity of a fluid element, we start with the incompressible Navier-Stokes equations in cartesian coordinates (Eq. (1.30)),

cfq n

-^ + q* Vq = f— V —+ v V2q

at p

The convective acceleration term is rewritten using the vector identity

q2

q*Vq = V^- — qX?

Now take the curl of Eq. (1.30), with the second term on the left-hand side replaced by the right-hand side of Eq. (2.5). Note that for a scalar A, V x VA = 0 and therefore the pressure term vanishes:

^-VX(qX?) = Vxf+vV25 (2.6)

at

To simplify the result, we use the following vector identity,

Vx(qXt) = qV-&-q-V£ + S-Vq-tV-q (2 J)

along with the incompressible continuity equation and the fact that the vorticity is divergence-free (note that for any vector A, V • V X A = 0). If we also assume that the body force acting is conservative virrotational, such as gravity) then

VXf=0

and the rate of change of vorticity equation becomes

= ^r + q’V^ = ^-Vq-l-v V2£ Dt dt 4 4 *

The inviscid incompressible version of the vorticity transport equation is then

fpS’Vq (2.9)

For a flow that is two-dimensional, the vorticity is perpendicular to the flow direction and Eq. (2.8) becomes

(2.10)

and for the two-dimensional flow of an inviscid, incompressible fluid

(2.11)

and the vorticity of each fluid element is seen to remain constant.

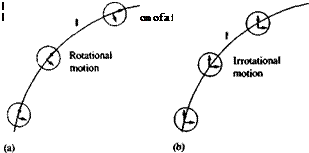
The vorticity equation (Eq. (2.8)) strongly resembles the Navier-Stokes equation and for very high values of the Reynolds number it is seen that the vorticity that is created at the solid boundary is convected along with the flow at a much faster rate than it can be diffused out across the flow and so it remains in the confines of the boundary layer and trailing wake. The fluid in the outer portion of the flowfield (the part that we will study) is seen to be effectively rotation-free (irrotational) as well as inviscid.

The above observation can be illustrated for the two-dimensional case using the nondimensional quantities defined in Eq. (1.46). Then, Eq. (2.10) can be rewritten in nondimensional form:

Dt* Re z

where the Reynolds number, Re, is defined in Eq. (1.56). Here a two – dimensional flow in the x-y plane is assumed and therefore the vorticity points in the z direction. The left-hand side in this equation is the rate at which vorticity is accumulated, which is equal to the rate at which it is being generated (near the solid boundaries of solid surfaces). It is clear from Eq. (2.10a) that for high Reynolds number flows, vorticity generation is small and can be neglected outside the boundary layer. Thus for an irrotational fluid Eq.

(2.2) reduces to

dw _dv Эи _ dw dv _ du

dy dz dz Эх Эх ду