Small Disturbance Linearization Method

As mentioned earlier, a unique solution exists for the inviscid, incompressible flow past an airfoil with sharp trailing edge. The solution satisfies the PDEs (2.1), the

tangency (2.4) and the asymptotic boundary conditions (2.6). It is of the general form

where the dependency on the geometrical parameters, coming from the tangency condition, is indicated. u and w are the exact perturbation velocity components and, in general, their analytical form cannot be obtained in closed form. However, since the solution depends continuously on the data, it is possible to expand u and w in a Taylor series with respect to the small parameters d, c and a. If we limit ourselves to the first order terms, we find

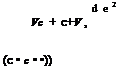
U

d e d e. .

Wd(x, z) + – we(x, z) + awa(x, z) + O +————— + a (3.16)

c c c c

where the terms O (d + ~c + a)2 represent the remainders in the Taylor expansions and are of second and higher order in the small parameters or products of them. They can be neglected if the parameters are small enough. Note that in the limit

de

a ^ 0 (3.17)

cc

the profile becomes a flat plate aligned with the incoming flow, and the uniform flow is recovered. This is the process we have used to derive the parametric representation of the Quasi-Joukowski profile geometry.

The governing equations are already linear. The next step is the linearization of the tangency condition.