Special Case, a = 0

If the disk AoA is zero (a = 0), an exact analytical solution for X can be determined. This is a physically unrealistic situation because the rotor must always be tilted slightly forward to produce a propulsive force. However, this special solution serves to illustrate the basic form of the induced part of the inflow through the rotor disk in forward flight and also provides a check case for the numerical solution (considered next). With a — 0, the induced velocity ratio in forward flight is

Squaring both sides of the above equation and rearranging gives

xf + ^x2-x4h = 0.

Dividing by XAh gives

(2.129)

which is a quadratic in (Xi/Xh)2. This quadratic has the solution

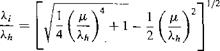(2.130)

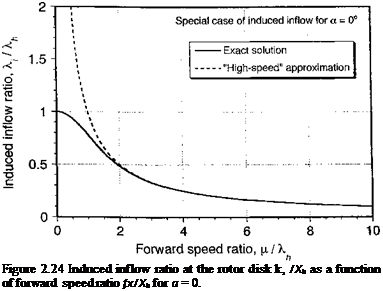This result is shown in nondimensional form in Fig. 2.24. Notice that the induced ve­locity decreases quite quickly with increasing forward flight velocity. The asymptotic

approximation is obtained by letting д A, so that in high-speed forward flight Eq. 2.127 gives

ki kfo Cj

– —– > — or that = — (Glauert’s high-speed approximation), (2.131)

A/j Д 2д

which is denoted by the broken line in Fig. 2.24. For most purposes, this approximation is satisfactory for д/А/, > 2, which in practice is the case for д > 0.1.