Dihedral/Anhedral Angles

Aircraft in a yaw/roll motion have a cross-flow over the wing affecting the aircraft roll stability (see Chapter 12). The dihedral angle (i. e., the wing tip chord raised above the wing root chord) assists roll stability. A typical dihedral angle is between 2 and 3 deg and rarely exceeds 5 deg. Figure 3.34a shows that the dihedral angle with a low-wing configuration also permits more ground clearance for the wing tip. The opposite of a dihedral angle is an anhedral angle, which lowers the wing tip with respect to the wing root and is typically associated with high-wing aircraft (Figure 3.34b). The dihedral or anhedral angle also can be applied to the horizontal tail.

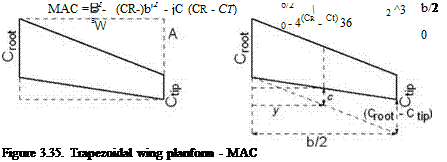
3.8 Mean Aerodynamic Chord

Various wing reference geometries and parameters are used in aerodynamic compu­tations. A most important parameter is the mean aerodynamic chord (MAC), which is the chord-weighted average chord length of the wing, defined as follows:

2 fb/2 2

MAC = c2dy, (3.37)

Sw 0

where c is the local wing chord and SW is the wing reference area:

trapezoidal wing reference area, SW (with sweep) (Figure 3.35) half wing area = rectangle – two triangles

= A x B – i/j(A – CR) x B – i/j(A – CT) x B = A x B – i/2(A x B) + i/2(B x CR) – i/2(A x B) + ^(B x CT)

= /2(Cr + CT) x B

For the full wing when the span b = 2B:

wing area, SW = /2 (CR + CT) x b Evaluating Equation 3.20 for the linear trapezoidal wing results in:

c = Croot 2(Croot Ctip)y/b

When substituting the integral, Equation 3.37 becomes:

= (2/SW)[bCr2/2 – bCR(CR – Ct)/2 – b(CR – Ct)2/6]

When substituting for the SW:

MAC = [2/(Cr + Ct)][c2 – C2 + CrCT + C2/3 + CT/3 – 2CrCT/3]

= [2/(Cr + Ct)][c2/3 + CT/3 – CrCT/3]

2 Г (Cr + Ct)2 CrCt ]

3 (Cr + Ct) (Cr + Ct)

For a linearly tapered (trapezoidal) wing, this integral is equal to:

MAC = 2/3[Croot + Ctip – CrootCtip/(Croot + Ctip)] (3.38)

For wings with a glove/yehudi, the MAC may be computed by evaluating each lin­early tapered portion and then taking an average, weighted by the area of each por­tion. In many cases, however, the MAC of the reference trapezoidal wing is used. The MAC is often used in the nondimensionalization of pitching moments as well as to compute the reference length for calculating the Re as part of the wing drag estimation. The MAC is preferred for computation over the simpler mean geomet­ric chord for aerodynamic quantities whose values are weighted more by the local chord, which are reflected by their contribution to the area.