Wing Aerodynamics

Similar to a bird’s wing, an aircraft’s wing is the lifting surface with the chosen aerofoil section, which can vary spanwise. The lift generated by the wing sustains the weight of the aircraft to make flight possible. Proper wing planform shape and size are crucial to improving aircraft efficiency and performance; however, aerofoil parameters are often compromised with the cost involved.

Downwash

Figure 3.24. Wing tip vortex

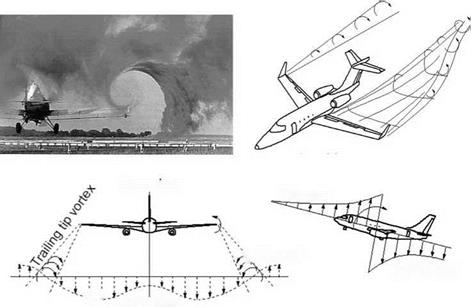
A 3D finite wing produces vortex flow as a result of tip effects, as shown in Figure 3.24 and explained in Figure 3.25. The high pressure from the lower surface rolls up at the free end of the finite wing, creating the tip vortex.

The direction of vortex flow is such that it generates downwash, which is dis­tributed spanwise at varying strengths. A reaction force of this downwash is the lift generated by the wing. Energy consumed by the downwash appears as lift- dependent induced drag, D, and its minimization is a goal of aircraft designers.

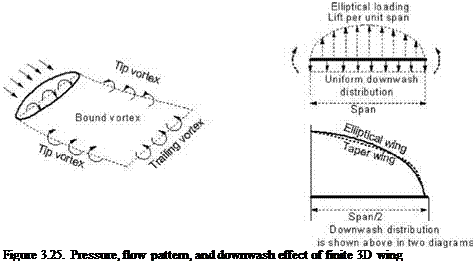The physics explained thus far is represented in geometrical definitions, as shown in Figure 3.26. This is used in formulations, as discussed herein. An elliptical wing planform (e. g., the Spitfire fighter of World War II) creates a uniform spanwise

Figure 3.26. Downwash angle and its distribution on elliptical wing planform

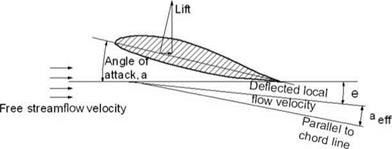
downwash at its lowest magnitude and leads to minimum induced drag. Figure 3.26 shows that the downwash effect of a 3D wing deflects free streamflow, VTO, by an angle, e, to Viocai. It can be interpreted as if the section of 3D wing behaves as a 2D infinite wing with:

effective angle of incidence, aeff = (a – e), (3.25)

where a is the angle of attack at the aerofoil section, by VTO.

Aerodynamics textbooks may be consulted to derive theoretically the down – wash angle:

e = Cl/^AR (in radians) = 57.3Cl/^AR (in deg) (3.26)

For a nonelliptical wing planform, the downwash will be higher and a semi-empirical correction factor, e, called Oswald’s efficiency factor (always < 1) is applied, as follows:

average downwash angle, e = CL/e^AR (in radians) = 57.3CL/eцAR (in deg)

(3.27)

The extent of downwash is lift-dependent; that is, it increases with an increase in Cl. Strictly speaking, Oswald’s efficiency factor, e, varies with wing incidence; however, the values used are considered an average of those found in the cruise segment and remain constant. In that case, for a particular aircraft design, the average downwash angle, e, is treated as a constant taken at the midcruise condition. Advanced wings of commercial transport aircraft can be designed in such a way that at the design point, e & 1.0.

Equations 3.26 and 3.27 show that the downwash decreases with an increase in the aspect ratio, AR. When the aspect ratio reaches infinity, there is no down – wash and the wing becomes a 2D infinite wing (i. e., no tip effects) and its sectional characteristics are represented by aerofoil characteristics. The downwash angle, e, is small – in general, less than 5 deg for aircraft with a small aspect ratio. The aerofoil section of the 3D wing apparently would produce less lift than the equivalent 2D aerofoil. Therefore, 2D aerofoil test results would require correction for a 3D wing application, as explained in the following section.

Local lift, Llocal, produced by a 3D wing, is resolved into components perpen­dicular and parallel to free streamflow, VTO. In coefficient form, the integral of these forces over the span gives the following:

CL = Lcos e/qSW and Cm = Lsin e/qSW (the induced-drag coefficient)

For small angles, e, it reduces to:

Cl = L/qSw and Cm = Le/qSw=CLe (3.28)

CDi is the drag generated from the downwash angle, e, and is lift-dependent (i. e., induced); hence, it is called the induced-drag coefficient. For a wing planform, Equa­tions 3.27 and 3.28 become:

Cm = CLe = Cl x Cl/єцAR = Cp /epAR (3.29)

Induced drag is lowest for an elliptical wing planform, when e = 1; however, it is costly to manufacture. In general, the industry uses a trapezoidal planform with a taper ratio, X & 0.4 to 0.5, resulting in an e value ranging from 0.85 to 0.98 (an optimal design approaches 1.0). A rectangular wing has a ratio of X = 1.0 and a delta wing has a ratio of X = 0, which result in an average e below 0.8. A rectangular wing with its constant chord is the least expensive planform to manufacture for having the same-sized ribs along the span.