CORRELATION PROCEDURES OF TEST DATA

From the discussion of the types and mechanism of stall­ing it is apparent that it is a separation phenomenon within the boundary layer, and thus the airfoil shape and operating Reynolds will have a large influence on the results. Because of this the test conditions, including tun­nel turbulence, also affect the results. Since we are inter­ested in the application of airfoils in free air conditions, the consideration of wind tunnel turbulence must be made before satisfactory results concerning shape and R’number can be presented. It will then be possible to correlate the results between various tunnels and will provide a reliable basis for comparisons with any newly developed theories.

Also important in the application of wind tunnel test results are the test conditions with regard to wall location if the test section is open or closed jet. Airfoils tested between walls will give two-dimensional results that must be corrected for application to a three dimensional wing. The use and correlation of such data requires that the airfoil has been tested with ends sealed as this can influ­ence the results, especially with regard to CLX • Data published before 1950 were often influenced by end leak­age problems, and caution must be exercised in their use.

(6) Maximum lift of sharp-edged sections:

a) Thin Plates and Circular Arcs, Erg TV AVA Got­tingen.

b) Doetsch. Modified Biconvex, Ybk D Lufo 1940 p. 1,54.

c) Circular-Arc Foil Sections, Bull Serv Tech Aeron (Bel­gium) 15 (1935).

d) Williams, Circular-Back Foils, ARC RM 2301 (1946).

e) See also references under (35).

f) Williams, 5% Biconvex in CAT, ARC RM 2413 (1950).

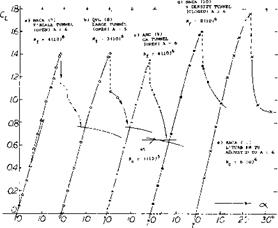
Wind Tunnel Test Conditions. Stream turbulence, found in many wind tunnels, considerably increases the Ci_x of most of the commonly used sections. This type of turbu­lence can be of practical interest, such as the case of propeller slipstream effects on a wing. Figure 7 presents the maximum lift coefficient of a given airfoil section as tested in several wind tunnels having different degrees of stream turbulence. Turbulence evidently affects the transi­tion of the boundary layer from laminar to turbulent flow so that the bubble bursting previously described is post­poned to higher lift coefficients. In this manner, turbu – [42] [43] [44] [45] [46]

Figure 7. Lift function of 23012 airfoils as tested in wind tunnels differing in Reynolds number, degree of stream turbulence and stream deflection. Comparison in different tunnels: a) FST and DVL, same type tunnels, same result, including re­duced L’curve slope to Cu = 0.4 or 0.5 (must have b’layer reason). b) Turbulent VDT does not show variation of L’curve slope; Ct_x = increased because of turbulence; but type of stall preserved. For same R’number = 8(10)6 , the Low-Turbulence Pressure Tunnel (38) indicates CL>c = 1.79, caused by effective camber. c) In the 2-dimensional Low-Turbulence Tunnel, Cuy s increased to a lesser degree by R’number (CL* is tested in that tunnel as 1.61, at 3(10)* ). Effective camber (as against A = 6) is 3.3(1.61) = 5.5%. Figure 14 suggests a corresponding maximum increase Cux = 0.15.

lence does something which is otherwise only obtained by increasing the Reynolds number. Consequently, a method for correlating wind tunnel data with free stream condi­tions has been proposed (12,a). This method consists of multiplying the actual Vc/r by a so-called turbulence factor “f”, in order to obtain the effective number R^. = “f ’ Rc. The turbulence factor suggested to be used is

“f’ = (10) /Rcrit where 4, and (3)

where Rcnf = Reynolds number of a sphere used to calibrate the particular wind tunnel, at the speed where the drag coefficient of that sphere passes through the point where either CD = 0.3, or where the pressure at the rear side of the sphere (12,b) is equal to the ambient pressure in the test section of the tunnel. The most turbulent tunnel is the NACA’s Variable Density Tunnel (13) where “f” ~ 2.7 (in its rebuilt condition, since 1929, see NACA TR 416), corresponding to root-mean square velocity fluctuations in the order of 2% of the tunnel

( 9) Airfoil sections teased by British ARC:

a) Jones, 0015 and 0030 in CAT, RM 2584 (1952).

b) Hilton, 18 Sections High Speed, RM 2058 (1942).

c) Williams, Strut Sections, RM 2457 (1951).

d) See also references (5) (28,c) (40,b), (7,c), Chapter II.

e) ARC, Investigations in the Compressed Air Tunnel, RM 1627 (R’number), 1635 (RAF-34), 1717 (RAF – 69+89), 1771 (Airscrew sections), 1772 (RAF-34), 1870 (Joukowsky), 1898 (23012), 2151 (23012 wing), 2301 (circular-arc backs), 2584 (0015 + 0030).

(10) Investigations and results on 23012 airfoils:

a) Jacobs, in FS and V’Density Tunnels, Rpt 530 (1935).

b) Jacobs, in V’Density Tunnel, NACA T Rpt 537 (1935).

c) Jacobs, Airfoil Series in VDT, NACA Rpt 610 (1937).

d) NACA, with Flaps, Rpt 534 (1935) & 664 (1939).

e) ARC, In CAT, RM 1898 (1937); also RM 2151 (1945).

0 Doetsch, In DVL Tunnel, Ybk D Lufo 1939 p. 1-88.

g) The foil section 23012 (figure 7) is outstanding in regard to maximum lift (see figure 19). The tested CLL corresponds to that of other sections having 4% camber, while 23012 has only 1.8%. The pitching moment co­efficient due to camber is but – 0.01, which is ^1/5 of that of the corresponding conventionally cambered sec­tion. Unfortunately, 23012 is not considered to be de­sirable at higher speeds.

(11) Foil sections tested in two dimensions by NACA:

a) Doenhoff, Low-Turbulence Pressure Tunnel, T Rpt 1283.

b) Abbott & von Downhoff, Collection of Data, T Rpt 824. (1945); also “Wing-Section Theory”, McGraw Hill 1949.

c) Loftin, At Very High R’Numbers, TN 1945 (1949) & Rpt 964 (1950); also RM L8L09 with results on 34 sections.

d) See also reference (37,b).

e) Loftin, 64-010 modified, TN 3244 (1954).

0 McCullough, 0006/7/8 in Ames Tunnel, TN 3524 (1955).

g) NACA, 64-xlO sections, TN 2753, 2824, 1945, 3871;

T Rpt 824, 903.

speed. Modern tunnels such as the NACA’s special low – turbulence tunnels (having fluctuations below 0.1%) would have factors only slightly above unity. Although there are strong reservations against using the Turbulence factor, the following values are for a number of the more important wind tunnels:

wind tunnel	type	stream V, dim. ft ft/sec	c, ft	Rc	“f
NACA, VDT (*)	closed 5 ft <(	75	0.4	3 (10)	2.7
Full-Scale Tunnel	open	30×60 100	6.0	4(10)	1.1
Low T Pressure TDT	closed 3×7.5	250	2.0	6(10)	1.0
AVA, 2.25 m Diameter open	7.4 ф	30	0.7	4(10)	1.2
DVL, “Large” Tunnel	open	16×23	130	2.6	3(10)	1.1
ARC-NPLCAT (*)	open	6ftjrt	80	0.7	4(10)	2.1

(*) variable-density or compressed-air tunnels with up to 10 and 25 at as operating pressure.

The airfoil chords, speeds and Reynolds numbers listed indicate typical, or possible, testing conditions in each tunnel.

Variation of “f”. Stream turbulence most certainly helps the flow pattern past typical airfoil sections to get quali­tatively above the critical phase (to be discussed later) between Rc = (1 and 2)105 . However, any quantitative agreement of CLX values thus obtained (7,a) (10,a) (12,a) only applies to a particular group of foil sections (of the sharp edge bursting-bubble type of stalling). Comparison of VDT results of sections such as the 0006 to 0015, 2412, 23012, 4412 to 21, Clark Y and RAF-34 with maximum lift coefficients obtained in low-turbulence tun­nels, extrapolated to zero turbulence, suggests “f” factors between 2.8 and 3.4 for that particular tunnel (rather than 2.7 as recommended). The factor does net “work”, on the other hand, for cambered and thicker sections (with typical trailing-edge type stalling) such as Go 387, 23015, 2415 to 21. In other words, the factor is not uniform. In its form as per equation (3) it only represents the degree of turbulence. A really reliable correction fac­tor should also take into account the specific influence of [47] the turbulence on the individual section shape. Since the critical Reynolds number of the sphere reduces as the tunnel speed is reduced (see Chapter X of “Fluid-Dynamic Drag”), it must also be suspected that the turbulence factor (in constant-pressure tunnels) varies approximately as

“f’~ 1/Vr (4)

where n ^ 4 in the carefully designed tunnels, n ^ 3 in average-quality test streams, and n ^ 2 in very turbulent conditions (14). This influence of speed can be consider­able when investigating one and the same airfoil section at different Reynolds numbers, obtained by varying the tun­nel speed.

Low-Turbulence Tunnels. It is reported in (12,e) that the turbulent fluctuations in the TDT facility increase with the operating speed from 0.02 to 0.15%. This variation is opposite to that indicated by equation (4). It seems that the source of turbulence in this elaborately designed tun­nel is acoustic (from the fan; power and blade frequency of which increase with speed). It is also mentioned in (12,e) that turbulence reduces as the operating pressure is increased. In terms of the Reynolds number, this trend is again opposite to that in other tunnels where the Rey­nolds number is varied by means of tunnel speed. In conclusion, tunnels built to a low turbulence level do not seem to follow equation (4). After all this introduction, it certainly must be clear that a realistic presentation of CLX values as a function of effective Reynolds number is problematic. We have applied turbulence factors similar to those listed above to the graphs of this text wherever this seemed to improve correlation of results from different sources. Regarding the variation of “f ’ with speed (equa­tion 4), factors different from (that is, larger than) the “standard” values have only been used wherever this seemed to eliminate obvious discrepancies. All this is somewhat arbitrary. It is believed, however, that the CLx functions thus obtained are more realistic than those presented to date in any other place.

Tunnel Corrections. The dimensions of the wind stream provided in wind tunnels are limited by reasons of econo­my (size and power of the installations). Corrections for the limited diameter of an open jet or the restraining walls in closed-type test sections have routinely been applied before presenting the lift and drag results. Since this is not a text on testing techniques, we will not go into the details of such corrections. There is one aspect, however, for which there is usually no correction applied; and that is the curvature (in contradistinction to deflection) of the stream of air passing through the tunnel. This curvature is caused by the interaction of the lift of the airfoil model

tested with the boundaries or walls of the test section. For wings of aspect ratios of 5 or 6, this effect causes a change in the effective camber ratio (discussed below) on the order of

Д (f/c)T = – 0.2% CL (5)

where the + sign is for closed, and the — sign for open tunnel test operations. For example, for Cux = 1.5, the difference is A(f/c) = – 0.3%. No direct attempt has been made, however, in the graphs presented later, to correct for this effect.

Effective Camber in Wind Tunnels. In wings of finite aspect ratio, the flow past the sections is deflected in the longitudinal direction, corresponding to the induced angle of attack. In comparison with two-dimensional condi­tions, the leading edge of a wing is, therefore, at a higher local angle of attack than the trailing edge, by the: value of ocL This effectively reduces the amount of camber of the airfoil as installed on the three dimensional wing. In terms of effective section camber, the difference may th en be

Д (f/c)% = -25 ocL = -2.5 CL /тґ A (6)

For example, at an aspect ratio A = 6, the difference is —1.3% CL, which is in the order of —2% at CLx • When testing such an airfoil in an open-throat wind tunnel flow conditions are also affected, as indicated by equation (5). On the other hand, when investigating an airfoil model in a two-dimensional closed-type wind tunnel, final down – wash is prevented. The floor of the tunnel produces an effect similar to the “ground” discussed in the Chapter III, and the ceiling of the test section doubles that effect. Conditions in the NACA’s two-dimensional tunnels (11) appears to increase effective camber of foil sections in comparison with those of airfoils in free flow. For aspect ratios between 5 and 6, the camber change is

(f/c)%~(1.4 + 0.2)CL = 1.6% C L (7)

where the second term indicates the wall effect similar to equation (5). For a value of CL* = 1.3, a difference in effective camber on the order of 2% (1) is thus found between tunnel and free flight. This conclusion is sub­stantiated by comparing maximum lift coefficients ob­tained at the same effective Reynolds number: (a) in the Low-Turbulence Pressure Tunnel (11), and (b) on finite – span wings having the same foil sections tested in the same tunnel (ll, b), or in different tunnels (7) (10). One exam­ple (15) is included in figure 7. Since airfoil sections are “always” used in three-dimensional devices (such as wings in particular), two-dimensional results obtained from test­ing airfoils between the walls in closed-type test: sections do not appear to be realistic. We have, therefore, primarily evaluated test data for airfoils on wings having finite aspect ratios of 5 or 6. However, quite a number of test points from (11), corrected (that is, increased) in effective camber to free flight conditions of an airplane wing by adding camber as per equation (7), have been included in the various graphs. All such results were evaluated for, and are classified by, their effective camber ratio.

Aspect Ratio. It is repeated at this point that all maxi­mum lift coefficients presented in the various graphs (un­less otherwise noted) are those, or are meant to represent those, of rectangular wings of aspect ratio between 5 and 6 (rather than of airfoil sections). Using equation (6), it can be estimated that the maximum lift coefficient of the wing sections represented in figure 14 varies as a function of the effective camber ratio, roughly corresponding to

d(ACLX )/d(l/A)~ —0.5 (8)

The difference between a wing with A = 5 in free flow, and a foil section in two-dimensional tunnel flow, is then Clx ~ —0.05. That is, the Clx of an airfoil with A = 5 or 6 is less than that of the foil section in a two-dimen­sional stream. By that amount on the other hand, see (15).