Problems

4.9.1

A thin profile with parabolic camber d = 0-02 is set at an angle of incidence a = 5°. Find the lift coefficient Cl in compressible flow at Mach M0 = 0.7. What is the corresponding drag coefficient Cd. If the profile is attached to a vertical axis located at x = 0 (nose of profile), write the equation of equilibrium that will give the equilibrium angle of incidence aeq at M0 = 0.7. Solve for aeq, and sketch the profile at equilibrium. Is the equilibrium stable? At which axis position along the chord would the equilibrium be neutral?

4.9.2

Consider a thin parabolic plate z = 4d| (1 – x) (zero thickness) in supersonic flow M0 > 1. Give the expressions of C+(x) and Cp (x) for this profile at a = 0. Make a plot of the pressure distributions and deduct from the graph the value of the lift coefficient. Give the formula for Cl (a). Compute the drag coefficient of the thin parabolic plate for arbitrary incidence a. Make a graph of the profile polar Cl (a) vs. Cd (a). Find on the polar the value a = a f of maximum “finesse”, i. e. corresponding to maximum f = Cl(a)/Cd(a). Calculate fmax. Does the value depend on M0? If it is considered desirable to fly the airfoil at maximum finesse at M0 = 2, give the optimum value of camber d. of the parabolic profile which should be used for a given design lift coefficient Ci, design = 0-1.

4.9.3

Consider the thin cambered plate z = 71 – § (|)2 + | (|)3^ (zero thickness),

where S is a small positive number, in a supersonic flow Mo > 1. Give the expressions of C + (x) and C – (x) for this profile at a = 0. Make a plot of the pressure distributions

and deduct from the graph the value of the lift coefficient. Calculate (Cm, o) 0. If

an axis is located at f = 2 and the thin cambered plate can rotate about it and is released at zero incidence with zero initial velocity, will it rotate with nose up, nose down or stay indifferent (neglect weight)?

4.9.4

The double wedge of Fig.4.6 of half-angle в is set at incidence a in an incoming uniform supersonic flow at Mach Mo > 1. Give the expressions of C + (x) and C~ (x) for this profile at incidence a. Make a plot of the pressure distributions. Give the expression of the lift coefficient C;(a). Compute the drag and moment coefficients, Cd (a) and Cm, o(a). Make a graph of the profile polar Ci (a) vs. Cd (a). Find on the polar the value a = a f of maximum “finesse”, i. e. corresponding to maximum f = Ci(a)/Cd(a). Sketch the waves as is done in Fig.4.6, indicating shock waves and expansion fans, when the double wedge is at the incidence of maximum finesse a f.

4.9.5

Consider a flat plate at incidence a in a uniform supersonic flow at Mach Mo > 1. Sketch the shock waves and expansion “fans”, the latter being represented by expansion shocks (zero thickness). Using the jump conditions derived in this chapter, find the two components of the perturbation velocity on the upper and lower surface of the plate that satisfy the tangency condition. From these results, find the pressure coefficients and the lift coefficient for the flat plate at incidence.

4.9.6

Describe and interpret what you see in Fig. 4.22 of a bullet cruising at Mach one. In particular discuss briefly: – the absence (out-of-frame?) of the bow shock, – the origin

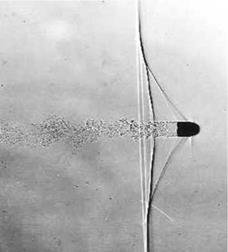Fig. 4.22 Problem 4.9.6: Shadowgraph of bullet at Mach = 1 (from https://en. wikipedia. org/wiki/File: Shockwave. jpg Author: Dpbsmith (Daniel P. B. Smith))

of the wave at the 50 % location from the nose of the bullet, – the lambda shock, – the base flow and how you would handle it in an approximate manner, within the small disturbance theory.