BASIC SOLUTION: POLYNOMIALS

Since Laplace’s equation is a second order differential equation, a linear function of position will be a solution, too:

<P = Ax + By + Cz (3.49)

The velocity components due to such a potential are

ЭФ ЗФ ЗФ

и = — = A = Ux v = — = B = V„ w = — = (3.50)

dx dy dz

where Цо, V„, and Wx are constant velocity components in the x, y, and z directions. Hence, the velocity potential due a constant free-stream flow in the x direction is

Along the same lines, additional polynomial solutions can be sought and as an example let’s consider the second-order polynomial with A, B, and C being constants:

Ф = Ax2 + By2 + Cz2 (3.53)

To satisfy the continuity equation,

У2Ф = A + B + C = 0

There are a large combination of constants that will satisfy this condition. However, one combination where one of the constants is equal to zero (e. g., В = 0) describes an interesting flow condition. Consequently

A = —C

and by substituting this result into Eq. (3.53) the velocity potential becomes

Ф = А(х2 —z2) (3.54)

The velocity components for this two-dimensional flow in the x-z plane are

и = 2Ax и = 0 w = -2Az (3.55)

To visualize this flow, the streamline equation (1.6a) is needed:

dx _dz и w

and substituting the velocity components yields

dx _ dz 2Ax 2Az

Integration by separation of variables results in

xy – const. = D (3.56)

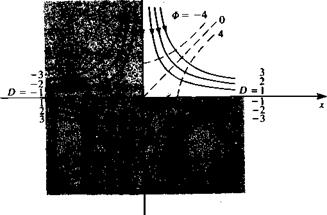
The streamlines for different constant values of D = 1, 2, 3 • • • are plotted in Fig. 3.6 and, for example, if only the first quadrant of the x-z plane is considered, then the potential describes the flow around a corner. If the upper half of the x-z plane is considered then this flow describes a stagnation flow against a wall. Note that when x = z = 0, the velocity components и = >v = 0 vanish too—which means that a stagnation point is present at the origin, and the coordinate axes x and z are also the stagnation streamline.

FIGURE 3.6 Streamlines defined by xz = constant. Note that each quadrant describes a flow in a comer.