Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Far-Field Noise Radiation
|
c0 R3 (1 – Mr) dt’ V1 – Mr dt’ V1 – Mr |
|
c0 R3(1 – Mr) dt’ І1 – Mr dt’ 1 – Mr |
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
||
 |
 |
 |
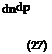In usual applications the observation point x is in the acoustic far – field, for which the general solution must be specified. This is achieved by applying commutation rules between the space derivatives and the retardedtime operator and only retaining the terms with the spherical attenuation (see Goldstein (1976) and Ffowcs Williams & Hawkings (1969)). Because the acoustic pressure is defined as p’ = c0 p’ eq. (26) is changed in
This formula shows that unsteadiness is a necessary condition for noise to be heard in the far-field. Because dipoles are fundamentally more efficient sources than quadrupoles, on the one hand, and because thickness noise is expected of secondary importance for thin surfaces on the other hand, loading noise is very often dominant and never negligible. This is why the emphasis is put on the loading-noise term to highlight the role of unsteadiness. Writing
д_ ( Pi = 1 P _ Pi д (1 – Mr)
dt’ V1 – Mr) 1 – Mr dt’ (1 – Mr)2 dt’
indicates that far-field noise has two origins, namely the intrinsic unsteadiness of the source terms, considered in the moving frame of reference attached to the surface, and the unsteadiness due to source motion expressed by the Doppler factor. Furthermore,
d(1 – Mr) = Mi (V(o) _ RjRj V(o) ____ Ri г
dt’ = R i R2 j ) Rco i ’
thus in turn the Doppler unsteadiness splits into two contributions. The second one results from the acceleration of the source; the first one also exists for non-accelerated motion but must be neglected as a near-field term in the far-field approximation. An important conclusion is that a steady force does produce sound if accelerated. This is a well-known contribution to the tonal noise radiated by high-speed rotating blades. In contrast a body in uniform translating motion produces sound in the far field only by virtue of unsteady aerodynamics, and variations of the denominator can be ignored to evaluate that noise.
 |
 |
 |
When applied to the first term of Ffowcs Williams & Hawkings’ equation the same analysis yields the simplified statement
useful for a preliminary understanding of the mixing noise from jets: far-field sound of translating quadrupoles arises from the second time derivatives of Lighthill’s stress tensor. In fact the result is just an extended version of the point-quadrupole formula derived in section 3.4.
