High-Temperature Real-Gas Effects

High-temperature real-gas effects are called those effects, which make a gas calorically imperfect as well as the effects of dissociation/recombination of molecules. We note that a molecule has four parts of internal energy [1]:

e — etrans + erot + evibr + eel• (5.4)

Here etrans is the translational energy, which also an atom has. Rotational energy erot of a molecule is fully present already at very low temperatures, and in aerothermodynamics in general is considered as fully excited.[43] Vibrational energy evibr is being excited in air at temperatures above 300 K.[44] Electronic
excitation energy eel, i. e., energy due to electronic excitation, is energy, which like ionization, usually can be neglected in the flight-speed/altitude domain considered in this book.

The high-temperature real-gas effects of interest thus are vibrational ex­citation and dissociation/recombination. Dissociated gases can be considered as mixtures of thermally perfect gases, whose molecular species are calorically imperfect.

We illustrate the parts of the internal energy by considering the degrees of freedom f, which the atoms and molecules under consideration have. We do this by means of the simple dumb-bell molecule shown in Figure 5.3.

We see that molecules (and partly also atoms) have:

— three translational degrees of freedom,

— two rotational degrees of freedom (the energy related to the rotation around the third axis a—a can be neglected),

— two vibrational degrees of freedom, i. e., one connected to the internal trans­lation movement, and one connected to the spring energy,

—

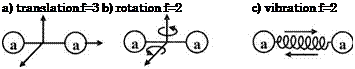the possibility of electron excitation, dissociation/recombination and ioni­zation.

d) electron excitation e) dissociation

^ (a (a –

ion electron

Fig. 5.3. Schematic of degrees of freedom f of a dumb-bell molecule, and illustra­tion of other high-temperature phenomena.

The internal energy of a mixture of thermally perfect gases in equilibrium is, with ші being the mass fraction of species i

n

e = ШіЄі. (5.5)

i=1

The internal energy of a species i is

ei etransi + eroti + evibri + eeli. (5*[45])

The terms etransi and eeli apply to both atoms and molecules, the terms eroti and evibri only to molecules.

The enthalpy of a gas is defined by [1]

h = e + ~. (5.7)

P

For thermally perfect gases we have, in general, with the specific heats at constant pressure cp and constant volume cv, and the gas constant R = cp –

cv

dh = cpdT = (cv + R) dT. (5.8)

Likewise it holds for the internal energy

de = cv dT. (5.9)

The principle of equipartition of energy [1] permits us to formulate the internal energy e, the specific heats cv, cp, and their ratio 7 = cp/cv of atoms and molecules i in terms of the degree of freedom f, which gives us insight into some basic high-temperature phenomena.

We assume excitation of all degrees of freedom (translational, rotational, vibrational) of atoms and molecules. We neglect eel, and obtain the general relations, [1], for a species with molecular weight M, R0 being the universal gas constant (R = R0/M)

— L—9.T – — L—4- — / + ^ До. _ f + 2

Є " 2 MT’ Cv ~ 2 M ’ Cp ~ 2 M ’ 7 " / ’ ^

which we now apply to the air species, Section 2.2.

Atoms (N, O). For atoms we obtain with three translational degrees of freedom (f = 3):

Molecules (N2, O2, NO)

— Molecules with translational and rotational excitation only (f = 5) have:6

6).

This case with a heat capacity twice as large as that of atoms was proposed by M. J. Lighthill in his study of the dynamics of dissociated gases [5]. It yields a good approximation for applications in a large temperature and pressure/density range[46]

ж, cpmoleCi Ж, Imoleci 1.

— Molecules with an infinitely large number of degrees of freedom (f — ж). This is a limiting case, which means

Actually this is the property of Newton flow, Sub-Section 6.7.1.