Total Power

In light of the forgoing, the total power coefficient for the helicopter in forward flight can be written in the form

Cp = Cq = —j=====.-—— ( + Kii )+- J li +XcCw + CpTR. (5.53)

The tail rotor power must always be added to obtain a proper estimate of total helicopter power requirements. For larger values of /x, then X <$C /x, so that Glauert’s formula allows Eq. 5.53 to be simplified to

Cp Ш Cq = if + 8 (1 + KhL ) + 2 ( A ) M + XcCw + Cp™’ (5’54)

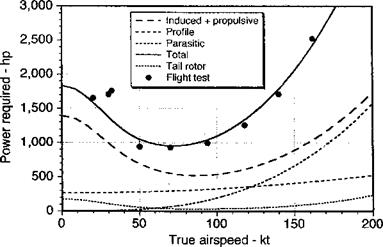
Representative results of net power required for the example helicopter in straight-and – level flight is shown in Fig. 5.10. A gross takeoff weight of 16,000 lb (7,256 kg) and an operating altitude of5,200 ft (1,585 m) has been assumed. The rotor disk AoA was calculated at each airspeed to satisfy the horizontal force equilibrium (see Fig. 2.23), which, although not a complete trim calculation, provides reasonably acceptable results. An analysis of the predicted components of the total rotor power are also shown, including that of the tail rotor. The equivalent flat-plate area, /, of the helicopter is 23.0 ft2 (2.137 m2). For both the main and tail rotors, it is assumed that к = 1.15 and Q0 = 0.008. The distance between the main and tail rotor shafts, xTR, is 32.5 ft (9.9 m).

Note from Fig. 5.10 that the induced and propulsive part of the power initially decreases with increasing airspeed but increases again as the disk is progressively tilted forward to meet greater propulsion requirements. This is because the rotor must do increasing work to overcome rotor profile and airframe parasitic losses. It is not sufficient to assume induced losses are only a result of lift generation, so induced losses decrease rapidly with airspeed to a point and then start to increase again as losses associated with propulsive forces. Unless

the problem is being solved for a free-flight trim (solving for the TPP to obtain vertical horizontal equilibrium of the helicopter) then the power curves do not represent a real flight condition. It can be seen that the power required for high-speed forward flight increases dramatically at higher airspeeds because the parasitic losses are proportional to p?. The rate of power growth is even higher when reverse flow and compressibility losses on the rotor are considered. However, the airframe drag makes a major contribution to the total power required in high-speed flight, and much can be done to expand the flight envelope by designing a more streamlined airframe. Unfortunately, because of various design constraints, this is not always an easy process. However, as discussed in Section 6.6.1, noticeable reductions in overall parasitic drag can be made by fairing-in the rotor hub to the fuselage, particularly by using streamlining downstream of the hub.