PITCHING MOMENT OF A TAIL

The forces on an isolated tail are represented just like those on an isolated wing. When the tail is mounted on an airplane, however, important inter­ferences occur. The most significant of these, and one that is usually pre­dictable by aerodynamic theory, is a downward deflection of the flow at the tail caused by the wing. This is characterized by the mean downwash angle e. Blanking of part of the tail by the body is a second effect, and a reduction of the relative wind when the tail lies in the wing wake is the third.

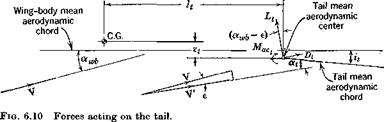
Figure 6.10 depicts the forces acting on the tail. V is the relative wind vector of the airplane, and V’ is the average or effective relative wind at the tail. The tail lift and drag forces are by definition respectively perpendicular and parallel to V’. The reader should note the tail angle it, which in keeping with Fig. 6.5 must be negative. The moment Ma c is the pitching moment of the tail about its own aerodynamic center. This is zero for a symmetrical tail section, and in any case would come mainly from the deflection of the elevator. The contribution of the tail to the airplane lift, which by definition is perpendicular to V, is

Lt cos e — Dt sin e

e is usually a small angle, and Dte may be neglected compared with Lt. The contribution of the tail to the airplane lift then becomes simply Lt. We introduce the symbol CL to represent the lift coefficient of the tail, based on the airplane dynamic pressure JtpV2 and the tail area St.

The reader should note that the lift coefficient of the tail is often based on the local dynamic pressure at the tail, which differs from JpF2 when the tail lies in the wing wake. This practice entails carrying the ratio V’JV in many subsequent equations. The definition employed here amounts to incorporating V’JV into the tail lift-curve slope at = dCLJdctt. This quantity is in any event different from that for the isolated tail, owing to the interference effects previously noted. This circumstance is handled in various ways in the literature. Sometimes a tail efficiency factor rjt is introduced, the isolated tail lift slope being multiplied by rj(. In other treatments, t]t is used to represent (V’JV)2. In the convention adopted here, at is the lift-curve slope of the tail, as measured in situ on the airplane, and based on the dynamic pressure pV2. This is the quantity that is directly obtained in a wind-tunnel test.

From Fig. 6.10 we find the pitching moment of the tail about the C. G. to be

= —lt[Lt cos (xwb — e) + Dt sin (xwb — є)]

– *([•£>( cos (xwb — e) — Lt sin (xwb – €)] + MaC( (6.3,6)

Experience has shown that in the majority of instances the dominant term in this equation is the first one, and that all others are negligible by com­parison. Only this case will be dealt with here. The reader is left to extend the analysis to situations where this approximation is not valid. With the above approximation, and that of small angles,

Mt = – ltLt = – ltCLilPV2St Upon conversion to coefficient form, we obtain

The combination ltStJ8c, is the ratio of two volumes characteristic of the airplane’s geometry. It is commonly called the “horizontal-tail volume ratio,” or more simply, the “tail volume.” It is denoted here by VH. Thus

COT( — — V H®Lt (6.3,8)

Since the center of gravity is not a fixed point, but varies with the loading condition and fuel consumption of the vehicle, VH in (6.3,8) is not a constant

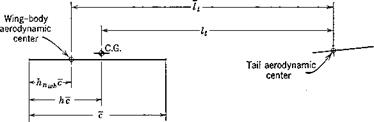
Fig. 6.11 Wing-body and tail aerodynamic centers.

(although it does not vary much). It is a little more convenient to calculate the moment of the tail about a fixed point, the mean aerodynamic center of the wing-body combination, and to use this moment in the subsequent algebraic manipulations. Figure 6.11 shows the relevant relationships, and we define

VH = ^ (6-3,9)

cS

which leads to

rH = Vs-^(h-hnJ (6.3,10)

The moment of the tail about the wing-body aerodynamic center is then [cf. (6.3,8)]

Cmt=-VHCLi (6.3,11)

and its moment about the C. G, is, from substitution of (6.3,10) into (6.3,8)

= – УвОц + CLt I (h ~ KJ (6-3,12)

PITCHING MOMENT OF A PROPULSIVE SYSTEM

The moment provided by a propulsive system is in two parts: (1) that coming from the forces acting on the unit itself, e. g. the thrust and in-plane force acting on a propeller, and (2) that coming from the interaction of the propulsive slipstream with the other parts of the airplane. These are dis­cussed in more detail in Sec. 7.3. We assume that the interference part is included in the moments already given for the wing, body, and tail, and denote by the remaining moment from the propulsion units.