Unsteady Aerodynamic Force and Work

In order to evaluate the frequency components of unsteady blade loadings, we use ’complex’ work coefficients defined by

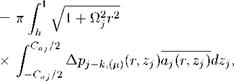j-k,(p)

(11)

where an overlined symbol denotes the complex conjugate.

Then the aerodynamic work per cycle on the vibrating blades of rotor 1 or rotor 2 is given by the imaginary part of CW1-1.(0) or CW2-2,(0) respectively. Therefore If 9[CW1_1(0)| > 0 or 3jCW2-2)(0)j > 0, the vibration is aerody­namically excited.

1.2 Coupled Bending-Torsion Flutter Analysis

The mathematical formulations of the coupled bending-torsion fhtter anal­ysis for the contra-rotating annular cascades are essentially same as conven­tional formulations for a single airfoil model except the aerodynamic force terms which include not only blade-to-blade aerodynamic coupling effects but also the effects of aerodynamic interaction between blade rows. The final form of the flutter equation can be written as

[K – A2(M + S) – A]q = 0.

Here M, S, K and A are generalized mass, static mass moment, stiffness and aerodynamic force matrices respectively, and q is a column matrix of the modal displacement amplitudes. Further A is the reduced frequency defined by

Note that the generalized aerodynamic force matrix A is complex.

In the present study equation (12) is further rearranged into the form

[C – XI] q = 0. (14)

Here C is a complex matrix and I is a unit matrix. Further

,2

x = – f, (15)

and u>b і denotes the natural circular frequency for the first order bending mode. Then X and q can be determined as eigenvalues and eigenvectors of the matrix C.

Let all elastic and aerodynamic parameters other than A be specified. Then the eigenvalues X(m) (A) : (m = 1,2,…) are functions of A. Put

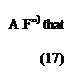
—– L^ = /W(A)+i9W(A).

m) (A)

Then the m-th flitter mode is critical at the reduced frequency A satisfies

g(m) (AFm)) = 0.

The m-th mode flutter velocity and flutter frequency are given by

Note that to search the condition (17) we should compute the aerodynamic force terms for various values of A. To this end computation by CFD may be too time-consuming. On the other hand the present analytical method is a very useful aerodynamic tool, which can provide numerical values of aerodynamic force terms for a specified reduced frequency within a few seconds on a modern personal computor.