Generalized Equations of Motion

Once the free-vibration modes have been determined for a linear, conservative system, it is a straightforward procedure to determine the system’s response to any external loading. This is accomplished by treating each mode of vibration as a dimensional degree of freedom whose scalar coordinate is the mode’s generalized coordinate. For each of these modal degrees of freedom, a “generalized equation of motion” can be formulated from Lagrange’s equations (see the Appendix and Section 2.1.5). The generalized equations of motion for the string problem can be formulated by substituting expressions for the potential and kinetic energies into

Lagrange’s equations; see Eq. (2.14), repeated here for convenience as

d dK d P

dt dji + Wi =

In the energy expressions, the string displacement is represented in terms generalized coordinates and mode shapes as

v(x, t) = Фі (x)Hi (t)

і =1

For the string, the mode shapes and their first derivatives are sinusoidal functions; consequently, they form an orthogonal set.2 That is

f Ф’і (x)<£j(x)dx = 0 (i = j) 0

It is not true in general that the derivatives of mode-shape functions form an orthogonal set.

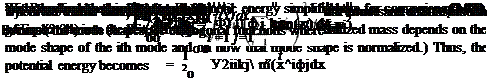
The integral in this expression can be integrated by parts as

the total kinetic energy becomes

O

K = – J2 Mi 12 (3.89)

І =1

The “generalized equations of motion” now can be obtained by substitution of the kinetic energy of Eq. (3.89) and the potential energy of Eq. (3.84) into Lagrange’s

equations given as Eqs. (3.74). The resulting equations are then

Mi (li + vfe) = E (i = 1, 2,…)

When using a modal representation, we may use equations of this form for the dynamic analysis of any linearly elastic structure. The generalized mass and natural frequencies, of course, will differ depending on whether the structure is a string, a beam in torsion or bending, a plate or shell, or a complete aircraft. The left-hand side of this equation has at least these terms regardless of the system being analyzed. Kinetic and potential energies also may contain contributions from discrete elements such as added particles, rigid bodies, or springs. Finally, additional terms could arise from potential energy of conservative applied loads, such as gravity.

The right-hand side, conversely, is highly problem-dependent and is addressed next. The case of a vibrating structure without external forces is a special case, previously discussed in Section 3.1.3. When there are no external forces, Ei = 0 for all i. The resulting general solution of Eq. (3.90) is the same as that presented in Section 3.1.3, which was obtained without reference to the generalized equations of motion and yields results depending only on the initial conditions. When including an entity such as a spring in the potential energy, we are enlarging the boundary of the system to include a new element. However, when the same entity is included through its contribution to the generalized forces, it is being treated as a source of external forces, something external to the system. Despite this philosophical distinction, the end result is the same (see Problem 5). Any effect that can be included in the generalized equations of motion through potential energy can be included instead through the generalized force. It is extremely important to not count the same effect twice (e. g., including the same entity through both potential energy and generalized forces).