Slender Bodies with General Cross Sections

Consider a slender body, with a contour A at x location and contour B at x + dx, as shown in Fig. 7.16.

In the neighborhood of the body, the governing equation in terms of a small disturbance potential is the Laplace equation in the (y, z) cross plane

Fig. 7.16 Slender body with general cross section

д 2ф д2ф 0 дy2 дz2

(7.103)

The boundary condition requires the flow to be tangent to the surface

дф dn ~Z body = U — д n dx

(7.104)

where n and t are in the normal and tangential directions of the contour of the cross section, respectively. Applying Green’s theorem in two-dimensions, yields a particular solution

ф = 27/(77- фдп)ln rdl where r = У(y – n)2 + (z – Z)2. For large r, ф can be approximated by

(7.105)

1 ф = ln r

дф dt д П

(7.106)

In this approximation, д In r is neglected compared to ln r and r — л/y2 + z2. From the boundary condition

dn dS dt = U dx dx

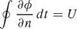
where S(x) is the cross sectional area.

The general solution is then

U

ф ~ S (x) ln r + g(x)

The pressure coefficient is

This is the same as the solution for an axisymmetric body having the same cross sectional area distribution as the actual body with a general cross section.

Oswatitsch andKeune [2], Ward [18-20] andAshley, andLandhal [1] were among the first who derived the equivalence rule for transonic, subsonic and supersonic flows: “Far away from a general slender body, the flow becomes axisymmetric and equal to the flow around the equivalent body of revolution.” “Near the slen­der body, the flow differs from that around the equivalent body of revolution by a two-dimensional constant-density cross flow part that makes the tangency condition at the body surface satisfied.” (See also Harder and Klunker [21], Guderley [22] and Cole and Cook [23]).

One can solve the axisymmetric small disturbance equation for transonic, sub­sonic or supersonic flows over the equivalent body of revolution to provide the far field boundary condition for a two-dimensional cross flow calculation at each cross section.

It can be shown that the pressure drag of the general slender body is equal to the drag of the equivalent body of revolution if the body ends in a point (S = 0) or in a cylindrical portion parallel to the free stream (S’ = 0 and ^ = 0). It is also true if the body ends with an axisymmetric portion.

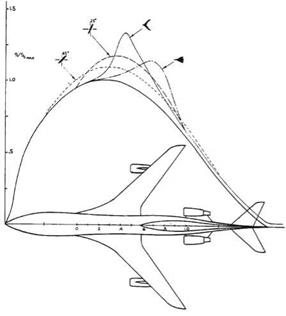
Whitcomb [24] had verified these results experimentally, and the agreement was good in the transonic regime. Therefore, it is possible to reduce the wave drag of a wing-body combination by indenting the body such that the equivalent body of revolution has a smooth area distribution. This is the basis of “transonic area rule.”

Whitcomb has also shown experimentally that at high angle of attack, the lift contributes to the cross sectional area distribution of the equivalent body.

Cheng and Hafez [25], and Cheng [26], developed a theoretical justification in the transonic regime due to the nonlinearity of the governing equation, therefore in a good design, the fuselage will have a reduced waist at the location of the wing. See Fig. 7.17 for several arrangements.

Fig. 7.17 Equivalent-body cross-section area at Mach 0.98 (from Chap. 23 of Numerical and Physical Aspects of Aerodynamic Flows, Ed. T. Cebeci, published by Springer Verlag, 1982, with kind permission of Springer)
Basic to their study are the parameters
. b _ Smax Lmax A = , t = , a = l bl pU2bl
(7.111)
where ф and ф2 are governed by two-dimensional Laplace’s equations in y and z. For larger r
Ub
(7.113)

The above expression yields an axisymmetric flow as r ^ to, confirming the area rule.

However, if t is much smaller than a, the cross flow solution breaks down before the source distribution prevails over the doublet distribution. in this case, nonlinear effects near the wing are important. The fuller equation governing the perturbation potential near the wing is

The nonlinear terms on the right hand side act as a source term in the cross flow equation (hence the doublet distribution contributes to the source distribution).

Away from the body, the three-dimensional equation must be solved to fully account for these nonlinear effects, and the solution is determined principally by the doublet and the source distributions from the solution of the cross flow equation, including the nonlinear corrections.

The doublet distribution is still proportional to b2(x), as in linear theory, however the effective source distribution consists of two parts

dSe dS d (dD2

– = + a (7.115)

dx dx dx dx

The second term in the above expression is due to the nonlinear correction in the cross flow solution, where a is a parameter representing the relative importance of the lift.

Now, one can solve the three dimensional small disturbance equation away from the body

with

and

г дф 1 _ dSe 1 sin в

= т — a D(x)

Ub д г 2п dx 2п г

the solution of the above three dimensional problem provides the far field boundary conditions for the cross flow problems at each cross section, including the nonlinear effects as a forcing function and with the tangency condition at the solid surface. Notice iterations are needed to account for the forcing function.

If lift effects are not dominant, the three dimensional problem can be split into two axisymmetric ones where

and ф0 and ф12 are governed by

Both ф0 ^ 0, and ф2 ^ 0 as г ^гх>, and as approaching the axis, we have

г дф0 1 _ dSe r2 дфі2

Ub дr 2n dx’ Ub дr

Special treatment is required to enforce the perturbed shock jump condition for ф12 problem.

For more details, the reader is referred to Cheng and Hafez [25], particularly for the estimate of the size of the region in the neighborhood of the body, which is obtained via asymptotic analysis.