Uniform Flow Plus Doublet Plus Vortex: Flow Around a Lifting Cylinder

The superposition of a uniform flow and a doublet yielded a useful body shape (i. e., a cylinder), but the flow field was symmetrical so that there was no net force on the cylinder. The addition of a vortex in the superposition creates an asymmetry in the flow. In particular, if the vortex is at the origin and has a clockwise sense, then it adds to the local velocity over the top half of the cylinder and subtracts from it over the bottom half. This asymmetry in the velocity field leads to an asymmetry in static pressure. From the Bernoulli Equation, the lower values of static pressure are on top of the cylinder and the higher values of static pressure are on the bottom of the cylinder. When integrated, this pressure asymmetry leads to a net force upward— that is, to a positive lift. Because no asymmetry is introduced about the у-axis by the superposition of the vortex at the origin, there is no unbalanced force in the x-direction. Thus, the drag of the cylinder is still zero.

Recall that in the development of Eq. 4.34 for the vortex, the constant of inte­gration in the stream-function expression arbitrarily was set equal to zero for con­venience. However, this constant may have any value because the velocity field is obtained by differentiation and the constant disappears. In this superposition to obtain the flow over a lifting cylinder, we write the constant of integration as the constant:

-—lnR

instead of setting the constant equal to zero.

The stream function for the vortex to replace Eq. 4.34 then becomes:

The constant of integration was changed so that at r = R, yv = 0. Thus, the zero streamline for the vortex now is on the circle r = R, just as it is for the superposition of a doublet with a uniform flow. Then,

The student should verify by substitution that the stream-function expression given by Eq. 4.45 is indeed a solution to the Laplace’s Equation. The streamlines resulting

from the superposition in Eq. 4.41 are seen by running Program PSI. Notice with respect to this program that:

1. There are two parameters to vary: the freestream velocity and the strength of the vortex. Note what happens when these are varied. In particular, hold the freestream velocity constant and vary the vortex strength.

2. Although the streamline у = 0 still lies on the circle r = R, it no longer lies along the x-axis away from the cylinder. In particular, from Eq. 4.45, along the stream­line у = 0:

Г lnX

sine =—– 2n R n,

Vr 1 – 4

Now, for Г > 0 (i. e., for a clockwise vortex according to the sign convention estab­lished previously), this expression states that if r is greater than R, then sin Є < 0, which places the zero streamline in the 3rd and 4th quadrants and not along the x-axis, as was the case for the nonlifting cylinder. Thus, as expected, the flow field is not symmetrical about the x-axis.

Next, we examine the velocity field:

ue = -^y = – V sin Є

e de “

Eq. 4.46 shows that the radial component of velocity is zero on the surface of the cylinder, r = R, so that the “no-flow-through” boundary condition still is satisfied. The boundary condition far from the body (i. e., for very large r) also is satisfied because far away from the body:

ur ^ V cose and ue ^ V sine so that ur[18] [19] + u2e ^ V2^

and the disturbance due to the body dies out. Finally, if we go back to the velocity – component equations for the three fundamental solutions that comprise this super­position, it is shown that the superposition represented by the new stream function in Eq. 4.45 also implies an addition of the three constituent velocity components at any point (Eq. 4.46). This fact can be useful.

Next, examine the asymmetry of the flow by locating the stagnation points on the cylinder. Again, refer to the computer solution represented by Program PSI and vary the vortex strength. Recall the condition for a stagnation point to exist— namely, that ur = ue = 0. From Eq. 4.46, ur = 0 when r = R (the cylinder surface) or when e = n/2 or 3n/2. Examine each of the following three possibilities:

Because the sine function is bounded between ± 1.0, this states that Г < 14kRV The zero value of circulation corresponds to the stagnation points on the x-axis (i. e., nonlifting case). Confirm this result by running the program.

2. If ur = 0 by virtue of 0 = n/2 and we demand that u0 = 0 there as well, then from Eq. 4.46, it follows that for a positive r, the circulation must be negative. Ignore this choice because it was shown that positive (i. e., clockwise) circulation pro­vides positive lift.

3. If ur = 0 because 0 = 3n/2, then if u0 = 0 as well, it follows from Eq. 4.46 that:

V

Solving this quadratic equation for r/R:

which is valid only if ——- > 10.

4nRVM

Now, recall from Condition (1) that if the stagnation point is on the surface of the cylinder, then Г < !4kRV! tc. Thus, the stagnation point in Condition (3) corres­ponds to a stagnation point along the negative y-axis (directly below the cylinder) and is either on or away from the surface (i. e., in the external flow field). We observe this by running the program.

Finally, we run Program PSI and take note of the pressure (and pressure – coefficient) distribution around the cylinder. The distributions are not symmetrical with respect to the x-axis and there is a resulting unbalanced (lift) force. The magni­tude of this force is determined later by integrating the pressure distribution. The pressure-coefficient information in this program is presented with positive values of the coefficient along the positive ordinate to emphasize the physical situation; how­ever, this is not the usual format for presenting airfoil pressure-coefficient information.

Does this superposition of a uniform flow, a doublet, and a vortex correspond to a physically realistic flow situation? As in the case of the nonlifting cylinder, the drag of the lifting cylinder is zero by virtue of the symmetry of the flow field about the y-axis. In this respect, the superposition is not realistic. However, it is physically possible to generate an unbalanced force on a symmetrical geometric object such as a cylinder. To understand this, the role of viscosity must be recognized. If we set up an experiment and rotate a cylinder in a clockwise direction in a uniform flow, then the large viscous-shearing action at the surface of this cylinder diffuses into the flow and tends to “pull” the flow along on the upper side and “retard” the flow on the lower side. (To visualize this, imagine rotating a cylinder in a flow of oil.) This viscous action creates a flow asymmetry that manifests as an unbalanced force on the cylinder. There is a circulation set up around the body by virtue of the viscous shear, or vorticity, at the surface; this circulation is represented by the vortex in the inviscid model. Notice that the presence of circulation does not imply that there are any circular streamlines around the cylinder.

As we would expect, the magnitude of the unbalanced force on the spinning cylinder is proportional to the rate of spin, which is an external input. There is no physical mechanism here to specify the magnitude of the spin and, hence, of the circulation. It is shown later that in the case of a sharp-edged body such as an airfoil, there is a physical mechanism present that selects a unique value of circulation for each angle of attack. Again, to explain the presence of this mechanism, it is necessary to appeal to experiment and to the role of viscosity.

Next, the force on a lifting cylinder is evaluated by integrating the surface pressure over the cylinder surface. The force in the lift (y) direction is sought. The drag (x)-direction force can be argued to be zero by symmetry or proven to be zero by integrating the net surface-pressure force in the x-direction.

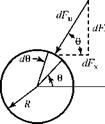
From Fig. 4.15, dFn is the normal force due to a pressure p (force per unit area) acting on an element of surface area Rd0 of the two-dimensional circular cylinder. Notice that the length of the element normal to the x-y plane is taken to be unity; the calculation then gives the force per unit length of the cylinder. Resolving this force into components in the coordinate directions:

dFx = – dFncos0 = – pRcos0d0. dFy = – dFnsin0 = – pRsin0d0.

Now, we sum these differential component forces by integration over the entire cyl­inder, recalling that V = V(0) is known so that the pressure can be expressed from the Bernoulli Equation as p = p(0). The evaluation of Fx is left to the student as an exercise. Fy is found to be:

2п 2П П/2

Fy = J dFy=- J pR sin0d0 = -2R J psin0d0,

0 0 – n/2

where the limits of integration are replaced by observing the symmetry about the y-axis.

From the Bernoulli Equation, p = po – 1/2pV2. Thus,

n/2 n/2

sm 0d0= 2Rp0 J sin0d0+pR J V2sin0d0.

-n/2 – n/2

The first integral is zero by virtue of the integration of the sine function between the limits. Now, on the surface of a cylinder with circulation:

Г

V = u0=-2V sin0——— ,

0 “ 2nR

y.

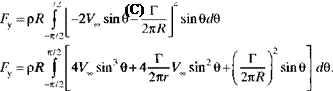because ur = 0. Thus,

The circulation and the freestream velocity are constants independent of 0. Hence, terms (A) and (C) integrate to zero between the limits, and the integral of sin20 in term (B) is n/2. Therefore:

or

(4.47)

where L’ is the lift on the cylinder (i. e., the force acting perpendicular to the freestream direction) per unit length of cylinder. The “prime” here denotes per unit length or per unit span.

Evaluating Fy in Eq. 4.47 entails using superposition to find a stream function, differentiating this function to determine velocity components, using the Bernoulli Equation to determine the pressure distribution on the cylinder, and integrating the pressure distribution to find the lift force. The final result is interesting; it shows that the lift per unit span is proportional to the circulation about the cylinder. If this were a general result, Eq. 4.47 indicates that we can evaluate the lift on a body by finding the circulation about the body; it is not necessary first to find the pressure distribution and then to integrate. This is an attractive idea because it is often easier to find the circulation about a body than it is to find the pressure distribution on the body surface and then integrate (e. g., in the thin-airfoil theory in Chapter 5). As shown in the next section, this is indeed a general result and Eq. 4.47 holds for any right cylinder (e. g., an airfoil), not only a right-circular cylinder.

Note that in Eq. 4.47, the magnitude of the circulation is arbitrary. There is nothing inherent in the flow field that determines it. Thus, the lift on the cylinder can have any value depending on the magnitude of the circulation (i. e., the magnitude of the spin that is given to the cylinder in an experiment). As discussed later, if a body in a uniform flow has a sharp edge (e. g., an airfoil with a sharp trailing edge), then the resulting flow field specifies the magnitude of the circulation about the body for any body attitude. The resulting lift force that is generated has a unique value.

EXAMPLE 4.12 Given: A lifting cylinder of radius 2 feet is experiencing a lift force of 8 pounds per foot in a freestream with a velocity of 20 ft/s. Assume steady, incompressible, inviscid flow at standard conditions.

Required: (a) What is the circulation about this cylinder? (b) Where are the stag­nation points located on the cylinder? (c) What is the maximum velocity on the surface of the cylinder? (d) What is the value of the pressure coefficient on the bottom of the cylinder?

Approach: Eqs. 4.46 and 4.47 yield the circulation strength and give velocity information. Eqs. 4.46 and 4.28 are needed for the pressure-coefficient part of the question.

Solution:

(a) From Eq. 4.47:

L’ = 8.0 = p VT = (0.002378)(20)Г ^ Г = 168ft2/s

(b)

On the cylinder surface, ur = 0 and, at the stagnation points, ue also is zero. Then, from Eq. 4.46:

Solving for Є and recognizing that the stagnation points are in the third and fourth quadrants, Є = 199.5° and Є = 340.5°.

(c) The maximum velocity is at the top of the cylinder. There, Є = n/2 and Eq. 4.46 yields a value for the tangential velocity of -53.4 ft/s; the negative sign indi­cates flow in the downstream direction.

(d) At the bottom of the cylinder, Є = 3n/2. From Eq. 4.46, ue = V = 26.6 ft/s (the positive sign now indicates the downstream direction), and from the pressure coefficient expression, Eq. 4.28:

V2

Cp = 1 – – Цг = -0.77. p V 2

Appraisal: (a) Check the units of Eq. 4.47 and confirm that circulation has the units ft2/s. Verify this by realizing that circulation is the line integral (summa­tion) of the product of a velocity and a line segment; hence, it should have the units [ft/s][ft].

(b) The locations of the stagnation points are as expected: They are symmetrical about the y axis.

(c) The velocity at the top of a nonlifting cylinder is twice the freestream value. Here, the velocity at that location is about 2.5 times the freestream value. This indicates the additive effect of the clockwise vortex and, from the Bernoulli Equation, implies that the static pressure at that point is less than it would be for the nonlifting case.

(d) The velocity at the bottom of the lifting cylinder (26.6 ft/s) is less than the value for the nonlifting cylinder (40 ft/s), indicating that at that point, the clock­wise vortex is opposing the oncoming stream. However, note that this velocity

is still greater than the oncoming freestream value (20 ft/s) so that the flow is retarded but not reversed. The same conclusion can be drawn from the negative sign on the value of the pressure coefficient at the bottom of the lifting cylinder. There is a local acceleration of the freestream flow at that point, but it is less of an acceleration than in the nonlifting case. When a stagnation point occurs on the bottom of the cylinder, the influence of the circulation is just sufficient to oppose the local flow in the downstream direction and bring it to rest.