Boundary Layer Thicknesses

Let S*(x) denote the boundary layer displacement thickness. It is defined as the distance the wall should be displaced to compensate for the deficit of mass flow rate of the boundary layer compared to the local uniform inviscid flow Ui (x), see Fig. 8.6. Mathematically this means

p(x, y)u(x, y)dy = Pi(x)ui(x) (S(x) — S*(x)) ^

(8.44)

Note: the definition of displacement thickness is valid for compressible as well as incompressible flows. S* will be used in viscous/inviscid interaction.

Let 6(x) represent the boundary layer momentum thickness. It is related to the drag due to friction as Df = pi (x)U2(x)6(x).

As will be shown later, by application of control volume (CV ) theorems, the drag due to friction can be evaluated in terms of the velocity profile exiting the CV.

rS(x) rS(x)

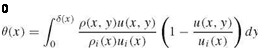— p(x, y)u2(x, y)dy + p(x, y)u(x, y)ui (x)dy = pi (x)u2(x)6(x) ^

or,

ejv = F p(x, y)u(x, у>/! – и(ЫЛ d y (8.46)

S(x) 0 Pi (x )«i (x) Ui (x) S(x)

Note: for incompressible flow: p = 1. Energy and enthalpy thicknesses can similarly be defined.

The skin friction coefficient is defined as

2 д (ul) Rei D (?)
u— Рд у
T
C	(8.47)
2 p™uiL