CONTROL FORCE GRADIENT

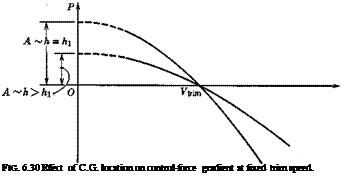It was pointed out in See. 6.7 how the trim tabs can he used to reduce the stick force to zero. A significant handling characteristic is the gradient of P with F at P = 0. The manner in which this changes as the C. G. is moved aft is illustrated in Fig. 6.30. The trim tab is assumed to be set so as to keep Ftrim the same. The gradient dP/dV is seen to decrease in magnitude as the C. G.

moves backward. When it is at the control-free neutral point, A = 0 for aircraft with or without tails, and, under the stated conditions, the P—F graph becomes a straight line lying on the F axis. This is an important characteristic of the control-free N. P.; i. e. when the C. G. is at that point, no force is required to change the trim speed.

A quantitative analysis of the control-force gradient follows.

The force is given by (6.8,9). From it we obtain the derivative

= BPV

At the speed Ftrim, P = 0, and В = —A/%pV2tIim, whence

dP _ 2 A

dV~ Ftrim

A is given following (6.8,9). Substituting the value into (6.9,1) we get

From (6.9,2) we deduce the following:

1. The control-force gradient is proportional to Sece; i. e. to the cube of airplane size.

2. It is inversely proportional to the trim speed; i. e. it increases with decreasing speed. This effect is also evident in Fig. 6.29.

3. It is directly proportional to wing loading.

4. It is independent of height for a given true speed, but decreases with height for a fixed Ге-

5. It is directly proportional to the control-free static margin.

Thus, in the absence of compressibility, the elevator control will be “heaviest” at sea-level, low-speed, forward C. G. and maximum weight.